Question Number 126039 by snipers237 last updated on 16/Dec/20
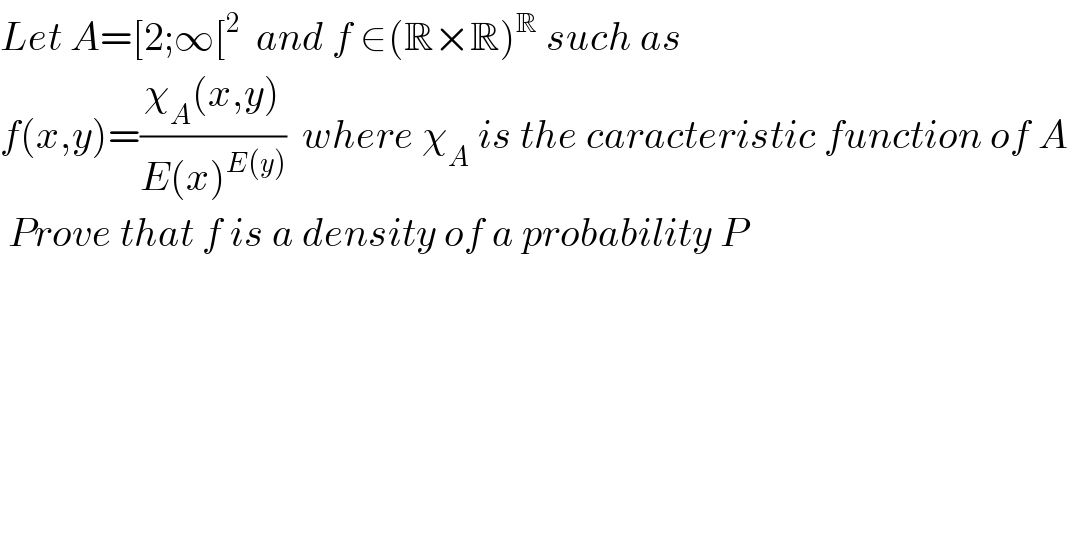
$${Let}\:{A}=\left[\mathrm{2};\infty\left[^{\mathrm{2}} \:\:{and}\:{f}\:\in\left(\mathbb{R}×\mathbb{R}\right)^{\mathbb{R}} \:{such}\:{as}\:\right.\right. \\ $$$${f}\left({x},{y}\right)=\frac{\chi_{{A}} \left({x},{y}\right)}{{E}\left({x}\right)^{{E}\left({y}\right)} }\:\:{where}\:\chi_{{A}} \:{is}\:{the}\:{caracteristic}\:{function}\:{of}\:{A} \\ $$$$\:{Prove}\:{that}\:{f}\:{is}\:{a}\:{density}\:{of}\:{a}\:{probability}\:{P} \\ $$
Answered by mindispower last updated on 18/Dec/20
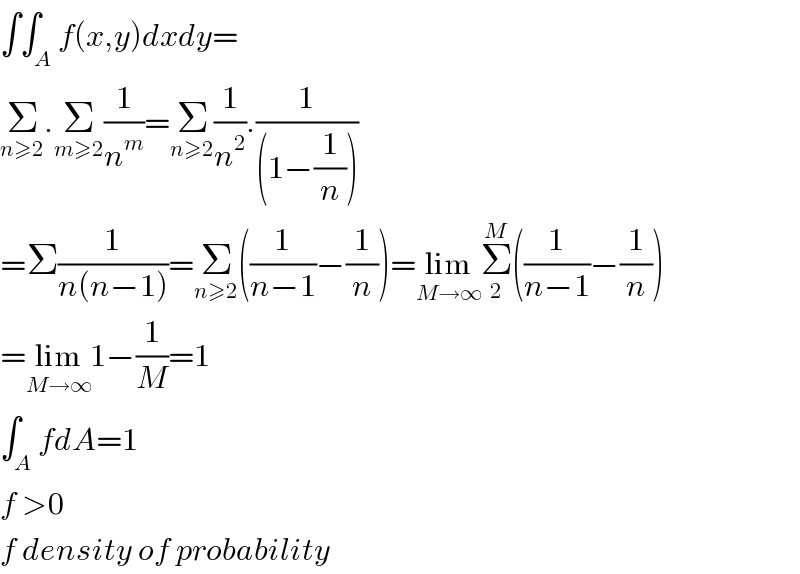
$$\int\int_{{A}} {f}\left({x},{y}\right){dxdy}= \\ $$$$\underset{{n}\geqslant\mathrm{2}} {\sum}.\underset{{m}\geqslant\mathrm{2}} {\sum}\frac{\mathrm{1}}{{n}^{{m}} }=\underset{{n}\geqslant\mathrm{2}} {\sum}\frac{\mathrm{1}}{{n}^{\mathrm{2}} }.\frac{\mathrm{1}}{\left(\mathrm{1}−\frac{\mathrm{1}}{{n}}\right)} \\ $$$$=\Sigma\frac{\mathrm{1}}{{n}\left({n}−\mathrm{1}\right)}=\underset{{n}\geqslant\mathrm{2}} {\sum}\left(\frac{\mathrm{1}}{{n}−\mathrm{1}}−\frac{\mathrm{1}}{{n}}\right)=\underset{{M}\rightarrow\infty} {\mathrm{lim}}\underset{\mathrm{2}} {\overset{{M}} {\sum}}\left(\frac{\mathrm{1}}{{n}−\mathrm{1}}−\frac{\mathrm{1}}{{n}}\right) \\ $$$$=\underset{{M}\rightarrow\infty} {\mathrm{lim}1}−\frac{\mathrm{1}}{{M}}=\mathrm{1} \\ $$$$\int_{{A}} {fdA}=\mathrm{1} \\ $$$${f}\:>\mathrm{0}\: \\ $$$${f}\:{density}\:{of}\:{probability} \\ $$
