Menu Close

x-0-pi-2-sinx-cosx-tg3x-

Tinku Tara 0 Comments
Pin




Question Number 60587 by behi83417@gmail.com last updated on 22/May/19
x∈[0,(π/2)] sinx+cosx=tg3x
$$\boldsymbol{\mathrm{x}}\in\left[\mathrm{0},\frac{\pi}{\mathrm{2}}\right] \\ $$$$\boldsymbol{\mathrm{sinx}}+\boldsymbol{\mathrm{cosx}}=\boldsymbol{\mathrm{tg}}\mathrm{3}\boldsymbol{\mathrm{x}} \\ $$
Commented by MJS last updated on 22/May/19
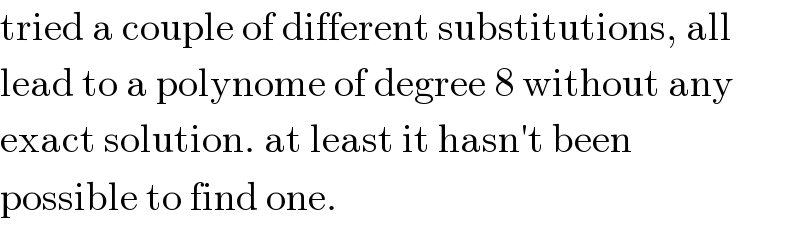
tried a couple of different substitutions, all lead to a polynome of degree 8 without any exact solution. at least it hasn′t been possible to find one.
$$\mathrm{tried}\:\mathrm{a}\:\mathrm{couple}\:\mathrm{of}\:\mathrm{different}\:\mathrm{substitutions},\:\mathrm{all} \\ $$$$\mathrm{lead}\:\mathrm{to}\:\mathrm{a}\:\mathrm{polynome}\:\mathrm{of}\:\mathrm{degree}\:\mathrm{8}\:\mathrm{without}\:\mathrm{any} \\ $$$$\mathrm{exact}\:\mathrm{solution}.\:\mathrm{at}\:\mathrm{least}\:\mathrm{it}\:\mathrm{hasn}'\mathrm{t}\:\mathrm{been} \\ $$$$\mathrm{possible}\:\mathrm{to}\:\mathrm{find}\:\mathrm{one}. \\ $$
Answered by ajfour last updated on 22/May/19
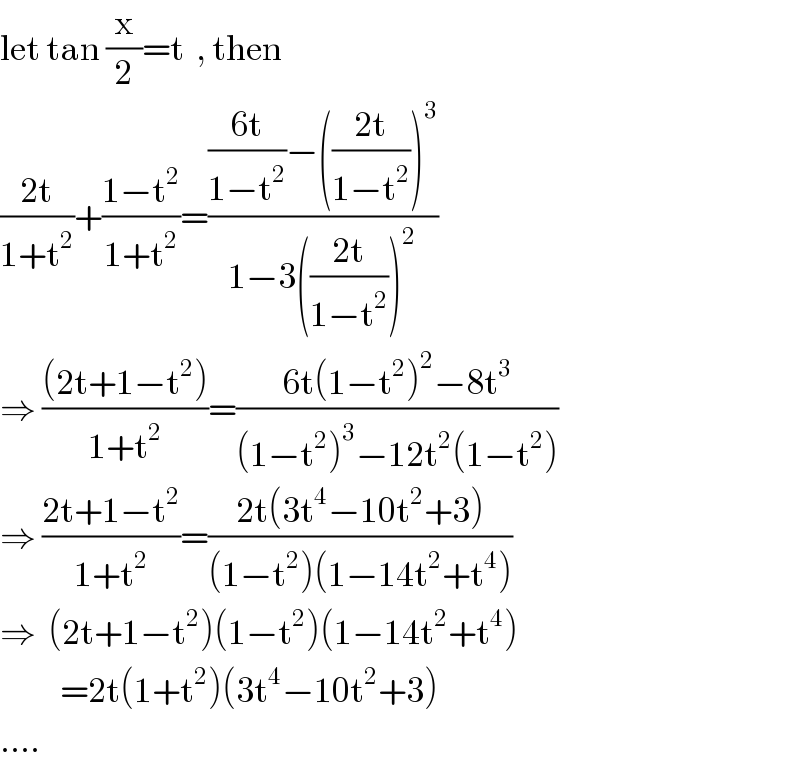
let tan (x/2)=t , then ((2t)/(1+t^2 ))+((1−t^2 )/(1+t^2 ))=((((6t)/(1−t^2 ))−(((2t)/(1−t^2 )))^3 )/(1−3(((2t)/(1−t^2 )))^2 )) ⇒ (((2t+1−t^2 ))/(1+t^2 ))=((6t(1−t^2 )^2 −8t^3 )/((1−t^2 )^3 −12t^2 (1−t^2 ))) ⇒ ((2t+1−t^2 )/(1+t^2 ))=((2t(3t^4 −10t^2 +3))/((1−t^2 )(1−14t^2 +t^4 ))) ⇒ (2t+1−t^2 )(1−t^2 )(1−14t^2 +t^4 ) =2t(1+t^2 )(3t^4 −10t^2 +3) ....
$$\mathrm{let}\:\mathrm{tan}\:\frac{\mathrm{x}}{\mathrm{2}}=\mathrm{t}\:\:,\:\mathrm{then} \\ $$$$\frac{\mathrm{2t}}{\mathrm{1}+\mathrm{t}^{\mathrm{2}} }+\frac{\mathrm{1}−\mathrm{t}^{\mathrm{2}} }{\mathrm{1}+\mathrm{t}^{\mathrm{2}} }=\frac{\frac{\mathrm{6t}}{\mathrm{1}−\mathrm{t}^{\mathrm{2}} }−\left(\frac{\mathrm{2t}}{\mathrm{1}−\mathrm{t}^{\mathrm{2}} }\right)^{\mathrm{3}} }{\mathrm{1}−\mathrm{3}\left(\frac{\mathrm{2t}}{\mathrm{1}−\mathrm{t}^{\mathrm{2}} }\right)^{\mathrm{2}} } \\ $$$$\Rightarrow\:\frac{\left(\mathrm{2t}+\mathrm{1}−\mathrm{t}^{\mathrm{2}} \right)}{\mathrm{1}+\mathrm{t}^{\mathrm{2}} }=\frac{\mathrm{6t}\left(\mathrm{1}−\mathrm{t}^{\mathrm{2}} \right)^{\mathrm{2}} −\mathrm{8t}^{\mathrm{3}} }{\left(\mathrm{1}−\mathrm{t}^{\mathrm{2}} \right)^{\mathrm{3}} −\mathrm{12t}^{\mathrm{2}} \left(\mathrm{1}−\mathrm{t}^{\mathrm{2}} \right)} \\ $$$$\Rightarrow\:\frac{\mathrm{2t}+\mathrm{1}−\mathrm{t}^{\mathrm{2}} }{\mathrm{1}+\mathrm{t}^{\mathrm{2}} }=\frac{\mathrm{2t}\left(\mathrm{3t}^{\mathrm{4}} −\mathrm{10t}^{\mathrm{2}} +\mathrm{3}\right)}{\left(\mathrm{1}−\mathrm{t}^{\mathrm{2}} \right)\left(\mathrm{1}−\mathrm{14t}^{\mathrm{2}} +\mathrm{t}^{\mathrm{4}} \right)} \\ $$$$\Rightarrow\:\:\left(\mathrm{2t}+\mathrm{1}−\mathrm{t}^{\mathrm{2}} \right)\left(\mathrm{1}−\mathrm{t}^{\mathrm{2}} \right)\left(\mathrm{1}−\mathrm{14t}^{\mathrm{2}} +\mathrm{t}^{\mathrm{4}} \right) \\ $$$$\:\:\:\:\:\:\:\:\:\:=\mathrm{2t}\left(\mathrm{1}+\mathrm{t}^{\mathrm{2}} \right)\left(\mathrm{3t}^{\mathrm{4}} −\mathrm{10t}^{\mathrm{2}} +\mathrm{3}\right) \\ $$$$…. \\ $$
Answered by tanmay last updated on 22/May/19
trying... when x∈[0,(π/2)] sinx∈[0,1] when x∈[0,(π/2)] cosx ∈[0,1] sinx+cosx (√2)((1/( (√2)))sinx+(1/( (√2)))cosx) =(√2) sin((π/4)+x) so when x∈[0,(π/2)] (sinx+cosx)∈[1,1.41] (x/(3x)) ((10^o )/(30^o )) ((20^o )/(60^o )) ((30^o )/(90^o )) ((40^o )/(120^o )) ((50^o )/(150^0 )) ((60^o )/(180^o )) ((70^o )/(210^o )) ((80^o )/( 240^o )) ((90^l )/(270^o )) when x=10^o tan3x≈0.58 when x=20^o tan3x≈1.73 so when x∈[10^o ,20^o ] tan3x=(sinx+cosx) (1)so one root lie when x ∈[(π/(18)),((2π)/(18))] when 60^o ≥x>30^o 180^o ≥3x>90^o value of tan3x (−ve) since angle lie in second quadrant. but (sinx+cosx)∈[1,1.41] (2)so no root lie when x∈[((3π)/(18)),((6π)/(18))] x=70^o tan3x≈0.58 x=80^o tan3x≈1.73 so one root lie when x∈[((7π)/(18)),((8π)/(18))] so given equation has two root in x∈[0,(π/2)] pls check...
$${trying}… \\ $$$${when}\:{x}\in\left[\mathrm{0},\frac{\pi}{\mathrm{2}}\right]\:\:\:{sinx}\in\left[\mathrm{0},\mathrm{1}\right] \\ $$$${when}\:{x}\in\left[\mathrm{0},\frac{\pi}{\mathrm{2}}\right]\:\:{cosx}\:\in\left[\mathrm{0},\mathrm{1}\right] \\ $$$${sinx}+{cosx} \\ $$$$\sqrt{\mathrm{2}}\left(\frac{\mathrm{1}}{\:\sqrt{\mathrm{2}}}{sinx}+\frac{\mathrm{1}}{\:\sqrt{\mathrm{2}}}{cosx}\right) \\ $$$$=\sqrt{\mathrm{2}}\:{sin}\left(\frac{\pi}{\mathrm{4}}+{x}\right) \\ $$$${so}\:{when}\:{x}\in\left[\mathrm{0},\frac{\pi}{\mathrm{2}}\right]\:\:\left({sinx}+{cosx}\right)\in\left[\mathrm{1},\mathrm{1}.\mathrm{41}\right] \\ $$$$\frac{{x}}{\mathrm{3}{x}}\:\frac{\mathrm{10}^{{o}} }{\mathrm{30}^{{o}} }\:\frac{\mathrm{20}^{{o}} }{\mathrm{60}^{{o}} }\:\frac{\mathrm{30}^{{o}} }{\mathrm{90}^{{o}} }\:\frac{\mathrm{40}^{{o}} }{\mathrm{120}^{{o}} }\:\frac{\mathrm{50}^{{o}} }{\mathrm{150}^{\mathrm{0}} }\:\frac{\mathrm{60}^{{o}} }{\mathrm{180}^{{o}} }\:\frac{\mathrm{70}^{{o}} }{\mathrm{210}^{{o}} }\:\frac{\mathrm{80}^{{o}} }{\:\mathrm{240}^{{o}} }\:\frac{\mathrm{90}^{{l}} }{\mathrm{270}^{{o}} } \\ $$$${when}\:{x}=\mathrm{10}^{{o}} \:\:{tan}\mathrm{3}{x}\approx\mathrm{0}.\mathrm{58} \\ $$$${when}\:{x}=\mathrm{20}^{{o}} \:{tan}\mathrm{3}{x}\approx\mathrm{1}.\mathrm{73} \\ $$$${so}\:{when}\:{x}\in\left[\mathrm{10}^{{o}} ,\mathrm{20}^{{o}} \right]\:\:\:\:{tan}\mathrm{3}{x}=\left({sinx}+{cosx}\right) \\ $$$$\left(\mathrm{1}\right){so}\:{one}\:{root}\:{lie}\:{when}\:\:{x}\:\in\left[\frac{\pi}{\mathrm{18}},\frac{\mathrm{2}\pi}{\mathrm{18}}\right] \\ $$$${when}\:\:\:\:\mathrm{60}^{{o}} \:\geqslant{x}>\mathrm{30}^{{o}} \\ $$$$\:\:\mathrm{180}^{{o}} \geqslant\mathrm{3}{x}>\mathrm{90}^{{o}} \:\:\:{value}\:{of}\:{tan}\mathrm{3}{x}\:\left(−{ve}\right) \\ $$$${since}\:{angle}\:{lie}\:{in}\:{second}\:{quadrant}. \\ $$$${but}\:\left({sinx}+{cosx}\right)\in\left[\mathrm{1},\mathrm{1}.\mathrm{41}\right] \\ $$$$\left(\mathrm{2}\right)\boldsymbol{{so}}\:\boldsymbol{{no}}\:\boldsymbol{{root}}\:{lie}\:{when}\:\boldsymbol{{x}}\in\left[\frac{\mathrm{3}\pi}{\mathrm{18}},\frac{\mathrm{6}\pi}{\mathrm{18}}\right] \\ $$$${x}=\mathrm{70}^{{o}} \:\:{tan}\mathrm{3}{x}\approx\mathrm{0}.\mathrm{58} \\ $$$${x}=\mathrm{80}^{{o}} \:\:{tan}\mathrm{3}{x}\approx\mathrm{1}.\mathrm{73} \\ $$$${so}\:{one}\:{root}\:{lie}\:{when}\:{x}\in\left[\frac{\mathrm{7}\pi}{\mathrm{18}},\frac{\mathrm{8}\pi}{\mathrm{18}}\right] \\ $$$$\boldsymbol{{so}}\:\boldsymbol{{given}}\:\boldsymbol{{equation}}\:\boldsymbol{{has}}\:\boldsymbol{{two}}\:\boldsymbol{{root}}\:\boldsymbol{{in}} \\ $$$$\boldsymbol{{x}}\in\left[\mathrm{0},\frac{\pi}{\mathrm{2}}\right] \\ $$$${pls}\:{check}… \\ $$$$ \\ $$
Commented by tanmay last updated on 22/May/19
most welcome sir
$${most}\:{welcome}\:{sir} \\ $$
Commented by behi83417@gmail.com last updated on 22/May/19
thaks in advance all my best friends. sir tanmay !i think you are right.
$${thaks}\:{in}\:{advance}\:{all}\:{my}\:\:{best}\:{friends}. \\ $$$${sir}\:{tanmay}\:!{i}\:{think}\:{you}\:{are}\:{right}. \\ $$

Pin

Leave a Reply

Your email address will not be published. Required fields are marked *