Menu Close

Question-6056

Tinku Tara 0 Comments
Pin




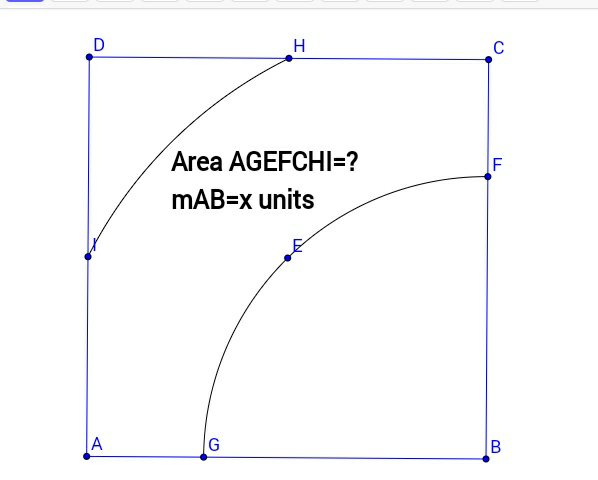
Question Number 6056 by Rasheed Soomro last updated on 11/Jun/16
Commented by Rasheed Soomro last updated on 11/Jun/16
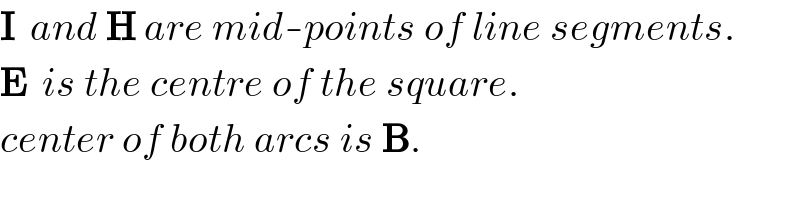
I and H are mid-points of line segments. E is the centre of the square. center of both arcs is B.
$$\boldsymbol{\mathrm{I}}\:\:{and}\:\boldsymbol{\mathrm{H}}\:{are}\:{mid}-{points}\:{of}\:{line}\:{segments}. \\ $$$$\boldsymbol{\mathrm{E}}\:\:{is}\:{the}\:{centre}\:{of}\:{the}\:{square}. \\ $$$${center}\:{of}\:{both}\:{arcs}\:{is}\:\boldsymbol{\mathrm{B}}. \\ $$
Commented by Yozzii last updated on 11/Jun/16
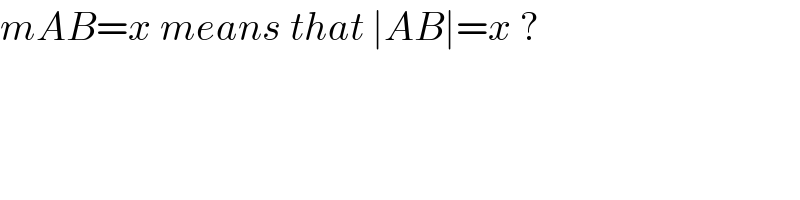
mAB=x means that ∣AB∣=x ?
$${mAB}={x}\:{means}\:{that}\:\mid{AB}\mid={x}\:? \\ $$
Commented by Rasheed Soomro last updated on 11/Jun/16
Yes.
$${Yes}. \\ $$
Answered by Yozzii last updated on 11/Jun/16
□ABCD, H lies on DC, F lies on BC, G lies on AB, I lies on AD, E lies on arc GF, E is the centre of □ABCD, HI is an arc, centre of both arcs is B, ∣HD∣=0.5∣DC∣, ∣AI∣=0.5∣AD∣, ∣AB∣=x>0, area of AGEFCHI=? −−−−−−−−−−−−−−−−−−−−−−−−−− Note that ∣AB∣=∣BC∣=∣CD∣=∣DA∣=x>0. By the Phythagorean theorem, ∣EB∣=(√((((∣AB∣)/2))^2 +(((∣BC∣)/2))^2 ))=(√((x^2 /4)×2))=x((√2)/2) ∠ABC=(π/2). Let the area of the sector FEGB be denoted by A. ∴ A=(1/2)r^2 θ A=(1/2)∣EB∣^2 (∠ABC)=(1/2)((x^2 /2))(π/2)=((πx^2 )/8) units^2 . For △HCB, its area is given by A_1 =(1/2)∣BC∣∣CH∣=(1/2)x×(1/2)x=(x^2 /4) units^2 . By symmetry of the figure, for △IAB its area is also A_1 =(x^2 /4) units^2 . Now, in △BCH, tan∠HBC=((∣HC∣)/(∣BC∣))=((x/2)/x)=(1/2) Since 0<∠HBC<(π/2)⇒∠HBC=tan^(−1) 0.5. Also, ∠IBA=tan^(−1) 0.5. Thus, ∠IBH=(π/2)−(∠IBA+∠HBC) ∠IBH=(π/2)−2tan^(−1) 0.5. Now, ∣HB∣^2 =∣IB∣^2 =∣HC∣^2 +∣BC∣^2 by the Phythagorean theorem. ∴∣HB∣^2 =(x^2 /4)+x^2 =((5x^2 )/4). Thus, the area of the sector IHB is given by A_2 =(1/2)∣HB∣^2 (∠IBH) or A_2 =(1/2)(((5x^2 )/4))((π/2)−2tan^(−1) 0.5) A_2 =((5x^2 (π−4tan^(−1) 0.5))/(16)) units^2 . The area of the region AGBFCHI is given by A_3 =A_2 +2A_1 A_3 =((5x^2 (π−4tan^(−1) 0.5))/(16))+2×(x^2 /4) A_3 =(x^2 /2)(((5π−20tan^(−1) 0.5+16)/(16))) A_3 =((x^2 (5π+16−20tan^(−1) 0.5))/(32)) The final answer is given by A_3 −A=x^2 (((5π+16−20tan^(−1) 0.5)/(32))−(π/8)) A_3 −A=x^2 (((π+16−20tan^(−1) 0.5)/(32))) units^2
$$\Box{ABCD},\:{H}\:{lies}\:{on}\:{DC},\:{F}\:{lies}\:{on}\:{BC}, \\ $$$${G}\:{lies}\:{on}\:{AB},\:{I}\:{lies}\:{on}\:{AD},\:{E}\:{lies} \\ $$$${on}\:{arc}\:{GF},\:{E}\:{is}\:{the}\:{centre}\:{of}\:\Box{ABCD}, \\ $$$${HI}\:{is}\:{an}\:{arc},\:{centre}\:{of}\:{both}\:{arcs}\:{is}\:{B}, \\ $$$$\mid{HD}\mid=\mathrm{0}.\mathrm{5}\mid{DC}\mid,\:\mid{AI}\mid=\mathrm{0}.\mathrm{5}\mid{AD}\mid, \\ $$$$\mid{AB}\mid={x}>\mathrm{0},\:{area}\:{of}\:{AGEFCHI}=? \\ $$$$−−−−−−−−−−−−−−−−−−−−−−−−−− \\ $$$${Note}\:{that}\:\mid{AB}\mid=\mid{BC}\mid=\mid{CD}\mid=\mid{DA}\mid={x}>\mathrm{0}. \\ $$$${By}\:{the}\:{Phythagorean}\:{theorem},\: \\ $$$$\mid{EB}\mid=\sqrt{\left(\frac{\mid{AB}\mid}{\mathrm{2}}\right)^{\mathrm{2}} +\left(\frac{\mid{BC}\mid}{\mathrm{2}}\right)^{\mathrm{2}} }=\sqrt{\frac{{x}^{\mathrm{2}} }{\mathrm{4}}×\mathrm{2}}={x}\frac{\sqrt{\mathrm{2}}}{\mathrm{2}} \\ $$$$\angle{ABC}=\frac{\pi}{\mathrm{2}}.\:{Let}\:{the}\:{area}\:{of}\:{the}\:{sector}\:{FEGB} \\ $$$${be}\:{denoted}\:{by}\:{A}.\:\therefore\:{A}=\frac{\mathrm{1}}{\mathrm{2}}{r}^{\mathrm{2}} \theta \\ $$$${A}=\frac{\mathrm{1}}{\mathrm{2}}\mid{EB}\mid^{\mathrm{2}} \left(\angle{ABC}\right)=\frac{\mathrm{1}}{\mathrm{2}}\left(\frac{{x}^{\mathrm{2}} }{\mathrm{2}}\right)\frac{\pi}{\mathrm{2}}=\frac{\pi{x}^{\mathrm{2}} }{\mathrm{8}}\:{units}^{\mathrm{2}} . \\ $$$$ \\ $$$${For}\:\bigtriangleup{HCB},\:{its}\:{area}\:{is}\:{given}\:{by} \\ $$$${A}_{\mathrm{1}} =\frac{\mathrm{1}}{\mathrm{2}}\mid{BC}\mid\mid{CH}\mid=\frac{\mathrm{1}}{\mathrm{2}}{x}×\frac{\mathrm{1}}{\mathrm{2}}{x}=\frac{{x}^{\mathrm{2}} }{\mathrm{4}}\:{units}^{\mathrm{2}} . \\ $$$${By}\:{symmetry}\:{of}\:{the}\:{figure},\:{for}\:\bigtriangleup{IAB} \\ $$$${its}\:{area}\:{is}\:{also}\:{A}_{\mathrm{1}} =\frac{{x}^{\mathrm{2}} }{\mathrm{4}}\:{units}^{\mathrm{2}} . \\ $$$${Now},\:{in}\:\bigtriangleup{BCH},\:{tan}\angle{HBC}=\frac{\mid{HC}\mid}{\mid{BC}\mid}=\frac{{x}/\mathrm{2}}{{x}}=\frac{\mathrm{1}}{\mathrm{2}} \\ $$$${Since}\:\mathrm{0}<\angle{HBC}<\frac{\pi}{\mathrm{2}}\Rightarrow\angle{HBC}={tan}^{−\mathrm{1}} \mathrm{0}.\mathrm{5}. \\ $$$${Also},\:\angle{IBA}={tan}^{−\mathrm{1}} \mathrm{0}.\mathrm{5}. \\ $$$${Thus},\:\angle{IBH}=\frac{\pi}{\mathrm{2}}−\left(\angle{IBA}+\angle{HBC}\right) \\ $$$$\angle{IBH}=\frac{\pi}{\mathrm{2}}−\mathrm{2}{tan}^{−\mathrm{1}} \mathrm{0}.\mathrm{5}. \\ $$$${Now},\:\mid{HB}\mid^{\mathrm{2}} =\mid{IB}\mid^{\mathrm{2}} =\mid{HC}\mid^{\mathrm{2}} +\mid{BC}\mid^{\mathrm{2}} \:{by}\: \\ $$$${the}\:{Phythagorean}\:{theorem}. \\ $$$$\therefore\mid{HB}\mid^{\mathrm{2}} =\frac{{x}^{\mathrm{2}} }{\mathrm{4}}+{x}^{\mathrm{2}} =\frac{\mathrm{5}{x}^{\mathrm{2}} }{\mathrm{4}}. \\ $$$${Thus},\:{the}\:{area}\:{of}\:{the}\:{sector}\:{IHB}\:{is} \\ $$$${given}\:{by}\:{A}_{\mathrm{2}} =\frac{\mathrm{1}}{\mathrm{2}}\mid{HB}\mid^{\mathrm{2}} \left(\angle{IBH}\right) \\ $$$${or}\:{A}_{\mathrm{2}} =\frac{\mathrm{1}}{\mathrm{2}}\left(\frac{\mathrm{5}{x}^{\mathrm{2}} }{\mathrm{4}}\right)\left(\frac{\pi}{\mathrm{2}}−\mathrm{2}{tan}^{−\mathrm{1}} \mathrm{0}.\mathrm{5}\right) \\ $$$${A}_{\mathrm{2}} =\frac{\mathrm{5}{x}^{\mathrm{2}} \left(\pi−\mathrm{4}{tan}^{−\mathrm{1}} \mathrm{0}.\mathrm{5}\right)}{\mathrm{16}}\:{units}^{\mathrm{2}} . \\ $$$${The}\:{area}\:{of}\:{the}\:{region}\:{AGBFCHI}\:{is} \\ $$$${given}\:{by}\:{A}_{\mathrm{3}} ={A}_{\mathrm{2}} +\mathrm{2}{A}_{\mathrm{1}} \\ $$$${A}_{\mathrm{3}} =\frac{\mathrm{5}{x}^{\mathrm{2}} \left(\pi−\mathrm{4}{tan}^{−\mathrm{1}} \mathrm{0}.\mathrm{5}\right)}{\mathrm{16}}+\mathrm{2}×\frac{{x}^{\mathrm{2}} }{\mathrm{4}} \\ $$$${A}_{\mathrm{3}} =\frac{{x}^{\mathrm{2}} }{\mathrm{2}}\left(\frac{\mathrm{5}\pi−\mathrm{20}{tan}^{−\mathrm{1}} \mathrm{0}.\mathrm{5}+\mathrm{16}}{\mathrm{16}}\right) \\ $$$${A}_{\mathrm{3}} =\frac{{x}^{\mathrm{2}} \left(\mathrm{5}\pi+\mathrm{16}−\mathrm{20}{tan}^{−\mathrm{1}} \mathrm{0}.\mathrm{5}\right)}{\mathrm{32}} \\ $$$${The}\:{final}\:{answer}\:{is}\:{given}\:{by}\: \\ $$$${A}_{\mathrm{3}} −{A}={x}^{\mathrm{2}} \left(\frac{\mathrm{5}\pi+\mathrm{16}−\mathrm{20}{tan}^{−\mathrm{1}} \mathrm{0}.\mathrm{5}}{\mathrm{32}}−\frac{\pi}{\mathrm{8}}\right) \\ $$$${A}_{\mathrm{3}} −{A}={x}^{\mathrm{2}} \left(\frac{\pi+\mathrm{16}−\mathrm{20}{tan}^{−\mathrm{1}} \mathrm{0}.\mathrm{5}}{\mathrm{32}}\right)\:{units}^{\mathrm{2}} \\ $$
Commented by Rasheed Soomro last updated on 11/Jun/16
G_(OO) D^(V) Strategy! A Strategy I didn′t think of!
$$\overset{\mathcal{V}} {\mathcal{G}_{\mathcal{OO}} \mathcal{D}}\:\:\mathcal{S}\mathfrak{t}{rategy}! \\ $$$$\mathcal{A}\:\mathcal{S}\mathfrak{t}{rategy}\:\mathcal{I}\:{didn}'{t}\:{think}\:{of}! \\ $$

Pin

Leave a Reply

Your email address will not be published. Required fields are marked *