Menu Close

solve-inside-N-2-x-1-y-2-2xy-

Tinku Tara 0 Comments
Pin




Question Number 61650 by maxmathsup by imad last updated on 05/Jun/19
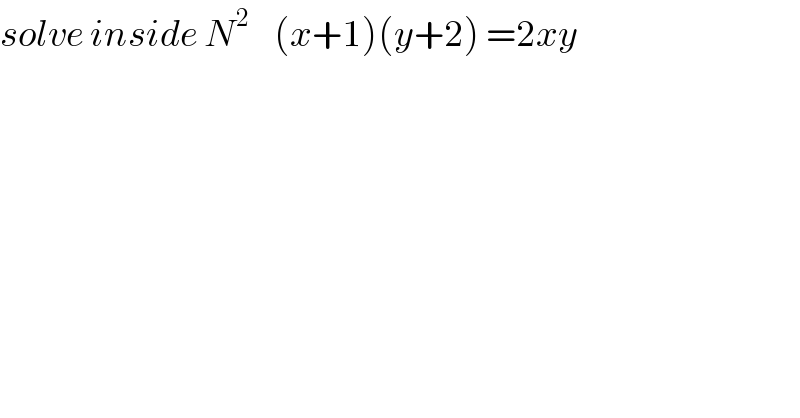
solve inside N^2 (x+1)(y+2) =2xy
$${solve}\:{inside}\:{N}^{\mathrm{2}} \:\:\:\:\left({x}+\mathrm{1}\right)\left({y}+\mathrm{2}\right)\:=\mathrm{2}{xy} \\ $$
Commented by kaivan.ahmadi last updated on 06/Jun/19
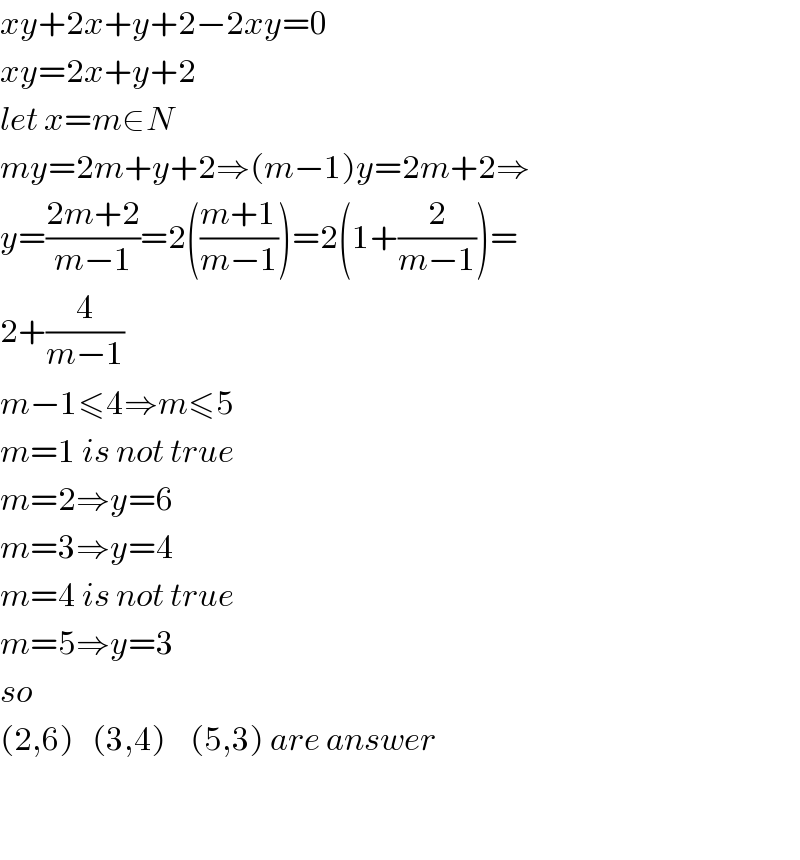
xy+2x+y+2−2xy=0 xy=2x+y+2 let x=m∈N my=2m+y+2⇒(m−1)y=2m+2⇒ y=((2m+2)/(m−1))=2(((m+1)/(m−1)))=2(1+(2/(m−1)))= 2+(4/(m−1)) m−1≤4⇒m≤5 m=1 is not true m=2⇒y=6 m=3⇒y=4 m=4 is not true m=5⇒y=3 so (2,6) (3,4) (5,3) are answer
$${xy}+\mathrm{2}{x}+{y}+\mathrm{2}−\mathrm{2}{xy}=\mathrm{0} \\ $$$${xy}=\mathrm{2}{x}+{y}+\mathrm{2} \\ $$$${let}\:{x}={m}\in{N} \\ $$$${my}=\mathrm{2}{m}+{y}+\mathrm{2}\Rightarrow\left({m}−\mathrm{1}\right){y}=\mathrm{2}{m}+\mathrm{2}\Rightarrow \\ $$$${y}=\frac{\mathrm{2}{m}+\mathrm{2}}{{m}−\mathrm{1}}=\mathrm{2}\left(\frac{{m}+\mathrm{1}}{{m}−\mathrm{1}}\right)=\mathrm{2}\left(\mathrm{1}+\frac{\mathrm{2}}{{m}−\mathrm{1}}\right)= \\ $$$$\mathrm{2}+\frac{\mathrm{4}}{{m}−\mathrm{1}} \\ $$$${m}−\mathrm{1}\leqslant\mathrm{4}\Rightarrow{m}\leqslant\mathrm{5} \\ $$$${m}=\mathrm{1}\:{is}\:{not}\:{true} \\ $$$${m}=\mathrm{2}\Rightarrow{y}=\mathrm{6} \\ $$$${m}=\mathrm{3}\Rightarrow{y}=\mathrm{4} \\ $$$${m}=\mathrm{4}\:{is}\:{not}\:{true} \\ $$$${m}=\mathrm{5}\Rightarrow{y}=\mathrm{3} \\ $$$${so}\: \\ $$$$\left(\mathrm{2},\mathrm{6}\right)\:\:\:\left(\mathrm{3},\mathrm{4}\right)\:\:\:\:\left(\mathrm{5},\mathrm{3}\right)\:{are}\:{answer} \\ $$$$ \\ $$$$ \\ $$
Commented by maxmathsup by imad last updated on 06/Jun/19
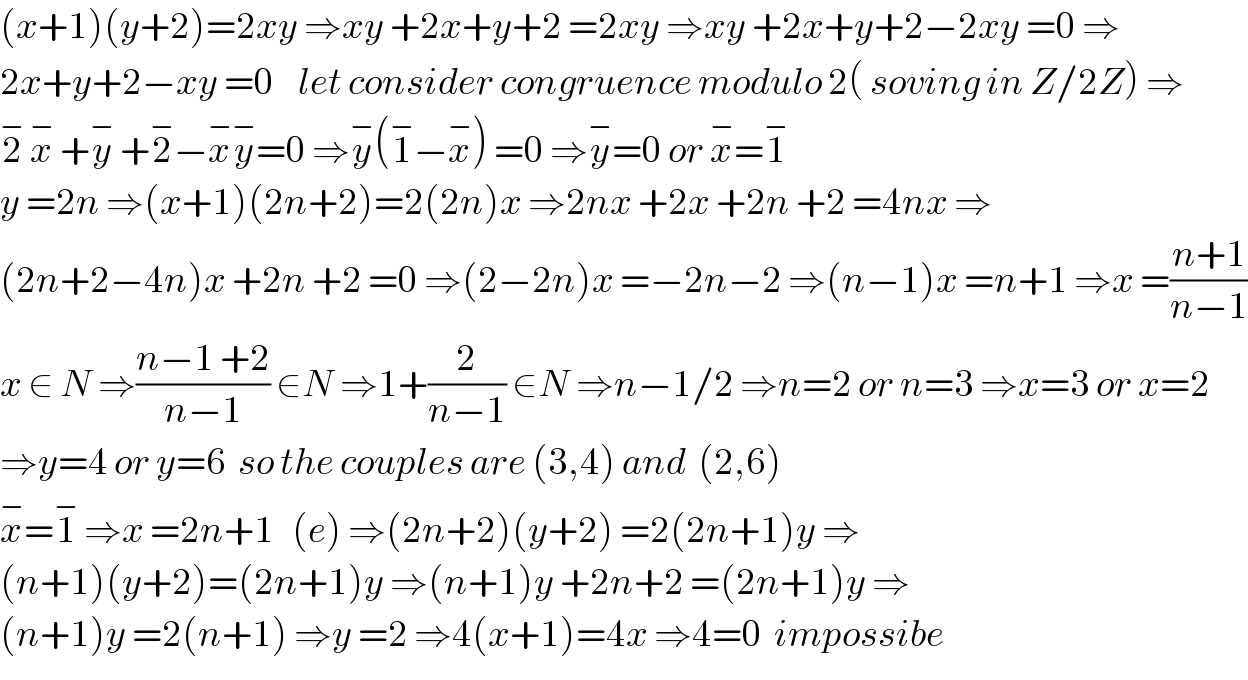
(x+1)(y+2)=2xy ⇒xy +2x+y+2 =2xy ⇒xy +2x+y+2−2xy =0 ⇒ 2x+y+2−xy =0 let consider congruence modulo 2( soving in Z/2Z) ⇒ 2^− x^− +y^− +2^− −x^− y^− =0 ⇒y^− (1^− −x^− ) =0 ⇒y^− =0 or x^− =1^− y =2n ⇒(x+1)(2n+2)=2(2n)x ⇒2nx +2x +2n +2 =4nx ⇒ (2n+2−4n)x +2n +2 =0 ⇒(2−2n)x =−2n−2 ⇒(n−1)x =n+1 ⇒x =((n+1)/(n−1)) x ∈ N ⇒((n−1 +2)/(n−1)) ∈N ⇒1+(2/(n−1)) ∈N ⇒n−1/2 ⇒n=2 or n=3 ⇒x=3 or x=2 ⇒y=4 or y=6 so the couples are (3,4) and (2,6) x^− =1^− ⇒x =2n+1 (e) ⇒(2n+2)(y+2) =2(2n+1)y ⇒ (n+1)(y+2)=(2n+1)y ⇒(n+1)y +2n+2 =(2n+1)y ⇒ (n+1)y =2(n+1) ⇒y =2 ⇒4(x+1)=4x ⇒4=0 impossibe
$$\left({x}+\mathrm{1}\right)\left({y}+\mathrm{2}\right)=\mathrm{2}{xy}\:\Rightarrow{xy}\:+\mathrm{2}{x}+{y}+\mathrm{2}\:=\mathrm{2}{xy}\:\Rightarrow{xy}\:+\mathrm{2}{x}+{y}+\mathrm{2}−\mathrm{2}{xy}\:=\mathrm{0}\:\Rightarrow \\ $$$$\mathrm{2}{x}+{y}+\mathrm{2}−{xy}\:=\mathrm{0}\:\:\:\:{let}\:{consider}\:{congruence}\:{modulo}\:\mathrm{2}\left(\:{soving}\:{in}\:{Z}/\mathrm{2}{Z}\right)\:\Rightarrow \\ $$$$\overset{−} {\mathrm{2}}\:\overset{−} {{x}}\:+\overset{−} {{y}}\:+\overset{−} {\mathrm{2}}−\overset{−} {{x}}\overset{−} {{y}}=\mathrm{0}\:\Rightarrow\overset{−} {{y}}\left(\overset{−} {\mathrm{1}}−\overset{−} {{x}}\right)\:=\mathrm{0}\:\Rightarrow\overset{−} {{y}}=\mathrm{0}\:{or}\:\overset{−} {{x}}=\overset{−} {\mathrm{1}} \\ $$$${y}\:=\mathrm{2}{n}\:\Rightarrow\left({x}+\mathrm{1}\right)\left(\mathrm{2}{n}+\mathrm{2}\right)=\mathrm{2}\left(\mathrm{2}{n}\right){x}\:\Rightarrow\mathrm{2}{nx}\:+\mathrm{2}{x}\:+\mathrm{2}{n}\:+\mathrm{2}\:=\mathrm{4}{nx}\:\Rightarrow \\ $$$$\left(\mathrm{2}{n}+\mathrm{2}−\mathrm{4}{n}\right){x}\:+\mathrm{2}{n}\:+\mathrm{2}\:=\mathrm{0}\:\Rightarrow\left(\mathrm{2}−\mathrm{2}{n}\right){x}\:=−\mathrm{2}{n}−\mathrm{2}\:\Rightarrow\left({n}−\mathrm{1}\right){x}\:={n}+\mathrm{1}\:\Rightarrow{x}\:=\frac{{n}+\mathrm{1}}{{n}−\mathrm{1}} \\ $$$${x}\:\in\:{N}\:\Rightarrow\frac{{n}−\mathrm{1}\:+\mathrm{2}}{{n}−\mathrm{1}}\:\in{N}\:\Rightarrow\mathrm{1}+\frac{\mathrm{2}}{{n}−\mathrm{1}}\:\in{N}\:\Rightarrow{n}−\mathrm{1}/\mathrm{2}\:\Rightarrow{n}=\mathrm{2}\:{or}\:{n}=\mathrm{3}\:\Rightarrow{x}=\mathrm{3}\:{or}\:{x}=\mathrm{2} \\ $$$$\Rightarrow{y}=\mathrm{4}\:{or}\:{y}=\mathrm{6}\:\:{so}\:{the}\:{couples}\:{are}\:\left(\mathrm{3},\mathrm{4}\right)\:{and}\:\:\left(\mathrm{2},\mathrm{6}\right) \\ $$$$\overset{−} {{x}}=\overset{−} {\mathrm{1}}\:\Rightarrow{x}\:=\mathrm{2}{n}+\mathrm{1}\:\:\:\left({e}\right)\:\Rightarrow\left(\mathrm{2}{n}+\mathrm{2}\right)\left({y}+\mathrm{2}\right)\:=\mathrm{2}\left(\mathrm{2}{n}+\mathrm{1}\right){y}\:\Rightarrow \\ $$$$\left({n}+\mathrm{1}\right)\left({y}+\mathrm{2}\right)=\left(\mathrm{2}{n}+\mathrm{1}\right){y}\:\Rightarrow\left({n}+\mathrm{1}\right){y}\:+\mathrm{2}{n}+\mathrm{2}\:=\left(\mathrm{2}{n}+\mathrm{1}\right){y}\:\Rightarrow \\ $$$$\left({n}+\mathrm{1}\right){y}\:=\mathrm{2}\left({n}+\mathrm{1}\right)\:\Rightarrow{y}\:=\mathrm{2}\:\Rightarrow\mathrm{4}\left({x}+\mathrm{1}\right)=\mathrm{4}{x}\:\Rightarrow\mathrm{4}=\mathrm{0}\:\:{impossibe}\: \\ $$
Commented by maxmathsup by imad last updated on 06/Jun/19
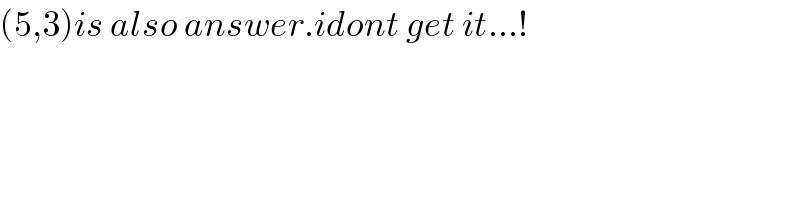
(5,3)is also answer.idont get it...!
$$\left(\mathrm{5},\mathrm{3}\right){is}\:{also}\:{answer}.{idont}\:{get}\:{it}…! \\ $$
Commented by maxmathsup by imad last updated on 06/Jun/19
thanks sir Ahmadi.
$${thanks}\:{sir}\:{Ahmadi}. \\ $$

Pin

Leave a Reply

Your email address will not be published. Required fields are marked *