Question Number 61835 by alphaprime last updated on 09/Jun/19
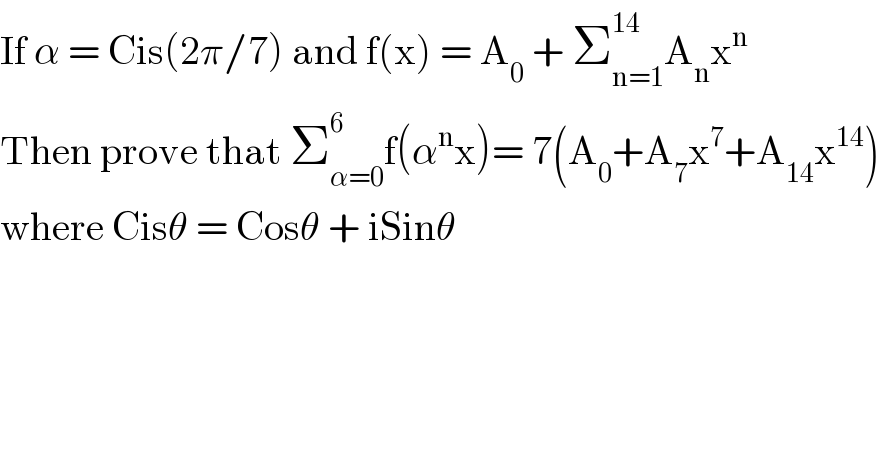
$$\mathrm{If}\:\alpha\:=\:\mathrm{Cis}\left(\mathrm{2}\pi/\mathrm{7}\right)\:\mathrm{and}\:\mathrm{f}\left(\mathrm{x}\right)\:=\:\mathrm{A}_{\mathrm{0}} \:+\:\sum_{\mathrm{n}=\mathrm{1}} ^{\mathrm{14}} \mathrm{A}_{\mathrm{n}} \mathrm{x}^{\mathrm{n}} \: \\ $$$$\mathrm{Then}\:\mathrm{prove}\:\mathrm{that}\:\sum_{\alpha=\mathrm{0}} ^{\mathrm{6}} \mathrm{f}\left(\alpha^{\mathrm{n}} \mathrm{x}\right)=\:\mathrm{7}\left(\mathrm{A}_{\mathrm{0}} +\mathrm{A}_{\mathrm{7}} \mathrm{x}^{\mathrm{7}} +\mathrm{A}_{\mathrm{14}} \mathrm{x}^{\mathrm{14}} \right) \\ $$$$\mathrm{where}\:\mathrm{Cis}\theta\:=\:\mathrm{Cos}\theta\:+\:\mathrm{iSin}\theta \\ $$
Commented by arcana last updated on 10/Jun/19
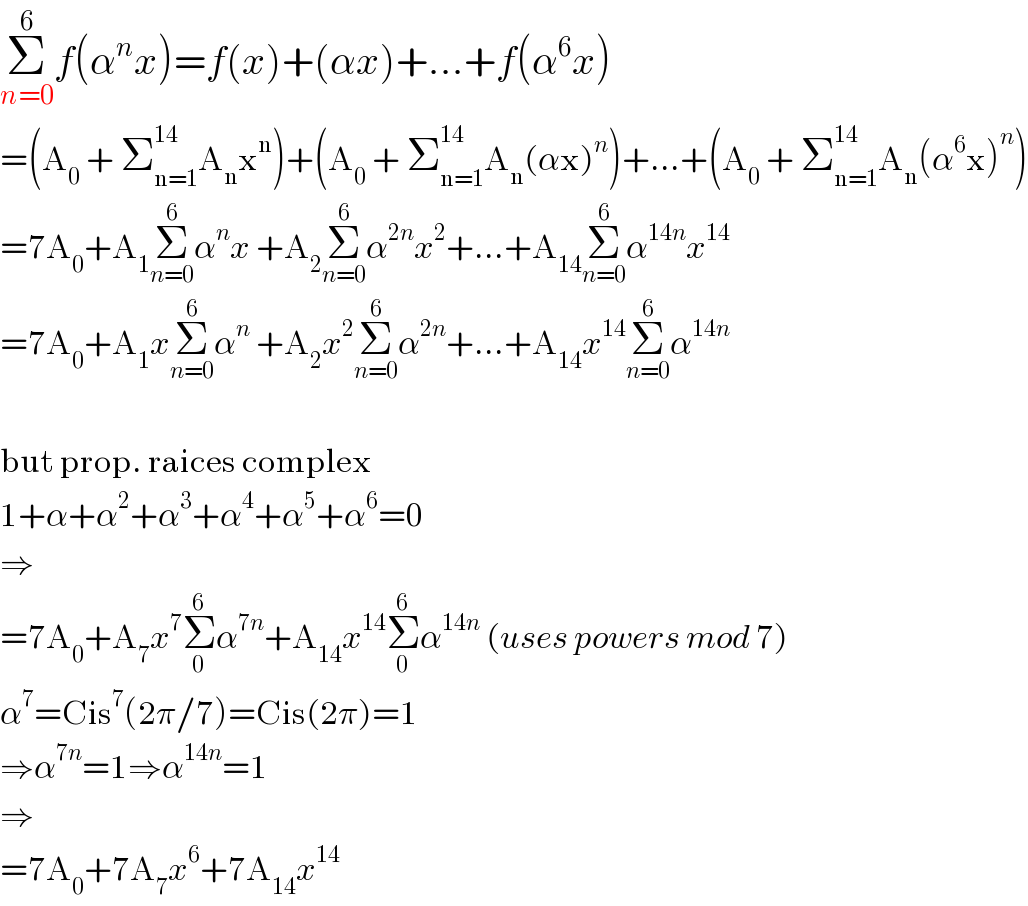
$$\underset{{n}=\mathrm{0}} {\overset{\mathrm{6}} {\sum}}{f}\left(\alpha^{{n}} {x}\right)={f}\left({x}\right)+\left(\alpha{x}\right)+…+{f}\left(\alpha^{\mathrm{6}} {x}\right) \\ $$$$=\left(\mathrm{A}_{\mathrm{0}} \:+\:\sum_{\mathrm{n}=\mathrm{1}} ^{\mathrm{14}} \mathrm{A}_{\mathrm{n}} \mathrm{x}^{\mathrm{n}} \right)+\left(\mathrm{A}_{\mathrm{0}} \:+\:\sum_{\mathrm{n}=\mathrm{1}} ^{\mathrm{14}} \mathrm{A}_{\mathrm{n}} \left(\alpha\mathrm{x}\right)^{{n}} \right)+…+\left(\mathrm{A}_{\mathrm{0}} \:+\:\sum_{\mathrm{n}=\mathrm{1}} ^{\mathrm{14}} \mathrm{A}_{\mathrm{n}} \left(\alpha^{\mathrm{6}} \mathrm{x}\right)^{{n}} \right) \\ $$$$=\mathrm{7A}_{\mathrm{0}} +\mathrm{A}_{\mathrm{1}} \underset{{n}=\mathrm{0}} {\overset{\mathrm{6}} {\sum}}\alpha^{{n}} {x}\:+\mathrm{A}_{\mathrm{2}} \underset{{n}=\mathrm{0}} {\overset{\mathrm{6}} {\sum}}\alpha^{\mathrm{2}{n}} {x}^{\mathrm{2}} +…+\mathrm{A}_{\mathrm{14}} \underset{{n}=\mathrm{0}} {\overset{\mathrm{6}} {\sum}}\alpha^{\mathrm{14}{n}} {x}^{\mathrm{14}} \\ $$$$=\mathrm{7A}_{\mathrm{0}} +\mathrm{A}_{\mathrm{1}} {x}\underset{{n}=\mathrm{0}} {\overset{\mathrm{6}} {\sum}}\alpha^{{n}} \:+\mathrm{A}_{\mathrm{2}} {x}^{\mathrm{2}} \underset{{n}=\mathrm{0}} {\overset{\mathrm{6}} {\sum}}\alpha^{\mathrm{2}{n}} +…+\mathrm{A}_{\mathrm{14}} {x}^{\mathrm{14}} \underset{{n}=\mathrm{0}} {\overset{\mathrm{6}} {\sum}}\alpha^{\mathrm{14}{n}} \\ $$$$ \\ $$$$\mathrm{but}\:\mathrm{prop}.\:\mathrm{raices}\:\mathrm{complex} \\ $$$$\mathrm{1}+\alpha+\alpha^{\mathrm{2}} +\alpha^{\mathrm{3}} +\alpha^{\mathrm{4}} +\alpha^{\mathrm{5}} +\alpha^{\mathrm{6}} =\mathrm{0} \\ $$$$\Rightarrow \\ $$$$=\mathrm{7A}_{\mathrm{0}} +\mathrm{A}_{\mathrm{7}} {x}^{\mathrm{7}} \underset{\mathrm{0}} {\overset{\mathrm{6}} {\sum}}\alpha^{\mathrm{7}{n}} +\mathrm{A}_{\mathrm{14}} {x}^{\mathrm{14}} \underset{\mathrm{0}} {\overset{\mathrm{6}} {\sum}}\alpha^{\mathrm{14}{n}} \:\left({uses}\:{powers}\:{mod}\:\mathrm{7}\right) \\ $$$$\alpha^{\mathrm{7}} =\mathrm{Cis}^{\mathrm{7}} \left(\mathrm{2}\pi/\mathrm{7}\right)=\mathrm{Cis}\left(\mathrm{2}\pi\right)=\mathrm{1} \\ $$$$\Rightarrow\alpha^{\mathrm{7}{n}} =\mathrm{1}\Rightarrow\alpha^{\mathrm{14}{n}} =\mathrm{1} \\ $$$$\Rightarrow \\ $$$$=\mathrm{7A}_{\mathrm{0}} +\mathrm{7A}_{\mathrm{7}} {x}^{\mathrm{6}} +\mathrm{7A}_{\mathrm{14}} {x}^{\mathrm{14}} \\ $$
