Question Number 127974 by Dwaipayan Shikari last updated on 03/Jan/21

$$\overset{\bullet\bullet} {\theta}+\frac{{g}}{{l}}{sin}\theta=\mathrm{0} \\ $$$${Exact}\:{form}\:\left({May}\:{include}\:{elliptic}\:{integral}\right) \\ $$
Commented by Dwaipayan Shikari last updated on 03/Jan/21
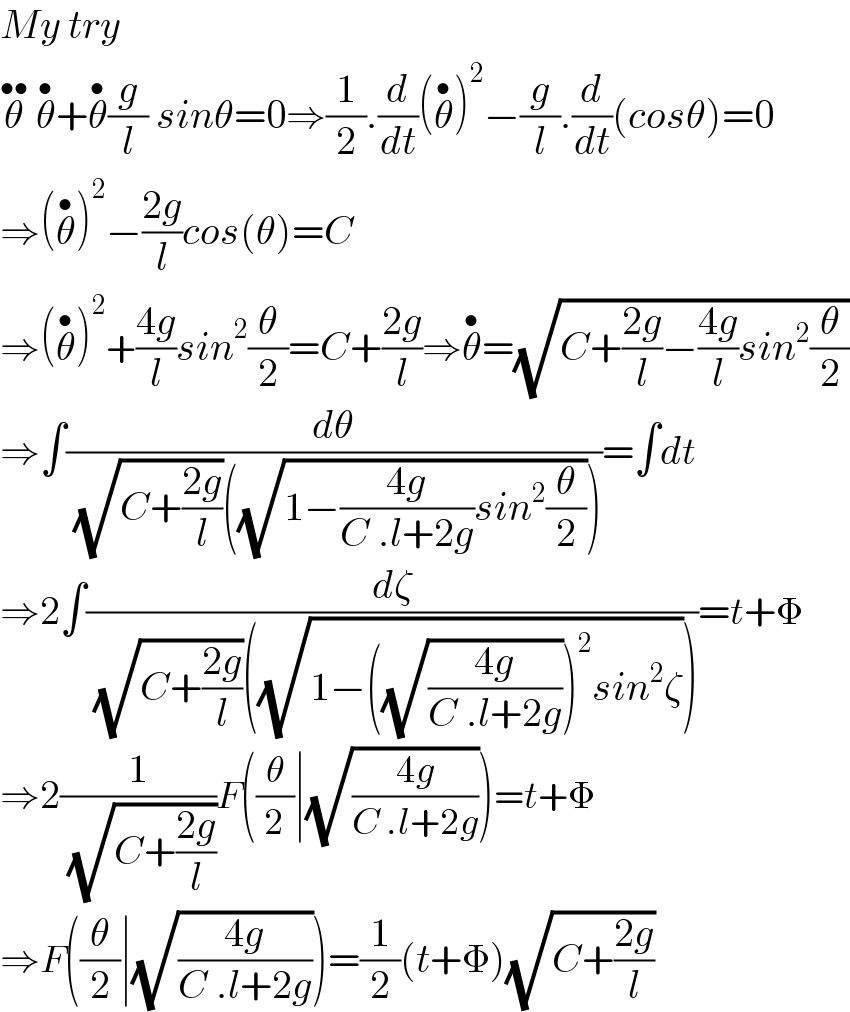
$${My}\:{try} \\ $$$$\overset{\bullet\bullet} {\theta}\:\overset{\bullet} {\theta}+\overset{\bullet} {\theta}\frac{{g}}{{l}}\:{sin}\theta=\mathrm{0}\Rightarrow\frac{\mathrm{1}}{\mathrm{2}}.\frac{{d}}{{dt}}\left(\overset{\bullet} {\theta}\right)^{\mathrm{2}} −\frac{{g}}{{l}}.\frac{{d}}{{dt}}\left({cos}\theta\right)=\mathrm{0} \\ $$$$\Rightarrow\left(\overset{\bullet} {\theta}\right)^{\mathrm{2}} −\frac{\mathrm{2}{g}}{{l}}{cos}\left(\theta\right)={C} \\ $$$$\Rightarrow\left(\overset{\bullet} {\theta}\right)^{\mathrm{2}} +\frac{\mathrm{4}{g}}{{l}}{sin}^{\mathrm{2}} \frac{\theta}{\mathrm{2}}={C}+\frac{\mathrm{2}{g}}{{l}}\Rightarrow\overset{\bullet} {\theta}=\sqrt{{C}+\frac{\mathrm{2}{g}}{{l}}−\frac{\mathrm{4}{g}}{{l}}{sin}^{\mathrm{2}} \frac{\theta}{\mathrm{2}}} \\ $$$$\Rightarrow\int\frac{{d}\theta}{\:\sqrt{{C}+\frac{\mathrm{2}{g}}{{l}}}\left(\sqrt{\mathrm{1}−\frac{\mathrm{4}{g}}{{C}\:.{l}+\mathrm{2}{g}}{sin}^{\mathrm{2}} \frac{\theta}{\mathrm{2}}}\right)}=\int{dt} \\ $$$$\Rightarrow\mathrm{2}\int\frac{{d}\zeta}{\:\sqrt{{C}+\frac{\mathrm{2}{g}}{{l}}}\left(\sqrt{\mathrm{1}−\left(\sqrt{\frac{\mathrm{4}{g}}{{C}\:.{l}+\mathrm{2}{g}}}\right)^{\mathrm{2}} {sin}^{\mathrm{2}} \zeta}\right)}={t}+\Phi \\ $$$$\Rightarrow\mathrm{2}\frac{\mathrm{1}}{\:\sqrt{{C}+\frac{\mathrm{2}{g}}{{l}}}}{F}\left(\frac{\theta}{\mathrm{2}}\mid\sqrt{\frac{\mathrm{4}{g}}{{C}\:.{l}+\mathrm{2}{g}}}\right)={t}+\Phi \\ $$$$\Rightarrow{F}\left(\frac{\theta}{\mathrm{2}}\mid\sqrt{\frac{\mathrm{4}{g}}{{C}\:.{l}+\mathrm{2}{g}}}\right)=\frac{\mathrm{1}}{\mathrm{2}}\left({t}+\Phi\right)\sqrt{{C}+\frac{\mathrm{2}{g}}{{l}}} \\ $$
Answered by mr W last updated on 03/Jan/21
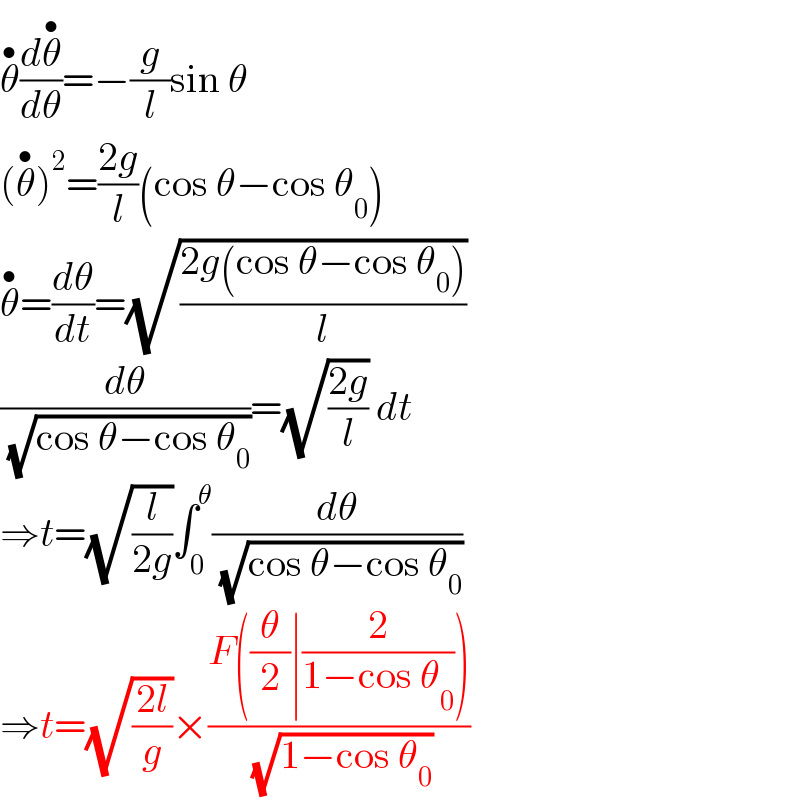
$$\overset{\bullet} {\theta}\frac{{d}\overset{\bullet} {\theta}}{{d}\theta}=−\frac{{g}}{{l}}\mathrm{sin}\:\theta \\ $$$$\left(\overset{\bullet} {\theta}\right)^{\mathrm{2}} =\frac{\mathrm{2}{g}}{{l}}\left(\mathrm{cos}\:\theta−\mathrm{cos}\:\theta_{\mathrm{0}} \right) \\ $$$$\overset{\bullet} {\theta}=\frac{{d}\theta}{{dt}}=\sqrt{\frac{\mathrm{2}{g}\left(\mathrm{cos}\:\theta−\mathrm{cos}\:\theta_{\mathrm{0}} \right)}{{l}}} \\ $$$$\frac{{d}\theta}{\:\sqrt{\mathrm{cos}\:\theta−\mathrm{cos}\:\theta_{\mathrm{0}} }}=\sqrt{\frac{\mathrm{2}{g}}{{l}}}\:{dt} \\ $$$$\Rightarrow{t}=\sqrt{\frac{{l}}{\mathrm{2}{g}}}\int_{\mathrm{0}} ^{\theta} \frac{{d}\theta}{\:\sqrt{\mathrm{cos}\:\theta−\mathrm{cos}\:\theta_{\mathrm{0}} }} \\ $$$$\Rightarrow{t}=\sqrt{\frac{\mathrm{2}{l}}{{g}}}×\frac{{F}\left(\frac{\theta}{\mathrm{2}}\mid\frac{\mathrm{2}}{\mathrm{1}−\mathrm{cos}\:\theta_{\mathrm{0}} }\right)}{\:\sqrt{\mathrm{1}−\mathrm{cos}\:\theta_{\mathrm{0}} }} \\ $$
Commented by Dwaipayan Shikari last updated on 03/Jan/21
$${Thanking}\:{you}\:{sir} \\ $$
