Question Number 127982 by Dwaipayan Shikari last updated on 03/Jan/21

$${Some}\:{Values}\:.. \\ $$$$\underset{{n}=−\infty} {\overset{\infty} {\sum}}{e}^{−\pi{n}^{\mathrm{2}} } =\frac{\pi^{\frac{\mathrm{1}}{\mathrm{4}}} }{\Gamma\left(\frac{\mathrm{3}}{\mathrm{4}}\right)} \\ $$$$\underset{{n}=−\infty} {\overset{\infty} {\sum}}{e}^{−\mathrm{2}\pi{n}^{\mathrm{2}} } =\frac{\pi^{\frac{\mathrm{1}}{\mathrm{4}}} }{\Gamma\left(\frac{\mathrm{3}}{\mathrm{4}}\right)}\:\frac{\sqrt[{\mathrm{4}}]{\mathrm{6}+\mathrm{4}\sqrt{\mathrm{2}}}}{\mathrm{2}} \\ $$$$\underset{{n}=−\infty} {\overset{\infty} {\sum}}{e}^{−\mathrm{6}\pi{n}^{\mathrm{2}} } =\frac{\pi^{\frac{\mathrm{1}}{\mathrm{4}}} }{\Gamma\left(\frac{\mathrm{3}}{\mathrm{4}}\right)}.\frac{\sqrt{\sqrt[{\mathrm{4}}]{\mathrm{1}}+\sqrt[{\mathrm{4}}]{\mathrm{3}}+\sqrt[{\mathrm{4}}]{\mathrm{4}}+\sqrt[{\mathrm{4}}]{\mathrm{9}}}}{\:\sqrt{\mathrm{1728}}} \\ $$$${Any}\:{Idea}\:{to}\:{prove}\:? \\ $$
Commented by Dwaipayan Shikari last updated on 03/Jan/21
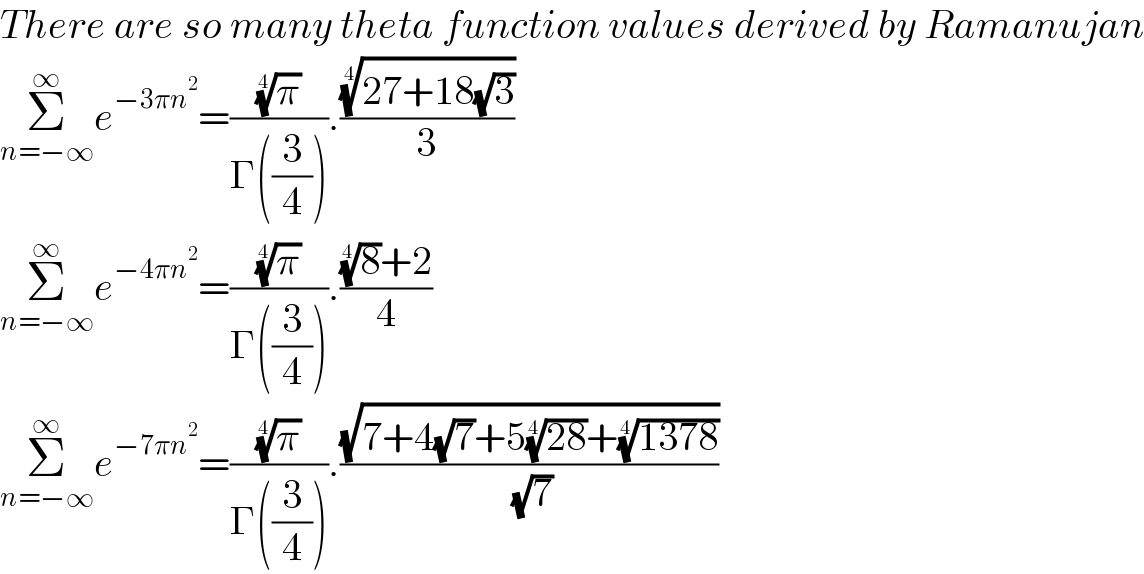
$${There}\:{are}\:{so}\:{many}\:{theta}\:{function}\:{values}\:{derived}\:{by}\:{Ramanujan} \\ $$$$\underset{{n}=−\infty} {\overset{\infty} {\sum}}{e}^{−\mathrm{3}\pi{n}^{\mathrm{2}} } =\frac{\sqrt[{\mathrm{4}}]{\pi}}{\Gamma\left(\frac{\mathrm{3}}{\mathrm{4}}\right)}.\frac{\sqrt[{\mathrm{4}}]{\mathrm{27}+\mathrm{18}\sqrt{\mathrm{3}}}}{\mathrm{3}} \\ $$$$\underset{{n}=−\infty} {\overset{\infty} {\sum}}{e}^{−\mathrm{4}\pi{n}^{\mathrm{2}} } =\frac{\sqrt[{\mathrm{4}}]{\pi}}{\Gamma\left(\frac{\mathrm{3}}{\mathrm{4}}\right)}.\frac{\sqrt[{\mathrm{4}}]{\mathrm{8}}+\mathrm{2}}{\mathrm{4}} \\ $$$$\underset{{n}=−\infty} {\overset{\infty} {\sum}}{e}^{−\mathrm{7}\pi{n}^{\mathrm{2}} } =\frac{\sqrt[{\mathrm{4}}]{\pi}}{\Gamma\left(\frac{\mathrm{3}}{\mathrm{4}}\right)}.\frac{\sqrt{\mathrm{7}+\mathrm{4}\sqrt{\mathrm{7}}+\mathrm{5}\sqrt[{\mathrm{4}}]{\mathrm{28}}+\sqrt[{\mathrm{4}}]{\mathrm{1378}}}}{\:\sqrt{\mathrm{7}}} \\ $$
