Menu Close

There-are-3-tangent-circumferences-inscribed-in-an-isosceles-right-triangle-Two-of-these-circumferences-have-radius-R-and-are-tangent-to-the-hypotenuse-and-to-the-two-cathetus-The-smaller-circumfere

Tinku Tara 0 Comments
Pin




Question Number 71819 by Maclaurin Stickker last updated on 20/Oct/19
There are 3 tangent circumferences inscribed in an isosceles right triangle Two of these circumferences have radius R and are tangent to the hypotenuse and to the two cathetus. The smaller circumference has radius r and is tangent to the two cathetus. How can I find the radius of the smaller circumference as a function of R? (I want a tip on how to solve the problem).
$${There}\:{are}\:\mathrm{3}\:{tangent}\:{circumferences} \\ $$$${inscribed}\:{in}\:{an}\:{isosceles}\:{right}\:{triangle} \\ $$$${Two}\:{of}\:{these}\:{circumferences}\:{have} \\ $$$${radius}\:\boldsymbol{{R}}\:{and}\:{are}\:{tangent}\:{to}\:{the}\: \\ $$$${hypotenuse}\:{and}\:{to}\:{the}\:{two}\:{cathetus}. \\ $$$${The}\:{smaller}\:{circumference}\:{has}\: \\ $$$${radius}\:\boldsymbol{{r}}\:{and}\:{is}\:{tangent}\:{to}\:{the}\:{two} \\ $$$${cathetus}.\:{How}\:{can}\:{I}\:{find}\:{the}\:{radius} \\ $$$${of}\:{the}\:{smaller}\:{circumference}\:{as}\:{a} \\ $$$${function}\:{of}\:\boldsymbol{{R}}? \\ $$$$\left({I}\:{want}\:{a}\:{tip}\:{on}\:{how}\:{to}\:{solve}\:{the}\:\right. \\ $$$$\left.{problem}\right). \\ $$
Commented by mr W last updated on 20/Oct/19
Commented by mr W last updated on 20/Oct/19
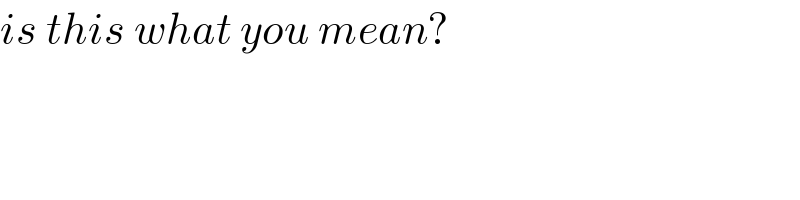
is this what you mean?
$${is}\:{this}\:{what}\:{you}\:{mean}? \\ $$
Commented by Maclaurin Stickker last updated on 20/Oct/19
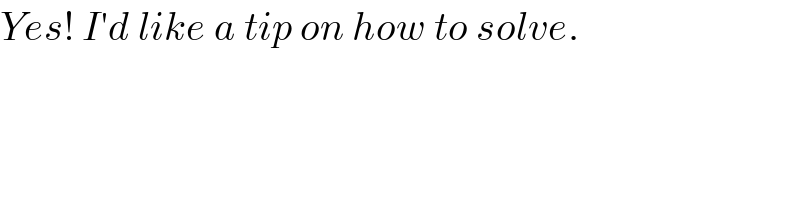
Yes! I′d like a tip on how to solve.
$${Yes}!\:{I}'{d}\:{like}\:{a}\:{tip}\:{on}\:{how}\:{to}\:{solve}. \\ $$
Answered by mr W last updated on 20/Oct/19
Commented by mr W last updated on 20/Oct/19
AB=(√2)r+(√((r+R)^2 −R^2 ))+R=(√2)r+(√(r(2R+r)))+R tan 22.5°=((sin 45°)/(1+cos 45°))=(((√2)/2)/(1+((√2)/2)))=(√2)−1 DC=(R/(tan 22.5°))=(R/( (√2)−1))=((√2)+1)R BC=R+((√2)+1)R=(2+(√2))R AB=BC (√2)r+(√(r(2R+r)))+R=(2+(√2))R (√(r(2R+r)))=(1+(√2))R−(√2)r r(2R+r)=(1+(√2))^2 R^2 +2r^2 −2(1+(√2))(√2)Rr ⇒r^2 −2((√2)+3)Rr+(1+(√2))^2 R^2 =0 ⇒r=(3+(√2)−2(√(2+(√2))))R ⇒r=((√(2+(√2)))−1)^2 R≈0.7187R
$${AB}=\sqrt{\mathrm{2}}{r}+\sqrt{\left({r}+{R}\right)^{\mathrm{2}} −{R}^{\mathrm{2}} }+{R}=\sqrt{\mathrm{2}}{r}+\sqrt{{r}\left(\mathrm{2}{R}+{r}\right)}+{R} \\ $$$$\mathrm{tan}\:\mathrm{22}.\mathrm{5}°=\frac{\mathrm{sin}\:\mathrm{45}°}{\mathrm{1}+\mathrm{cos}\:\mathrm{45}°}=\frac{\frac{\sqrt{\mathrm{2}}}{\mathrm{2}}}{\mathrm{1}+\frac{\sqrt{\mathrm{2}}}{\mathrm{2}}}=\sqrt{\mathrm{2}}−\mathrm{1} \\ $$$${DC}=\frac{{R}}{\mathrm{tan}\:\mathrm{22}.\mathrm{5}°}=\frac{{R}}{\:\sqrt{\mathrm{2}}−\mathrm{1}}=\left(\sqrt{\mathrm{2}}+\mathrm{1}\right){R} \\ $$$${BC}={R}+\left(\sqrt{\mathrm{2}}+\mathrm{1}\right){R}=\left(\mathrm{2}+\sqrt{\mathrm{2}}\right){R} \\ $$$${AB}={BC} \\ $$$$\sqrt{\mathrm{2}}{r}+\sqrt{{r}\left(\mathrm{2}{R}+{r}\right)}+{R}=\left(\mathrm{2}+\sqrt{\mathrm{2}}\right){R} \\ $$$$\sqrt{{r}\left(\mathrm{2}{R}+{r}\right)}=\left(\mathrm{1}+\sqrt{\mathrm{2}}\right){R}−\sqrt{\mathrm{2}}{r} \\ $$$${r}\left(\mathrm{2}{R}+{r}\right)=\left(\mathrm{1}+\sqrt{\mathrm{2}}\right)^{\mathrm{2}} {R}^{\mathrm{2}} +\mathrm{2}{r}^{\mathrm{2}} −\mathrm{2}\left(\mathrm{1}+\sqrt{\mathrm{2}}\right)\sqrt{\mathrm{2}}{Rr} \\ $$$$\Rightarrow{r}^{\mathrm{2}} −\mathrm{2}\left(\sqrt{\mathrm{2}}+\mathrm{3}\right){Rr}+\left(\mathrm{1}+\sqrt{\mathrm{2}}\right)^{\mathrm{2}} {R}^{\mathrm{2}} =\mathrm{0} \\ $$$$\Rightarrow{r}=\left(\mathrm{3}+\sqrt{\mathrm{2}}−\mathrm{2}\sqrt{\mathrm{2}+\sqrt{\mathrm{2}}}\right){R} \\ $$$$\Rightarrow{r}=\left(\sqrt{\mathrm{2}+\sqrt{\mathrm{2}}}−\mathrm{1}\right)^{\mathrm{2}} {R}\approx\mathrm{0}.\mathrm{7187}{R} \\ $$

Pin

Leave a Reply

Your email address will not be published. Required fields are marked *