Question Number 130837 by EDWIN88 last updated on 29/Jan/21
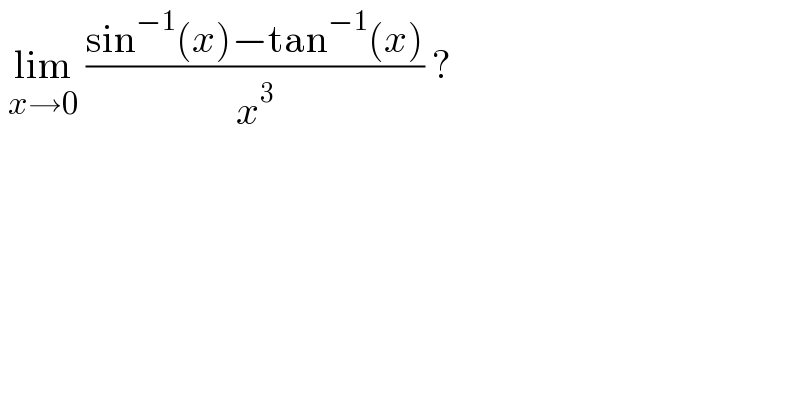
$$\:\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\:\frac{\mathrm{sin}^{−\mathrm{1}} \left({x}\right)−\mathrm{tan}^{−\mathrm{1}} \left({x}\right)}{{x}^{\mathrm{3}} }\:? \\ $$
Answered by bemath last updated on 29/Jan/21

$$\left(\mathrm{1}\right)\mathrm{sin}^{−\mathrm{1}} \left(\mathrm{x}\right)=\mathrm{x}+\frac{\mathrm{x}^{\mathrm{3}} }{\mathrm{3}!}+\frac{\mathrm{1}^{\mathrm{2}} .\mathrm{3}^{\mathrm{2}} \mathrm{x}^{\mathrm{5}} }{\mathrm{5}!}+… \\ $$$$\left(\mathrm{2}\right)\mathrm{tan}^{−\mathrm{1}} \left(\mathrm{x}\right)=\mathrm{x}−\frac{\mathrm{x}^{\mathrm{3}} }{\mathrm{3}}+\frac{\mathrm{x}^{\mathrm{5}} }{\mathrm{5}}−… \\ $$$$\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\:\frac{\left(\mathrm{x}+\frac{\mathrm{x}^{\mathrm{3}} }{\mathrm{6}}\right)−\left(\mathrm{x}−\frac{\mathrm{x}^{\mathrm{3}} }{\mathrm{3}}\right)}{\mathrm{x}^{\mathrm{3}} }\:=\:\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\:\frac{\frac{\mathrm{3}}{\mathrm{6}}\mathrm{x}^{\mathrm{3}} }{\mathrm{x}^{\mathrm{3}} }=\frac{\mathrm{1}}{\mathrm{2}} \\ $$
