Question Number 6407 by FilupSmith last updated on 26/Jun/16

$$\mathrm{Can}\:\mathrm{you}\:\mathrm{evaluate}: \\ $$$$\underset{{x}\rightarrow\infty} {\mathrm{lim}}\:\left(−\mathrm{1}\right)^{{x}} {x} \\ $$$$ \\ $$$$\mathrm{WolframAlpha}\:\mathrm{writes}\:\mathrm{the}\:\mathrm{answer}\:\mathrm{as} \\ $$$$={e}^{\mathrm{2}{i}\:\:\mathrm{to}\:\:\pi} \infty \\ $$
Commented by FilupSmith last updated on 26/Jun/16

$$\mathrm{Is}\:\mathrm{there}\:\mathrm{a}\:\mathrm{more}\:\mathrm{formal}\:\mathrm{way}\:\mathrm{to}\:\mathrm{write} \\ $$$$\mathrm{the}\:\mathrm{answer}? \\ $$
Commented by FilupSmith last updated on 26/Jun/16
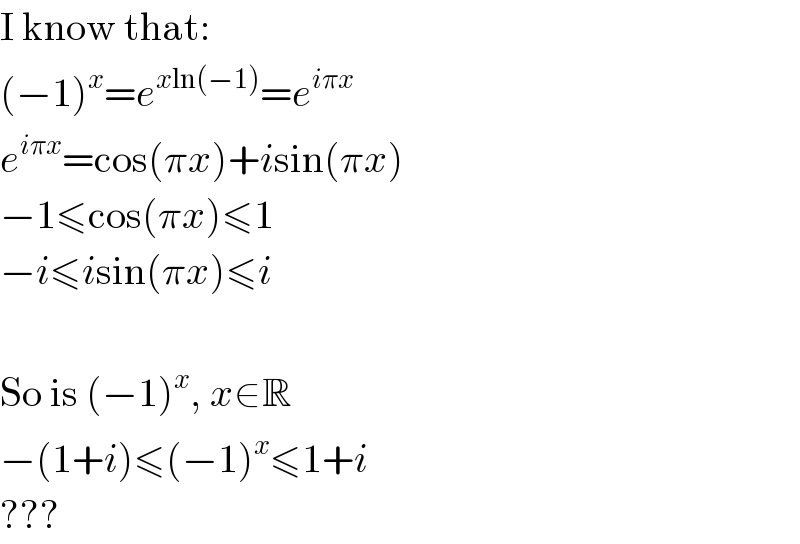
$$\mathrm{I}\:\mathrm{know}\:\mathrm{that}: \\ $$$$\left(−\mathrm{1}\right)^{{x}} ={e}^{{x}\mathrm{ln}\left(−\mathrm{1}\right)} ={e}^{{i}\pi{x}} \\ $$$${e}^{{i}\pi{x}} =\mathrm{cos}\left(\pi{x}\right)+{i}\mathrm{sin}\left(\pi{x}\right) \\ $$$$−\mathrm{1}\leqslant\mathrm{cos}\left(\pi{x}\right)\leqslant\mathrm{1} \\ $$$$−{i}\leqslant{i}\mathrm{sin}\left(\pi{x}\right)\leqslant{i} \\ $$$$ \\ $$$$\mathrm{So}\:\mathrm{is}\:\left(−\mathrm{1}\right)^{{x}} ,\:{x}\in\mathbb{R} \\ $$$$−\left(\mathrm{1}+{i}\right)\leqslant\left(−\mathrm{1}\right)^{{x}} \leqslant\mathrm{1}+{i} \\ $$$$??? \\ $$
Answered by Temp last updated on 27/Jun/16

$$\left(−\mathrm{1}\right)^{{x}} ={e}^{{i}\pi{x}} \\ $$$${e}^{{i}\pi{x}} =\mathrm{cos}\left({x}\pi\right)+{i}\mathrm{sin}\left({x}\pi\right) \\ $$$$ \\ $$$$\Re\left({e}^{{i}\pi{x}} \right)=\mathrm{cos}\left(\pi{x}\right) \\ $$$$−\mathrm{1}\leqslant\Re\left({e}^{{i}\pi{x}} \right)\leqslant\mathrm{1} \\ $$$$ \\ $$$$\Im\left({e}^{{i}\pi{x}} \right)=\mathrm{sin}\left(\pi{x}\right) \\ $$$$−\mathrm{1}\leqslant\Im\left({e}^{{i}\pi{x}} \right)\leqslant\mathrm{1} \\ $$$$ \\ $$$$\mathrm{Let}\:{L}=\underset{{x}\rightarrow\infty} {\mathrm{lim}}\left({e}^{{i}\pi{x}} \right) \\ $$$$\mathrm{if}\:{L}\in\mathbb{R}\Rightarrow{x}\in\mathbb{Z}:−\mathrm{1}\leqslant{x}\leqslant\mathrm{1} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\therefore\left(−\mathrm{1}\right)^{{x}} =\begin{cases}{\mathrm{1}\:\:\:\mathrm{if}\:\mathrm{2}\mid{x}}\\{−\mathrm{1}\:\:\mathrm{if}\:\mathrm{2}\nmid{x}}\end{cases} \\ $$$$\mathrm{if}\:{L}\in\mathbb{C}\Rightarrow{x}\in\mathbb{R} \\ $$$$−\left({i}+\mathrm{1}\right)\leqslant{L}\leqslant\left({i}+\mathrm{1}\right) \\ $$
Commented by Temp last updated on 27/Jun/16

$$\mathrm{Im}\left({x}\right)=\Im\left({x}\right) \\ $$$$\mathrm{Re}\left({x}\right)=\Re\left({x}\right) \\ $$
