Question Number 55089 by eso last updated on 17/Feb/19
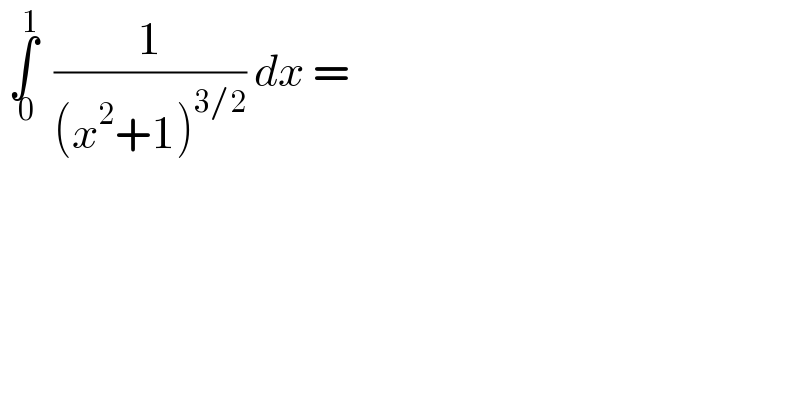
$$\:\underset{\:\mathrm{0}} {\overset{\mathrm{1}} {\int}}\:\:\frac{\mathrm{1}}{\left({x}^{\mathrm{2}} +\mathrm{1}\right)^{\mathrm{3}/\mathrm{2}} }\:{dx}\:= \\ $$
Commented by maxmathsup by imad last updated on 17/Feb/19
![let I =∫_0 ^1 (dx/((1+x^2 )^(3/2) )) changement x =tant give I =∫_0 ^(π/4) ((1+tan^2 t)/((1+tan^2 t)^(3/2) ))dt = ∫_0 ^(π/4) (dt/((1+tan^2 t)^(1/2) )) =∫_0 ^(π/4) (cos^2 t)^(1/2) dt =∫_0 ^(π/4) cost dt =[sint]_0 ^(π/4) =((√2)/2)](https://www.tinkutara.com/question/Q55090.png)
$${let}\:{I}\:=\int_{\mathrm{0}} ^{\mathrm{1}} \:\:\frac{{dx}}{\left(\mathrm{1}+{x}^{\mathrm{2}} \right)^{\frac{\mathrm{3}}{\mathrm{2}}} }\:\:\:{changement}\:{x}\:={tant}\:{give}\: \\ $$$${I}\:=\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{4}}} \:\:\:\:\frac{\mathrm{1}+{tan}^{\mathrm{2}} {t}}{\left(\mathrm{1}+{tan}^{\mathrm{2}} {t}\right)^{\frac{\mathrm{3}}{\mathrm{2}}} }{dt}\:=\:\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{4}}} \:\:\:\frac{{dt}}{\left(\mathrm{1}+{tan}^{\mathrm{2}} {t}\right)^{\frac{\mathrm{1}}{\mathrm{2}}} }\:=\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{4}}} \:\:\left({cos}^{\mathrm{2}} {t}\right)^{\frac{\mathrm{1}}{\mathrm{2}}} \:{dt} \\ $$$$=\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{4}}} \:{cost}\:{dt}\:=\left[{sint}\right]_{\mathrm{0}} ^{\frac{\pi}{\mathrm{4}}} \:=\frac{\sqrt{\mathrm{2}}}{\mathrm{2}} \\ $$
