Question Number 63490 by kumaranjul306@gmail.com last updated on 04/Jul/19

$$\mathrm{A}\:\mathrm{father}\:\mathrm{with}\:\mathrm{8}\:\mathrm{children}\:\mathrm{takes}\:\mathrm{3}\:\mathrm{at}\:\mathrm{a}\: \\ $$$$\mathrm{time}\:\mathrm{to}\:\mathrm{the}\:\mathrm{garden}\:\mathrm{as}\:\mathrm{often}\:\mathrm{as}\:\mathrm{he} \\ $$$$\mathrm{without}\:\mathrm{taking}\:\mathrm{the}\:\mathrm{same}\:\mathrm{3}\:\mathrm{children} \\ $$$$\mathrm{together}\:\mathrm{more}\:\mathrm{than}\:\mathrm{once}.\:\mathrm{The}\:\mathrm{number} \\ $$$$\mathrm{of}\:\mathrm{times}\:\mathrm{he}\:\mathrm{will}\:\mathrm{go}\:\mathrm{to}\:\mathrm{the}\:\mathrm{garden}\:\mathrm{is} \\ $$
Commented by Prithwish sen last updated on 05/Jul/19
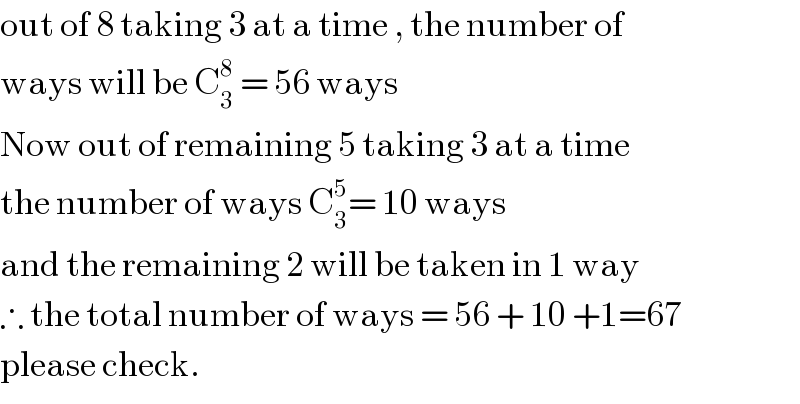
$$\mathrm{out}\:\mathrm{of}\:\mathrm{8}\:\mathrm{taking}\:\mathrm{3}\:\mathrm{at}\:\mathrm{a}\:\mathrm{time}\:,\:\mathrm{the}\:\mathrm{number}\:\mathrm{of} \\ $$$$\mathrm{ways}\:\mathrm{will}\:\mathrm{be}\:\mathrm{C}_{\mathrm{3}} ^{\mathrm{8}} \:=\:\mathrm{56}\:\mathrm{ways} \\ $$$$\mathrm{Now}\:\mathrm{out}\:\mathrm{of}\:\mathrm{remaining}\:\mathrm{5}\:\mathrm{taking}\:\mathrm{3}\:\mathrm{at}\:\mathrm{a}\:\mathrm{time} \\ $$$$\mathrm{the}\:\mathrm{number}\:\mathrm{of}\:\mathrm{ways}\:\mathrm{C}_{\mathrm{3}} ^{\mathrm{5}} =\:\mathrm{10}\:\mathrm{ways} \\ $$$$\mathrm{and}\:\mathrm{the}\:\mathrm{remaining}\:\mathrm{2}\:\mathrm{will}\:\mathrm{be}\:\mathrm{taken}\:\mathrm{in}\:\mathrm{1}\:\mathrm{way} \\ $$$$\therefore\:\mathrm{the}\:\mathrm{total}\:\mathrm{number}\:\mathrm{of}\:\mathrm{ways}\:=\:\mathrm{56}\:+\:\mathrm{10}\:+\mathrm{1}=\mathrm{67} \\ $$$$\mathrm{please}\:\mathrm{check}. \\ $$
Answered by Rio Michael last updated on 05/Jul/19
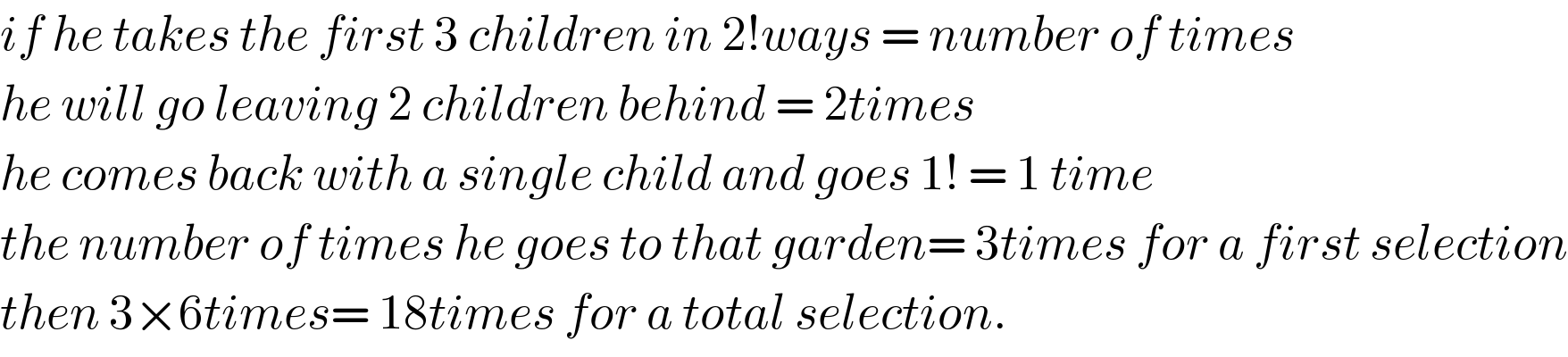
$${if}\:{he}\:{takes}\:{the}\:{first}\:\mathrm{3}\:{children}\:{in}\:\mathrm{2}!{ways}\:=\:{number}\:{of}\:{times} \\ $$$${he}\:{will}\:{go}\:{leaving}\:\mathrm{2}\:{children}\:{behind}\:=\:\mathrm{2}{times} \\ $$$${he}\:{comes}\:{back}\:{with}\:{a}\:{single}\:{child}\:{and}\:{goes}\:\mathrm{1}!\:=\:\mathrm{1}\:{time} \\ $$$${the}\:{number}\:{of}\:{times}\:{he}\:{goes}\:{to}\:{that}\:{garden}=\:\mathrm{3}{times}\:{for}\:{a}\:{first}\:{selection} \\ $$$${then}\:\mathrm{3}×\mathrm{6}{times}=\:\mathrm{18}{times}\:{for}\:{a}\:{total}\:{selection}. \\ $$
Answered by ajfour last updated on 05/Jul/19
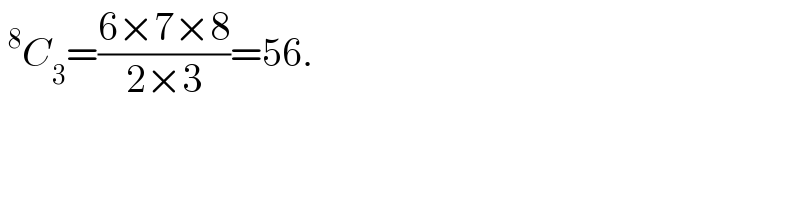
$$\:^{\mathrm{8}} {C}_{\mathrm{3}} =\frac{\mathrm{6}×\mathrm{7}×\mathrm{8}}{\mathrm{2}×\mathrm{3}}=\mathrm{56}. \\ $$
