Question Number 66064 by mathmax by abdo last updated on 08/Aug/19
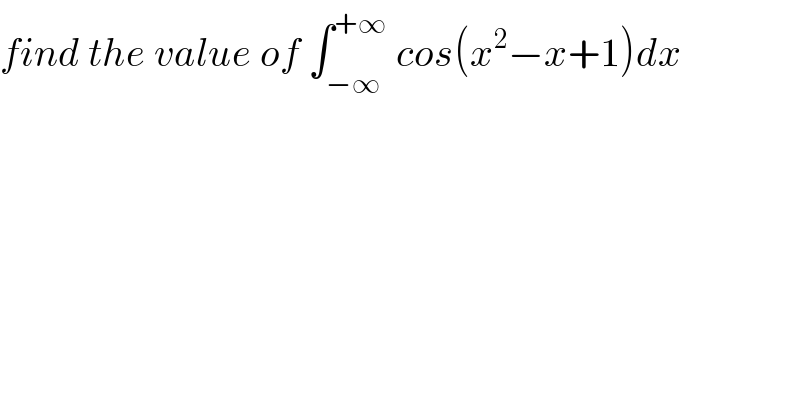
$${find}\:{the}\:{value}\:{of}\:\int_{−\infty} ^{+\infty} \:{cos}\left({x}^{\mathrm{2}} −{x}+\mathrm{1}\right){dx} \\ $$
Commented by mathmax by abdo last updated on 09/Aug/19
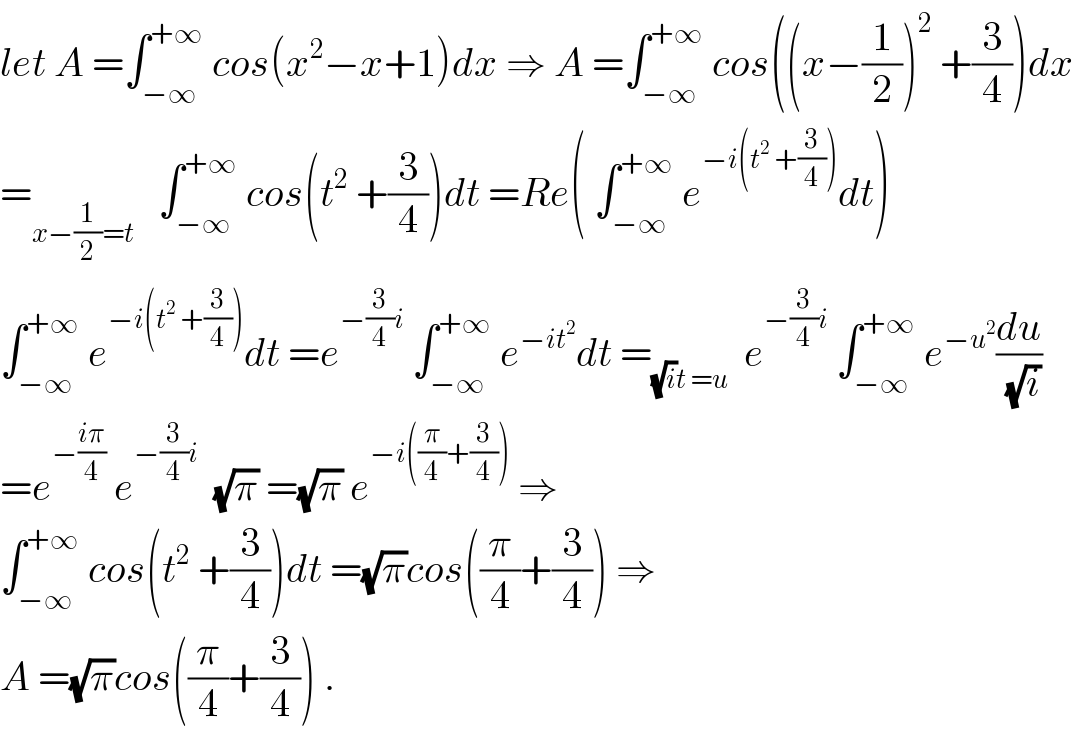
$${let}\:{A}\:=\int_{−\infty} ^{+\infty} \:{cos}\left({x}^{\mathrm{2}} −{x}+\mathrm{1}\right){dx}\:\Rightarrow\:{A}\:=\int_{−\infty} ^{+\infty} \:{cos}\left(\left({x}−\frac{\mathrm{1}}{\mathrm{2}}\right)^{\mathrm{2}} \:+\frac{\mathrm{3}}{\mathrm{4}}\right){dx} \\ $$$$=_{{x}−\frac{\mathrm{1}}{\mathrm{2}}={t}} \:\:\:\int_{−\infty} ^{+\infty} \:{cos}\left({t}^{\mathrm{2}} \:+\frac{\mathrm{3}}{\mathrm{4}}\right){dt}\:={Re}\left(\:\int_{−\infty} ^{+\infty} \:{e}^{−{i}\left({t}^{\mathrm{2}} \:+\frac{\mathrm{3}}{\mathrm{4}}\right)} {dt}\right) \\ $$$$\int_{−\infty} ^{+\infty} \:{e}^{−{i}\left({t}^{\mathrm{2}} \:+\frac{\mathrm{3}}{\mathrm{4}}\right)} {dt}\:={e}^{−\frac{\mathrm{3}}{\mathrm{4}}{i}} \:\int_{−\infty} ^{+\infty} \:{e}^{−{it}^{\mathrm{2}} } {dt}\:=_{\sqrt{{i}}{t}\:={u}} \:\:{e}^{−\frac{\mathrm{3}}{\mathrm{4}}{i}} \:\int_{−\infty} ^{+\infty} \:{e}^{−{u}^{\mathrm{2}} } \frac{{du}}{\:\sqrt{{i}}} \\ $$$$={e}^{−\frac{{i}\pi}{\mathrm{4}}} \:{e}^{−\frac{\mathrm{3}}{\mathrm{4}}{i}} \:\:\sqrt{\pi}\:=\sqrt{\pi}\:{e}^{−{i}\left(\frac{\pi}{\mathrm{4}}+\frac{\mathrm{3}}{\mathrm{4}}\right)} \:\Rightarrow \\ $$$$\int_{−\infty} ^{+\infty} \:{cos}\left({t}^{\mathrm{2}} \:+\frac{\mathrm{3}}{\mathrm{4}}\right){dt}\:=\sqrt{\pi}{cos}\left(\frac{\pi}{\mathrm{4}}+\frac{\mathrm{3}}{\mathrm{4}}\right)\:\Rightarrow \\ $$$${A}\:=\sqrt{\pi}{cos}\left(\frac{\pi}{\mathrm{4}}+\frac{\mathrm{3}}{\mathrm{4}}\right)\:. \\ $$
