Question Number 66071 by AnjanDey last updated on 08/Aug/19
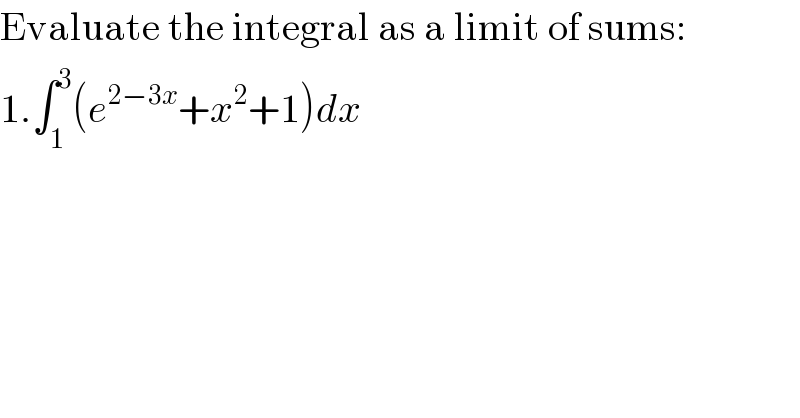
$$\mathrm{Evaluate}\:\mathrm{the}\:\mathrm{integral}\:\mathrm{as}\:\mathrm{a}\:\mathrm{limit}\:\mathrm{of}\:\mathrm{sums}: \\ $$$$\mathrm{1}.\int_{\mathrm{1}} ^{\mathrm{3}} \left({e}^{\mathrm{2}−\mathrm{3}{x}} +{x}^{\mathrm{2}} +\mathrm{1}\right){dx} \\ $$
Answered by meme last updated on 08/Aug/19
![=∫_1 ^3 e^(2−3x) dx+∫_1 ^3 x^2 dx+∫_1 ^3 dx =−3 ∫_1 ^3 −(1/3)e^(2−3x) dx+∫_1 ^3 x^2 dx+∫_1 ^3 dx = −3[e^(2−3x) ]_1 ^3 +(1/3)[x^3 ]_1 ^3 +[x]_1 ^3 =−3e^(−7) +3e^(−1) +9−(1/3)+3−1 = 3(e^(−1) −e^(−7) )+((32)/3)](https://www.tinkutara.com/question/Q66074.png)
$$ \\ $$$$\:\:\:\:=\int_{\mathrm{1}} ^{\mathrm{3}} {e}^{\mathrm{2}−\mathrm{3}{x}} {dx}+\int_{\mathrm{1}} ^{\mathrm{3}} {x}^{\mathrm{2}} {dx}+\int_{\mathrm{1}} ^{\mathrm{3}} {dx} \\ $$$$\:\:\:\:\:=−\mathrm{3}\:\int_{\mathrm{1}} ^{\mathrm{3}} −\frac{\mathrm{1}}{\mathrm{3}}{e}^{\mathrm{2}−\mathrm{3}{x}} {dx}+\int_{\mathrm{1}} ^{\mathrm{3}} {x}^{\mathrm{2}} {dx}+\int_{\mathrm{1}} ^{\mathrm{3}} {dx} \\ $$$$=\:−\mathrm{3}\left[{e}^{\mathrm{2}−\mathrm{3}{x}} \right]_{\mathrm{1}} ^{\mathrm{3}} +\frac{\mathrm{1}}{\mathrm{3}}\left[{x}^{\mathrm{3}} \right]_{\mathrm{1}} ^{\mathrm{3}} +\left[{x}\right]_{\mathrm{1}} ^{\mathrm{3}} \\ $$$$=−\mathrm{3}{e}^{−\mathrm{7}} +\mathrm{3}{e}^{−\mathrm{1}} +\mathrm{9}−\frac{\mathrm{1}}{\mathrm{3}}+\mathrm{3}−\mathrm{1} \\ $$$$=\:\mathrm{3}\left({e}^{−\mathrm{1}} −{e}^{−\mathrm{7}} \right)+\frac{\mathrm{32}}{\mathrm{3}} \\ $$
Commented by AnjanDey last updated on 09/Aug/19
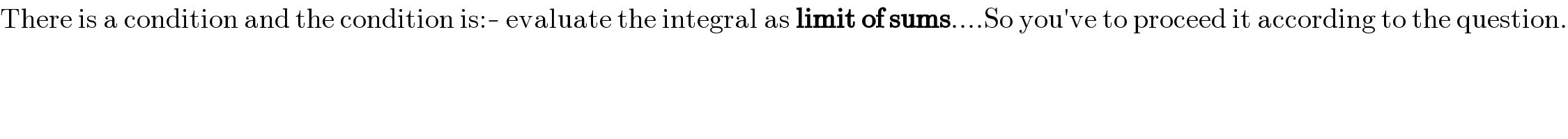
$$\mathrm{There}\:\mathrm{is}\:\mathrm{a}\:\mathrm{condition}\:\mathrm{and}\:\mathrm{the}\:\mathrm{condition}\:\mathrm{is}:-\:\mathrm{evaluate}\:\mathrm{the}\:\mathrm{integral}\:\mathrm{as}\:\boldsymbol{\mathrm{limit}}\:\boldsymbol{\mathrm{of}}\:\boldsymbol{\mathrm{sums}}….\mathrm{So}\:\mathrm{you}'\mathrm{ve}\:\mathrm{to}\:\mathrm{proceed}\:\mathrm{it}\:\mathrm{according}\:\mathrm{to}\:\mathrm{the}\:\mathrm{question}. \\ $$
