Question Number 548 by 123456 last updated on 25/Jan/15
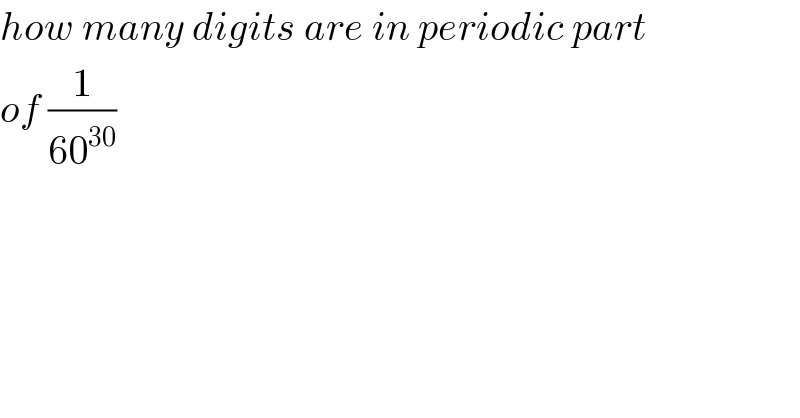
$${how}\:{many}\:{digits}\:{are}\:{in}\:{periodic}\:{part} \\ $$$${of}\:\frac{\mathrm{1}}{\mathrm{60}^{\mathrm{30}} } \\ $$
Answered by prakash jain last updated on 25/Jan/15
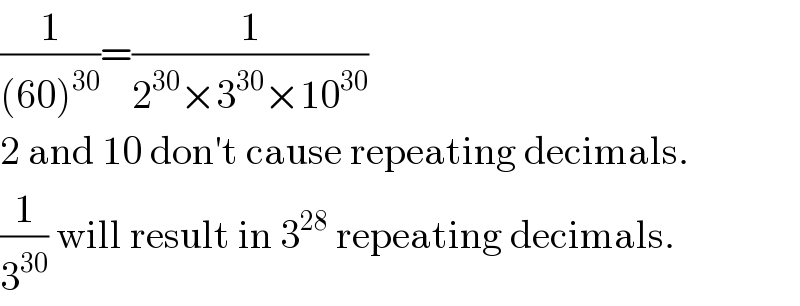
$$\frac{\mathrm{1}}{\left(\mathrm{60}\right)^{\mathrm{30}} }=\frac{\mathrm{1}}{\mathrm{2}^{\mathrm{30}} ×\mathrm{3}^{\mathrm{30}} ×\mathrm{10}^{\mathrm{30}} } \\ $$$$\mathrm{2}\:\mathrm{and}\:\mathrm{10}\:\mathrm{don}'\mathrm{t}\:\mathrm{cause}\:\mathrm{repeating}\:\mathrm{decimals}. \\ $$$$\frac{\mathrm{1}}{\mathrm{3}^{\mathrm{30}} }\:\mathrm{will}\:\mathrm{result}\:\mathrm{in}\:\mathrm{3}^{\mathrm{28}} \:\mathrm{repeating}\:\mathrm{decimals}. \\ $$
