Menu Close

Question-72606

Tinku Tara 0 Comments
Pin




Question Number 72606 by TawaTawa last updated on 30/Oct/19
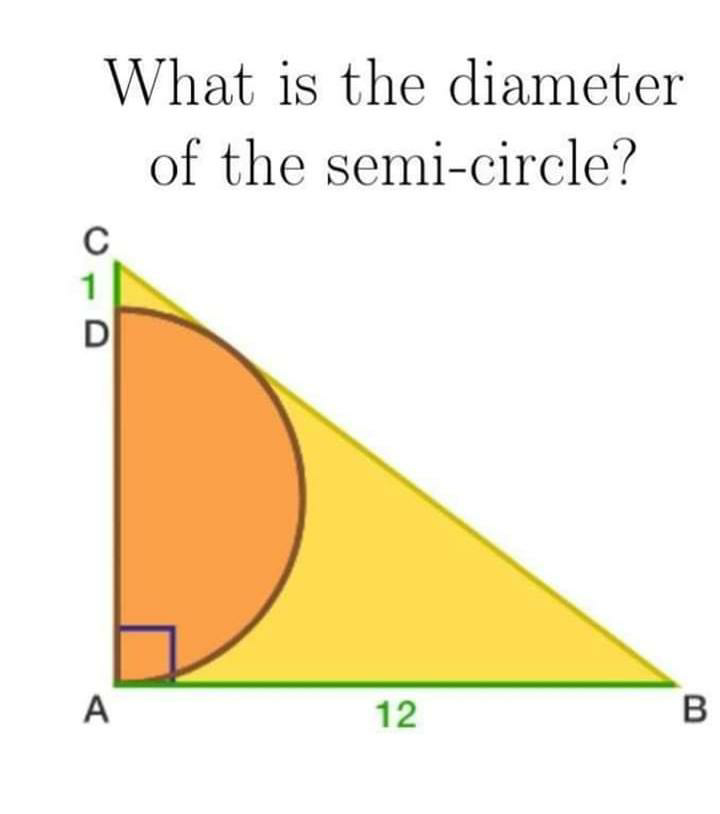
Answered by mr W last updated on 30/Oct/19
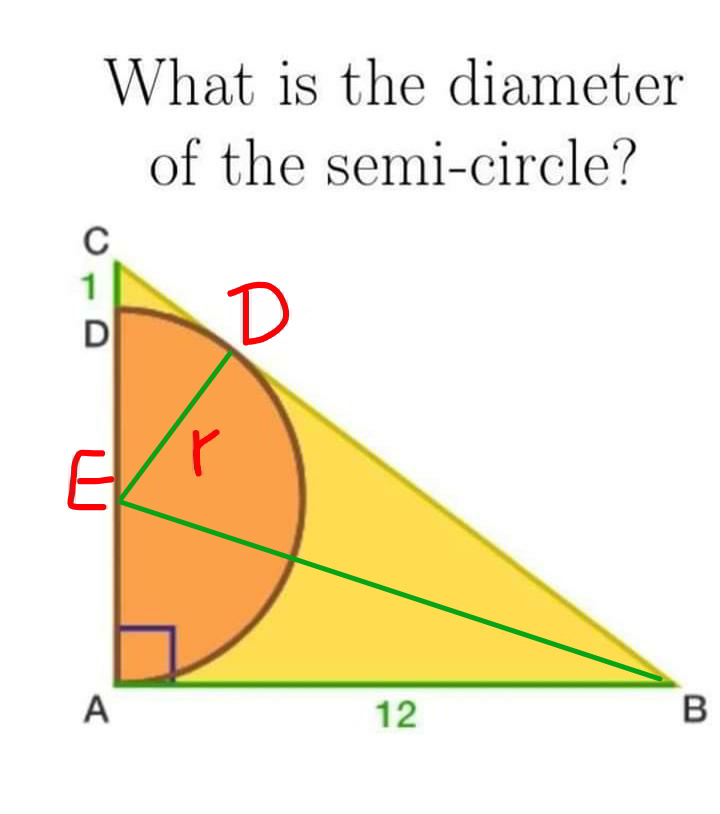
Commented by mr W last updated on 30/Oct/19
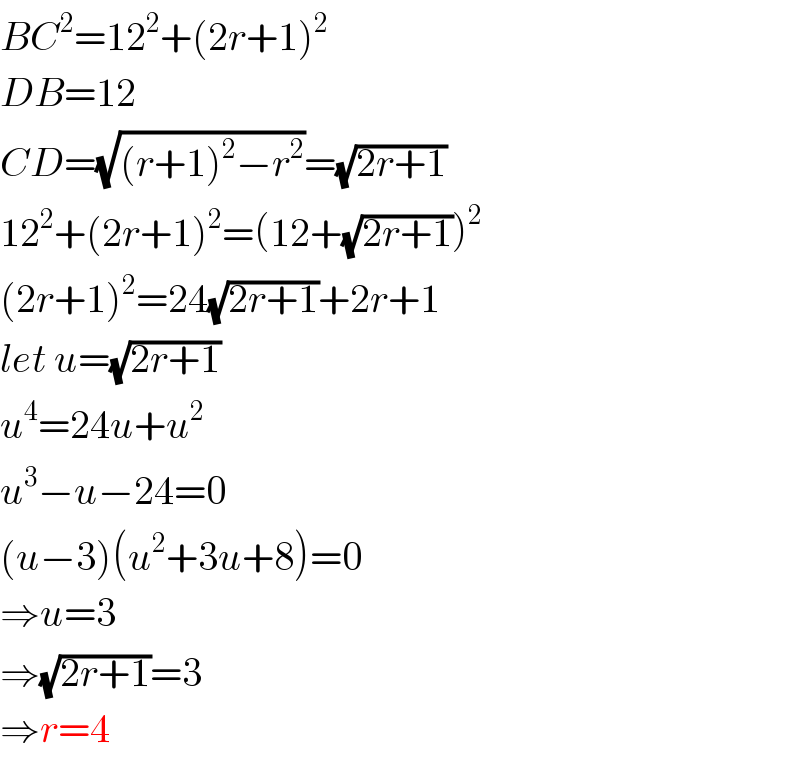
BC^2 =12^2 +(2r+1)^2 DB=12 CD=(√((r+1)^2 −r^2 ))=(√(2r+1)) 12^2 +(2r+1)^2 =(12+(√(2r+1)))^2 (2r+1)^2 =24(√(2r+1))+2r+1 let u=(√(2r+1)) u^4 =24u+u^2 u^3 −u−24=0 (u−3)(u^2 +3u+8)=0 ⇒u=3 ⇒(√(2r+1))=3 ⇒r=4
$${BC}^{\mathrm{2}} =\mathrm{12}^{\mathrm{2}} +\left(\mathrm{2}{r}+\mathrm{1}\right)^{\mathrm{2}} \\ $$$${DB}=\mathrm{12} \\ $$$${CD}=\sqrt{\left({r}+\mathrm{1}\right)^{\mathrm{2}} −{r}^{\mathrm{2}} }=\sqrt{\mathrm{2}{r}+\mathrm{1}} \\ $$$$\mathrm{12}^{\mathrm{2}} +\left(\mathrm{2}{r}+\mathrm{1}\right)^{\mathrm{2}} =\left(\mathrm{12}+\sqrt{\mathrm{2}{r}+\mathrm{1}}\right)^{\mathrm{2}} \\ $$$$\left(\mathrm{2}{r}+\mathrm{1}\right)^{\mathrm{2}} =\mathrm{24}\sqrt{\mathrm{2}{r}+\mathrm{1}}+\mathrm{2}{r}+\mathrm{1} \\ $$$${let}\:{u}=\sqrt{\mathrm{2}{r}+\mathrm{1}} \\ $$$${u}^{\mathrm{4}} =\mathrm{24}{u}+{u}^{\mathrm{2}} \\ $$$${u}^{\mathrm{3}} −{u}−\mathrm{24}=\mathrm{0} \\ $$$$\left({u}−\mathrm{3}\right)\left({u}^{\mathrm{2}} +\mathrm{3}{u}+\mathrm{8}\right)=\mathrm{0} \\ $$$$\Rightarrow{u}=\mathrm{3} \\ $$$$\Rightarrow\sqrt{\mathrm{2}{r}+\mathrm{1}}=\mathrm{3} \\ $$$$\Rightarrow{r}=\mathrm{4} \\ $$
Commented by TawaTawa last updated on 30/Oct/19
God bless you sir.
$$\mathrm{God}\:\mathrm{bless}\:\mathrm{you}\:\mathrm{sir}. \\ $$
Answered by Tanmay chaudhury last updated on 30/Oct/19
circle x^2 +(y−r)^2 =r^2 (x/(12))+(y/(2r+1))=1 is tangent→BC distance from(0,r) to BC is radius (2r+1)x+12y−12(2r+1)=0 ∣(((2r+1)×0+12(r)−24r−12)/( (√((2r+1)^2 +12^2 ))))∣=r −12r−12=r×(√(4r^2 +4r+1+144)) 144r^2 +144+288r=4r^4 +4r^3 +145r^2 4r^4 +4r^3 +r^2 −288r−144=0 r^2 (4r^2 +4r+1)−144(2r+1)=0 r^2 (2r+1)^2 −144(2r+1)=0 (2r+1)(2r^3 +r^2 −144)=0 r≠−0.5 2r^3 +r^2 −128−16=0 2(r^3 −64)+(r+4)(r−4)=0 2(r−4)(r^2 +4r+16)+(r+4)(r−4)=0 (r−4)(2r^2 +8r+32+r+4)=0 so r=4 answer
$${circle}\:{x}^{\mathrm{2}} +\left({y}−{r}\right)^{\mathrm{2}} ={r}^{\mathrm{2}} \\ $$$$\frac{{x}}{\mathrm{12}}+\frac{{y}}{\mathrm{2}{r}+\mathrm{1}}=\mathrm{1}\:{is}\:{tangent}\rightarrow{BC} \\ $$$${distance}\:{from}\left(\mathrm{0},{r}\right)\:{to}\:{BC}\:{is}\:{radius} \\ $$$$\left(\mathrm{2}{r}+\mathrm{1}\right){x}+\mathrm{12}{y}−\mathrm{12}\left(\mathrm{2}{r}+\mathrm{1}\right)=\mathrm{0} \\ $$$$\mid\frac{\left(\mathrm{2}{r}+\mathrm{1}\right)×\mathrm{0}+\mathrm{12}\left({r}\right)−\mathrm{24}{r}−\mathrm{12}}{\:\sqrt{\left(\mathrm{2}{r}+\mathrm{1}\right)^{\mathrm{2}} +\mathrm{12}^{\mathrm{2}} }}\mid={r} \\ $$$$−\mathrm{12}{r}−\mathrm{12}={r}×\sqrt{\mathrm{4}{r}^{\mathrm{2}} +\mathrm{4}{r}+\mathrm{1}+\mathrm{144}}\: \\ $$$$\mathrm{144}{r}^{\mathrm{2}} +\mathrm{144}+\mathrm{288}{r}=\mathrm{4}{r}^{\mathrm{4}} +\mathrm{4}{r}^{\mathrm{3}} +\mathrm{145}{r}^{\mathrm{2}} \\ $$$$\mathrm{4}{r}^{\mathrm{4}} +\mathrm{4}{r}^{\mathrm{3}} +{r}^{\mathrm{2}} −\mathrm{288}{r}−\mathrm{144}=\mathrm{0} \\ $$$${r}^{\mathrm{2}} \left(\mathrm{4}{r}^{\mathrm{2}} +\mathrm{4}{r}+\mathrm{1}\right)−\mathrm{144}\left(\mathrm{2}{r}+\mathrm{1}\right)=\mathrm{0} \\ $$$${r}^{\mathrm{2}} \left(\mathrm{2}{r}+\mathrm{1}\right)^{\mathrm{2}} −\mathrm{144}\left(\mathrm{2}{r}+\mathrm{1}\right)=\mathrm{0} \\ $$$$\left(\mathrm{2}{r}+\mathrm{1}\right)\left(\mathrm{2}{r}^{\mathrm{3}} +{r}^{\mathrm{2}} −\mathrm{144}\right)=\mathrm{0} \\ $$$${r}\neq−\mathrm{0}.\mathrm{5} \\ $$$$\mathrm{2}{r}^{\mathrm{3}} +{r}^{\mathrm{2}} −\mathrm{128}−\mathrm{16}=\mathrm{0} \\ $$$$\mathrm{2}\left({r}^{\mathrm{3}} −\mathrm{64}\right)+\left({r}+\mathrm{4}\right)\left({r}−\mathrm{4}\right)=\mathrm{0} \\ $$$$\mathrm{2}\left({r}−\mathrm{4}\right)\left({r}^{\mathrm{2}} +\mathrm{4}{r}+\mathrm{16}\right)+\left({r}+\mathrm{4}\right)\left({r}−\mathrm{4}\right)=\mathrm{0} \\ $$$$\left({r}−\mathrm{4}\right)\left(\mathrm{2}{r}^{\mathrm{2}} +\mathrm{8}{r}+\mathrm{32}+{r}+\mathrm{4}\right)=\mathrm{0} \\ $$$${so}\:\:{r}=\mathrm{4}\:{answer} \\ $$
Commented by TawaTawa last updated on 30/Oct/19
God bless you sir.
$$\mathrm{God}\:\mathrm{bless}\:\mathrm{you}\:\mathrm{sir}. \\ $$

Pin

Leave a Reply

Your email address will not be published. Required fields are marked *