Question Number 131729 by ajfour last updated on 07/Feb/21

Commented by ajfour last updated on 07/Feb/21

$${Find}\:{radius}\:{of}\:{sphere}\:{of}\:{density} \\ $$$$\rho\:{that}\:{receives}\:{maximum} \\ $$$${normal}\:{reaction}\:{from}\:{wall}. \\ $$
Answered by mr W last updated on 08/Feb/21
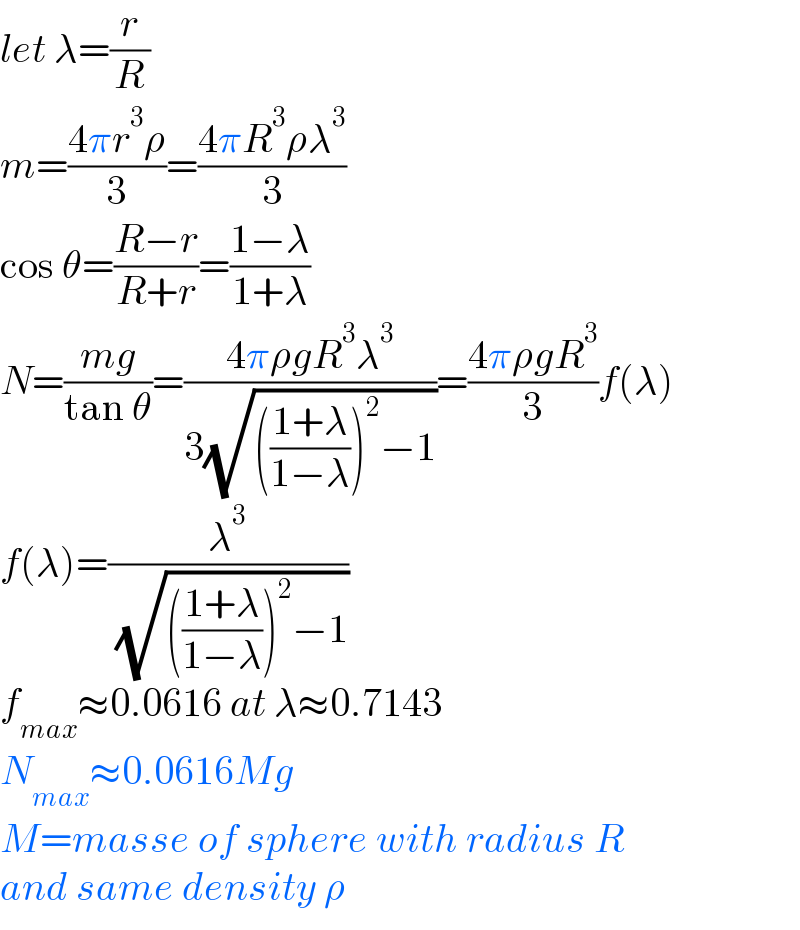
$${let}\:\lambda=\frac{{r}}{{R}} \\ $$$${m}=\frac{\mathrm{4}\pi{r}^{\mathrm{3}} \rho}{\mathrm{3}}=\frac{\mathrm{4}\pi{R}^{\mathrm{3}} \rho\lambda^{\mathrm{3}} }{\mathrm{3}} \\ $$$$\mathrm{cos}\:\theta=\frac{{R}−{r}}{{R}+{r}}=\frac{\mathrm{1}−\lambda}{\mathrm{1}+\lambda} \\ $$$${N}=\frac{{mg}}{\mathrm{tan}\:\theta}=\frac{\mathrm{4}\pi\rho{gR}^{\mathrm{3}} \lambda^{\mathrm{3}} }{\mathrm{3}\sqrt{\left(\frac{\mathrm{1}+\lambda}{\mathrm{1}−\lambda}\right)^{\mathrm{2}} −\mathrm{1}}}=\frac{\mathrm{4}\pi\rho{gR}^{\mathrm{3}} }{\mathrm{3}}{f}\left(\lambda\right) \\ $$$${f}\left(\lambda\right)=\frac{\lambda^{\mathrm{3}} }{\:\sqrt{\left(\frac{\mathrm{1}+\lambda}{\mathrm{1}−\lambda}\right)^{\mathrm{2}} −\mathrm{1}}} \\ $$$${f}_{{max}} \approx\mathrm{0}.\mathrm{0616}\:{at}\:\lambda\approx\mathrm{0}.\mathrm{7143} \\ $$$${N}_{{max}} \approx\mathrm{0}.\mathrm{0616}{Mg} \\ $$$${M}={masse}\:{of}\:{sphere}\:{with}\:{radius}\:{R} \\ $$$${and}\:{same}\:{density}\:\rho \\ $$
Commented by mr W last updated on 08/Feb/21
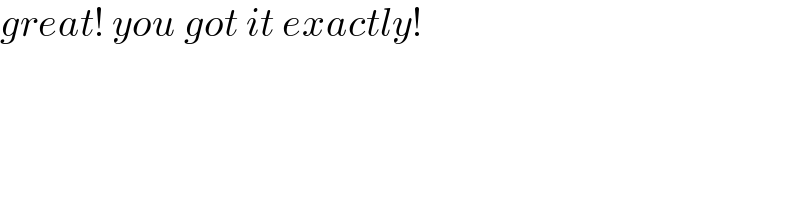
$${great}!\:{you}\:{got}\:{it}\:{exactly}! \\ $$
Commented by ajfour last updated on 08/Feb/21

Commented by ajfour last updated on 08/Feb/21

$$\mathrm{cos}\:\theta=\frac{\mathrm{1}−\lambda}{\mathrm{1}+\lambda} \\ $$$$\lambda=\frac{\mathrm{1}−\mathrm{cos}\:\theta}{\mathrm{1}+\mathrm{cos}\:\theta}=\mathrm{tan}\:^{\mathrm{2}} \frac{\theta}{\mathrm{2}} \\ $$$${N}=\left(\frac{\mathrm{4}}{\mathrm{3}}\pi\rho{R}^{\mathrm{3}} \right){g}\left\{\frac{\mathrm{tan}\:^{\mathrm{6}} \frac{\theta}{\mathrm{2}}\left(\mathrm{1}−\mathrm{tan}\:^{\mathrm{2}} \frac{\theta}{\mathrm{2}}\right)}{\mathrm{2tan}\:\frac{\theta}{\mathrm{2}}}\right\} \\ $$$${say}\:\:\mathrm{tan}\:\frac{\theta}{\mathrm{2}}={x} \\ $$$$\Rightarrow\:{N}=\left(\frac{\mathrm{2}}{\mathrm{3}}\pi\rho{R}^{\mathrm{3}} {g}\right)\left({x}^{\mathrm{5}} −{x}^{\mathrm{7}} \right) \\ $$$$\frac{{dN}}{{d}\left(\mathrm{tan}\:\frac{\theta}{\mathrm{2}}\right)}=\mathrm{0}\:\:\Rightarrow\:\mathrm{5}{x}^{\mathrm{4}} =\mathrm{7}{x}^{\mathrm{6}} \\ $$$${x}^{\mathrm{2}} =\frac{\mathrm{5}}{\mathrm{7}}=\lambda=\frac{{r}}{{R}}\approx\mathrm{0}.\mathrm{7143} \\ $$$${N}_{{max}} =\frac{{Mg}}{\mathrm{2}}\left(\frac{\mathrm{25}}{\mathrm{49}}−\frac{\mathrm{125}}{\mathrm{343}}\right)\sqrt{\frac{\mathrm{5}}{\mathrm{7}}} \\ $$$${N}_{{max}} =\frac{\mathrm{25}\sqrt{\mathrm{5}}}{\mathrm{343}\sqrt{\mathrm{7}}}{Mg} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:=\frac{\mathrm{25}\sqrt{\mathrm{5}}}{\mathrm{343}\sqrt{\mathrm{7}}}\left(\frac{\mathrm{1}}{\lambda^{\mathrm{3}} }\right){mg} \\ $$$${N}_{{max}} =\frac{{mg}}{\:\sqrt{\mathrm{35}}} \\ $$$${N}_{{max}} \approx\mathrm{0}.\mathrm{0616}{Mg}\:\: \\ $$
Commented by ajfour last updated on 08/Feb/21

$${Thank}\:{you}\:{Sir},\:{exact}\:{answer} \\ $$$${isn}'{t}\:{complicated},\:{Sir}! \\ $$
