Question Number 131733 by LYKA last updated on 07/Feb/21

$$\boldsymbol{{find}}\:\boldsymbol{{the}}\:\boldsymbol{{minimum}}\:\boldsymbol{{distance}} \\ $$$$\boldsymbol{{between}}\:\boldsymbol{{the}}\:\boldsymbol{{point}}\:\left(\mathrm{1},\mathrm{1},\mathrm{1}\right)\:{and} \\ $$$$\boldsymbol{{the}}\:\boldsymbol{{plane}}\:\boldsymbol{{x}}+\mathrm{2}\boldsymbol{{y}}+\mathrm{3}\boldsymbol{{z}}=\mathrm{6} \\ $$$$ \\ $$
Answered by physicstutes last updated on 07/Feb/21
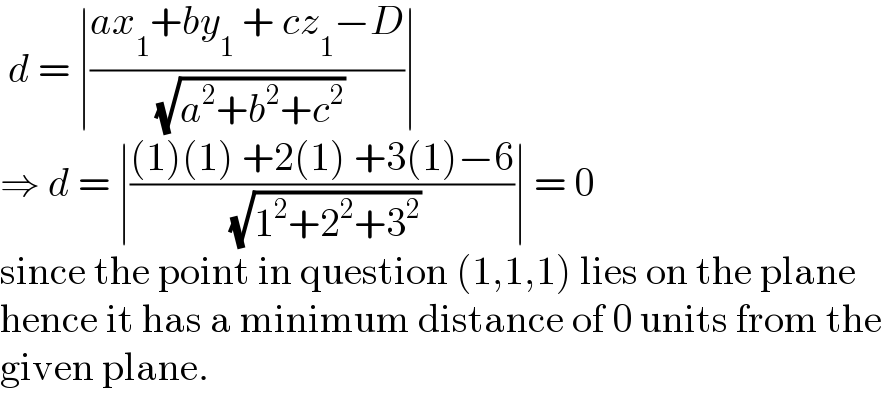
$$\:{d}\:=\:\mid\frac{{ax}_{\mathrm{1}} +{by}_{\mathrm{1}} \:+\:{cz}_{\mathrm{1}} −{D}}{\:\sqrt{{a}^{\mathrm{2}} +{b}^{\mathrm{2}} +{c}^{\mathrm{2}} }}\mid \\ $$$$\Rightarrow\:{d}\:=\:\mid\frac{\left(\mathrm{1}\right)\left(\mathrm{1}\right)\:+\mathrm{2}\left(\mathrm{1}\right)\:+\mathrm{3}\left(\mathrm{1}\right)−\mathrm{6}}{\:\sqrt{\mathrm{1}^{\mathrm{2}} +\mathrm{2}^{\mathrm{2}} +\mathrm{3}^{\mathrm{2}} }}\mid\:=\:\mathrm{0} \\ $$$$\mathrm{since}\:\mathrm{the}\:\mathrm{point}\:\mathrm{in}\:\mathrm{question}\:\left(\mathrm{1},\mathrm{1},\mathrm{1}\right)\:\mathrm{lies}\:\mathrm{on}\:\mathrm{the}\:\mathrm{plane} \\ $$$$\mathrm{hence}\:\mathrm{it}\:\mathrm{has}\:\mathrm{a}\:\mathrm{minimum}\:\mathrm{distance}\:\mathrm{of}\:\mathrm{0}\:\mathrm{units}\:\mathrm{from}\:\mathrm{the}\: \\ $$$$\mathrm{given}\:\mathrm{plane}. \\ $$
