Menu Close

calculate-dx-x-2-1-x-2-2-

Tinku Tara 0 Comments
Pin




Question Number 66309 by mathmax by abdo last updated on 12/Aug/19
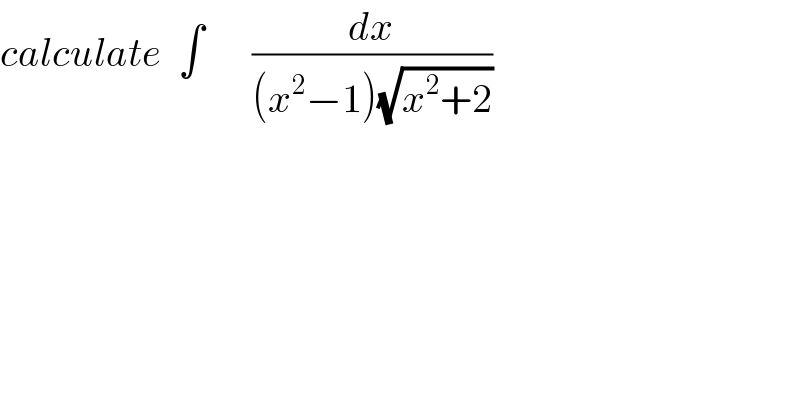
calculate ∫ (dx/((x^2 −1)(√(x^2 +2))))
$${calculate}\:\:\int\:\:\:\:\:\:\frac{{dx}}{\left({x}^{\mathrm{2}} −\mathrm{1}\right)\sqrt{{x}^{\mathrm{2}} +\mathrm{2}}} \\ $$
Commented by prof Abdo imad last updated on 15/Aug/19
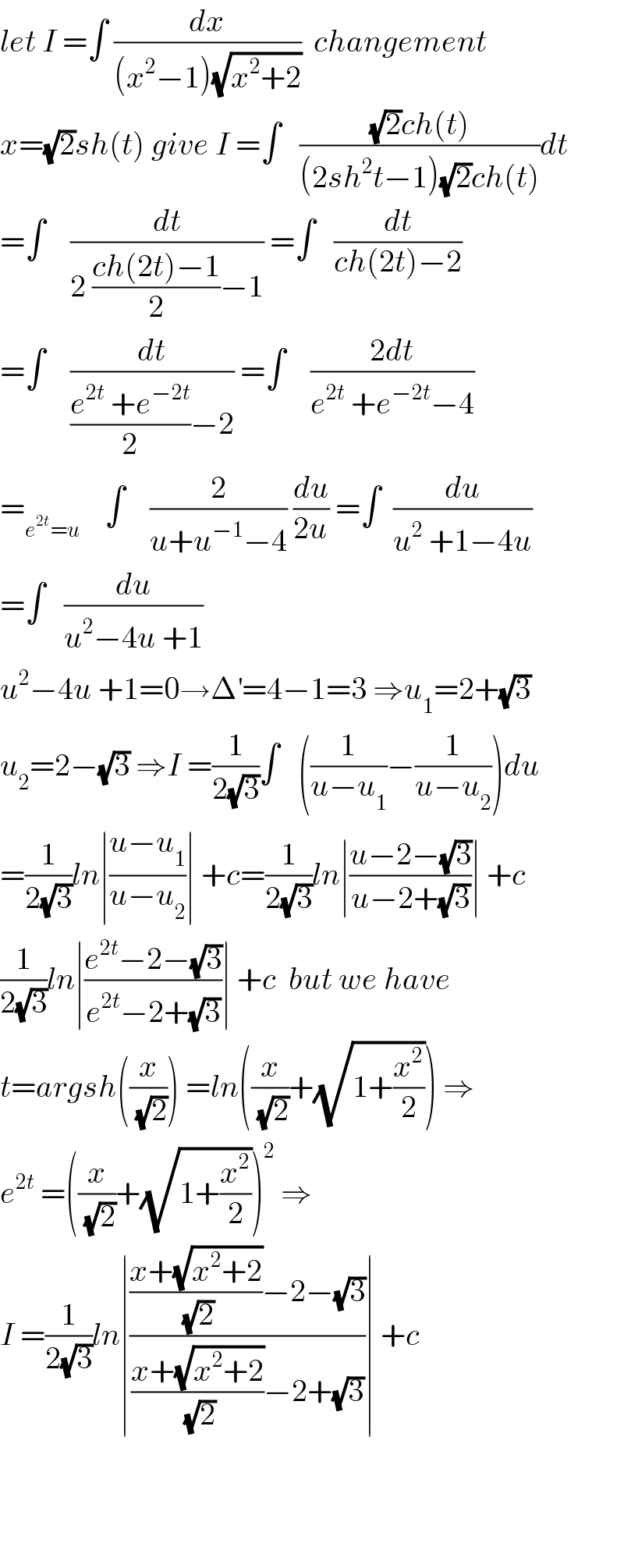
let I =∫ (dx/((x^2 −1)(√(x^2 +2)))) changement x=(√2)sh(t) give I =∫ (((√2)ch(t))/((2sh^2 t−1)(√2)ch(t)))dt =∫ (dt/(2 ((ch(2t)−1)/2)−1)) =∫ (dt/(ch(2t)−2)) =∫ (dt/(((e^(2t) +e^(−2t) )/2)−2)) =∫ ((2dt)/(e^(2t) +e^(−2t) −4)) =_(e^(2t) =u) ∫ (2/(u+u^(−1) −4)) (du/(2u)) =∫ (du/(u^2 +1−4u)) =∫ (du/(u^2 −4u +1)) u^2 −4u +1=0→Δ^′ =4−1=3 ⇒u_1 =2+(√3) u_2 =2−(√3) ⇒I =(1/(2(√3)))∫ ((1/(u−u_1 ))−(1/(u−u_2 )))du =(1/(2(√3)))ln∣((u−u_1 )/(u−u_2 ))∣ +c=(1/(2(√3)))ln∣((u−2−(√3))/(u−2+(√3)))∣ +c (1/(2(√3)))ln∣((e^(2t) −2−(√3))/(e^(2t) −2+(√3)))∣ +c but we have t=argsh((x/( (√2)))) =ln((x/( (√2)))+(√(1+(x^2 /2)))) ⇒ e^(2t) =((x/( (√2)))+(√(1+(x^2 /2))))^2 ⇒ I =(1/(2(√3)))ln∣((((x+(√(x^2 +2)))/( (√2)))−2−(√3))/(((x+(√(x^2 +2)))/( (√2)))−2+(√3)))∣ +c
$${let}\:{I}\:=\int\:\frac{{dx}}{\left({x}^{\mathrm{2}} −\mathrm{1}\right)\sqrt{{x}^{\mathrm{2}} +\mathrm{2}}}\:\:{changement} \\ $$$${x}=\sqrt{\mathrm{2}}{sh}\left({t}\right)\:{give}\:{I}\:=\int\:\:\:\frac{\sqrt{\mathrm{2}}{ch}\left({t}\right)}{\left(\mathrm{2}{sh}^{\mathrm{2}} {t}−\mathrm{1}\right)\sqrt{\mathrm{2}}{ch}\left({t}\right)}{dt} \\ $$$$=\int\:\:\:\:\frac{{dt}}{\mathrm{2}\:\frac{{ch}\left(\mathrm{2}{t}\right)−\mathrm{1}}{\mathrm{2}}−\mathrm{1}}\:=\int\:\:\:\frac{{dt}}{{ch}\left(\mathrm{2}{t}\right)−\mathrm{2}} \\ $$$$=\int\:\:\:\:\frac{{dt}}{\frac{{e}^{\mathrm{2}{t}} \:+{e}^{−\mathrm{2}{t}} }{\mathrm{2}}−\mathrm{2}}\:=\int\:\:\:\:\frac{\mathrm{2}{dt}}{{e}^{\mathrm{2}{t}} \:+{e}^{−\mathrm{2}{t}} −\mathrm{4}} \\ $$$$=_{{e}^{\mathrm{2}{t}} ={u}} \:\:\:\:\int\:\:\:\:\frac{\mathrm{2}}{{u}+{u}^{−\mathrm{1}} −\mathrm{4}}\:\frac{{du}}{\mathrm{2}{u}}\:=\int\:\:\frac{{du}}{{u}^{\mathrm{2}} \:+\mathrm{1}−\mathrm{4}{u}} \\ $$$$=\int\:\:\:\frac{{du}}{{u}^{\mathrm{2}} −\mathrm{4}{u}\:+\mathrm{1}} \\ $$$${u}^{\mathrm{2}} −\mathrm{4}{u}\:+\mathrm{1}=\mathrm{0}\rightarrow\Delta^{'} =\mathrm{4}−\mathrm{1}=\mathrm{3}\:\Rightarrow{u}_{\mathrm{1}} =\mathrm{2}+\sqrt{\mathrm{3}} \\ $$$${u}_{\mathrm{2}} =\mathrm{2}−\sqrt{\mathrm{3}}\:\Rightarrow{I}\:=\frac{\mathrm{1}}{\mathrm{2}\sqrt{\mathrm{3}}}\int\:\:\:\left(\frac{\mathrm{1}}{{u}−{u}_{\mathrm{1}} }−\frac{\mathrm{1}}{{u}−{u}_{\mathrm{2}} }\right){du} \\ $$$$=\frac{\mathrm{1}}{\mathrm{2}\sqrt{\mathrm{3}}}{ln}\mid\frac{{u}−{u}_{\mathrm{1}} }{{u}−{u}_{\mathrm{2}} }\mid\:+{c}=\frac{\mathrm{1}}{\mathrm{2}\sqrt{\mathrm{3}}}{ln}\mid\frac{{u}−\mathrm{2}−\sqrt{\mathrm{3}}}{{u}−\mathrm{2}+\sqrt{\mathrm{3}}}\mid\:+{c} \\ $$$$\frac{\mathrm{1}}{\mathrm{2}\sqrt{\mathrm{3}}}{ln}\mid\frac{{e}^{\mathrm{2}{t}} −\mathrm{2}−\sqrt{\mathrm{3}}}{{e}^{\mathrm{2}{t}} −\mathrm{2}+\sqrt{\mathrm{3}}}\mid\:+{c}\:\:{but}\:{we}\:{have} \\ $$$${t}={argsh}\left(\frac{{x}}{\:\sqrt{\mathrm{2}}}\right)\:={ln}\left(\frac{{x}}{\:\sqrt{\mathrm{2}}}+\sqrt{\mathrm{1}+\frac{{x}^{\mathrm{2}} }{\mathrm{2}}}\right)\:\Rightarrow \\ $$$${e}^{\mathrm{2}{t}} \:=\left(\frac{{x}}{\:\sqrt{\mathrm{2}}}+\sqrt{\mathrm{1}+\frac{{x}^{\mathrm{2}} }{\mathrm{2}}}\right)^{\mathrm{2}} \:\Rightarrow \\ $$$${I}\:=\frac{\mathrm{1}}{\mathrm{2}\sqrt{\mathrm{3}}}{ln}\mid\frac{\frac{{x}+\sqrt{{x}^{\mathrm{2}} +\mathrm{2}}}{\:\sqrt{\mathrm{2}}}−\mathrm{2}−\sqrt{\mathrm{3}}}{\frac{{x}+\sqrt{{x}^{\mathrm{2}} +\mathrm{2}}}{\:\sqrt{\mathrm{2}}}−\mathrm{2}+\sqrt{\mathrm{3}}}\mid\:+{c} \\ $$$$ \\ $$$$ \\ $$$$ \\ $$
Commented by prof Abdo imad last updated on 15/Aug/19
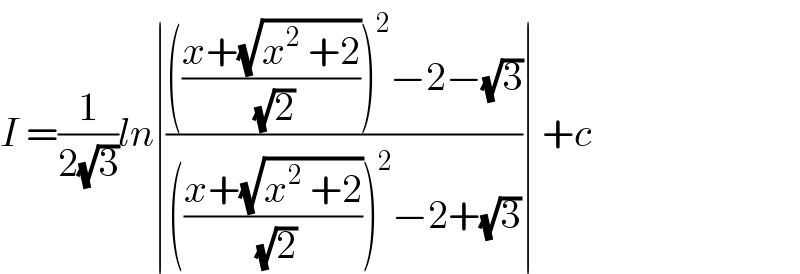
I =(1/(2(√3)))ln∣(((((x+(√(x^2 +2)))/( (√2))))^2 −2−(√3))/((((x+(√(x^2 +2)))/( (√2))))^2 −2+(√3)))∣ +c
$${I}\:=\frac{\mathrm{1}}{\mathrm{2}\sqrt{\mathrm{3}}}{ln}\mid\frac{\left(\frac{{x}+\sqrt{{x}^{\mathrm{2}} \:+\mathrm{2}}}{\:\sqrt{\mathrm{2}}}\right)^{\mathrm{2}} −\mathrm{2}−\sqrt{\mathrm{3}}}{\left(\frac{{x}+\sqrt{{x}^{\mathrm{2}} \:+\mathrm{2}}}{\:\sqrt{\mathrm{2}}}\right)^{\mathrm{2}} −\mathrm{2}+\sqrt{\mathrm{3}}}\mid\:+{c} \\ $$

Pin

Leave a Reply

Your email address will not be published. Required fields are marked *