Menu Close

n-1-1-n-e-2pin-1-

Tinku Tara 0 Comments
Pin




Question Number 131877 by Dwaipayan Shikari last updated on 09/Feb/21
Σ_(n=1) ^∞ (1/(n(e^(2πn) −1)))
$$\underset{{n}=\mathrm{1}} {\overset{\infty} {\sum}}\frac{\mathrm{1}}{{n}\left({e}^{\mathrm{2}\pi{n}} −\mathrm{1}\right)} \\ $$
Commented by Dwaipayan Shikari last updated on 09/Feb/21
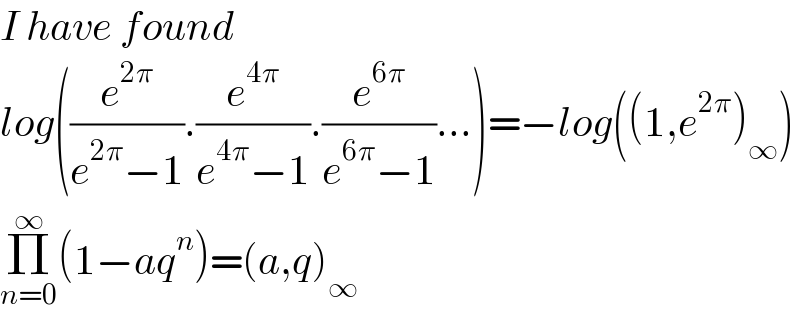
I have found log((e^(2π) /(e^(2π) −1)).(e^(4π) /(e^(4π) −1)).(e^(6π) /(e^(6π) −1))...)=−log((1,e^(2π) )_∞ ) Π_(n=0) ^∞ (1−aq^n )=(a,q)_∞
$${I}\:{have}\:{found}\: \\ $$$${log}\left(\frac{{e}^{\mathrm{2}\pi} }{{e}^{\mathrm{2}\pi} −\mathrm{1}}.\frac{{e}^{\mathrm{4}\pi} }{{e}^{\mathrm{4}\pi} −\mathrm{1}}.\frac{{e}^{\mathrm{6}\pi} }{{e}^{\mathrm{6}\pi} −\mathrm{1}}…\right)=−{log}\left(\left(\mathrm{1},{e}^{\mathrm{2}\pi} \right)_{\infty} \right) \\ $$$$\underset{{n}=\mathrm{0}} {\overset{\infty} {\prod}}\left(\mathrm{1}−{aq}^{{n}} \right)=\left({a},{q}\right)_{\infty} \:\: \\ $$
Commented by SEKRET last updated on 09/Feb/21
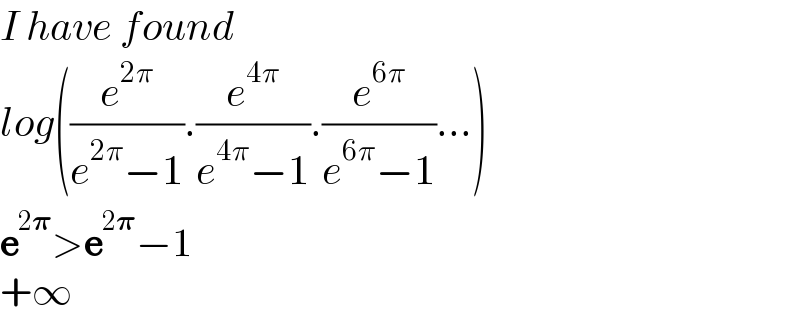
I have found log((e^(2π) /(e^(2π) −1)).(e^(4π) /(e^(4π) −1)).(e^(6π) /(e^(6π) −1))...) e^(2𝛑) >e^(2𝛑) −1 +∞
$${I}\:{have}\:{found}\: \\ $$$${log}\left(\frac{{e}^{\mathrm{2}\pi} }{{e}^{\mathrm{2}\pi} −\mathrm{1}}.\frac{{e}^{\mathrm{4}\pi} }{{e}^{\mathrm{4}\pi} −\mathrm{1}}.\frac{{e}^{\mathrm{6}\pi} }{{e}^{\mathrm{6}\pi} −\mathrm{1}}…\right) \\ $$$$\boldsymbol{\mathrm{e}}^{\mathrm{2}\boldsymbol{\pi}} >\boldsymbol{\mathrm{e}}^{\mathrm{2}\boldsymbol{\pi}} −\mathrm{1}\:\:\:\:\: \\ $$$$+\infty \\ $$
Commented by Dwaipayan Shikari last updated on 09/Feb/21
But not significantly (e^(2π) /(e^(2π) −1))=1.0018.. (e^(4π) /(e^(4π) −1))<1.0018 (e^(6π) /(e^(6π) −1))<(e^(4π) /(e^(4π) −1))<1.0018 So (e^(2π) /(e^(2π) −1)).(e^(4π) /(e^(4π) −1)).(e^(6π) /(e^(6π) −1))...=(1.0018)(1.0018−ε)(1.00018−2ε)... So it converges ε=+ve
$${But}\:{not}\:{significantly}\:\frac{{e}^{\mathrm{2}\pi} }{{e}^{\mathrm{2}\pi} −\mathrm{1}}=\mathrm{1}.\mathrm{0018}.. \\ $$$$\frac{{e}^{\mathrm{4}\pi} }{{e}^{\mathrm{4}\pi} −\mathrm{1}}<\mathrm{1}.\mathrm{0018} \\ $$$$\frac{{e}^{\mathrm{6}\pi} }{{e}^{\mathrm{6}\pi} −\mathrm{1}}<\frac{{e}^{\mathrm{4}\pi} }{{e}^{\mathrm{4}\pi} −\mathrm{1}}<\mathrm{1}.\mathrm{0018} \\ $$$${So}\:\frac{{e}^{\mathrm{2}\pi} }{{e}^{\mathrm{2}\pi} −\mathrm{1}}.\frac{{e}^{\mathrm{4}\pi} }{{e}^{\mathrm{4}\pi} −\mathrm{1}}.\frac{{e}^{\mathrm{6}\pi} }{{e}^{\mathrm{6}\pi} −\mathrm{1}}…=\left(\mathrm{1}.\mathrm{0018}\right)\left(\mathrm{1}.\mathrm{0018}−\epsilon\right)\left(\mathrm{1}.\mathrm{00018}−\mathrm{2}\epsilon\right)… \\ $$$${So}\:{it}\:{converges}\:\:\:\epsilon=+{ve} \\ $$
Commented by Dwaipayan Shikari last updated on 09/Feb/21
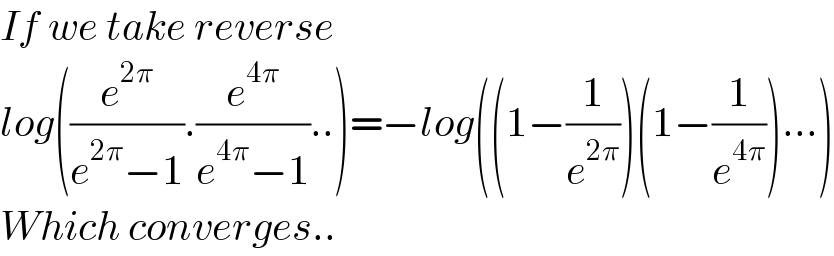
If we take reverse log((e^(2π) /(e^(2π) −1)).(e^(4π) /(e^(4π) −1))..)=−log((1−(1/e^(2π) ))(1−(1/e^(4π) ))...) Which converges..
$${If}\:{we}\:{take}\:{reverse} \\ $$$${log}\left(\frac{{e}^{\mathrm{2}\pi} }{{e}^{\mathrm{2}\pi} −\mathrm{1}}.\frac{{e}^{\mathrm{4}\pi} }{{e}^{\mathrm{4}\pi} −\mathrm{1}}..\right)=−{log}\left(\left(\mathrm{1}−\frac{\mathrm{1}}{{e}^{\mathrm{2}\pi} }\right)\left(\mathrm{1}−\frac{\mathrm{1}}{{e}^{\mathrm{4}\pi} }\right)…\right) \\ $$$${Which}\:{converges}.. \\ $$

Pin

Leave a Reply

Your email address will not be published. Required fields are marked *