Evaluate-sinx-x-2-2x-2-dx-

Question Number 131957 by rs4089 last updated on 10/Feb/21

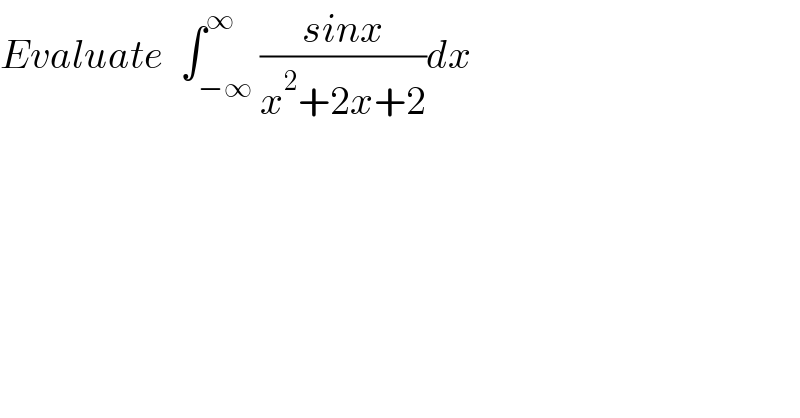
$${Evaluate}\:\:\int_{−\infty} ^{\infty} \frac{{sinx}}{{x}^{\mathrm{2}} +\mathrm{2}{x}+\mathrm{2}}{dx} \\ $$

Answered by Dwaipayan Shikari last updated on 10/Feb/21

∫_(−∞) ^∞ ((sinx)/((x+1)^2 +1)) x+1=u =∫_(−∞) ^∞ ((sinu cos1−sin1cosu)/(u^2 +1))du =cos1∫_(−∞) ^∞ ((sinu)/(u^2 +1))−sin1∫_(−∞) ^∞ ((cosu)/(u^2 +1))du =0−(π/e)sin(1)=−(π/e)sin(1)

$$\int_{−\infty} ^{\infty} \frac{{sinx}}{\left({x}+\mathrm{1}\right)^{\mathrm{2}} +\mathrm{1}}\:\:\:\:\:\:\:{x}+\mathrm{1}={u} \\ $$$$=\int_{−\infty} ^{\infty} \frac{{sinu}\:{cos}\mathrm{1}−{sin}\mathrm{1}{cosu}}{{u}^{\mathrm{2}} +\mathrm{1}}{du} \\ $$$$={cos}\mathrm{1}\int_{−\infty} ^{\infty} \frac{{sinu}}{{u}^{\mathrm{2}} +\mathrm{1}}−{sin}\mathrm{1}\int_{−\infty} ^{\infty} \frac{{cosu}}{{u}^{\mathrm{2}} +\mathrm{1}}{du} \\ $$$$=\mathrm{0}−\frac{\pi}{{e}}{sin}\left(\mathrm{1}\right)=−\frac{\pi}{{e}}{sin}\left(\mathrm{1}\right) \\ $$

Commented by mnjuly1970 last updated on 10/Feb/21

$${very}\:{nice}\:{mr}\:{payan}… \\ $$

Answered by mathmax by abdo last updated on 10/Feb/21

let f(λ)=∫_(−∞) ^(+∞) ((sin(λx))/(x^2 +2x+2))dx withλ>0 ⇒f(λ)=Im(∫_(−∞) ^(+∞) (e^(iλx) /(x^2 +2x+2))dx) Φ(z)=(e^(iλz) /(z^2 +2z+2)) we have z^2 +2z+2=0⇒(z+1)^2 +1=0 ⇒ (z+1)^2 =−1 ⇒z+1=+^− i ⇒z =−1+^− i si the poles of Φ are z_1 =−1+i and z_2 =−1−i ∫_R Φ(z)dz =2iπRes(Φ,z_1 )=2iπ .(e^(iλz_1 ) /(2i)) =π e^(iλ(−1+i)) =π e^(−iλ−λ) =πe^(−λ) {cos(λ)−isin(λ)} ⇒f(λ)=−πe^(−λ) sin(λ) ∫_(−∞) ^(+∞) ((sinx)/(x^2 +2x+2))dx =f(1)=−(π/e)sin(1)

$$\mathrm{let}\:\mathrm{f}\left(\lambda\right)=\int_{−\infty} ^{+\infty} \:\frac{\mathrm{sin}\left(\lambda\mathrm{x}\right)}{\mathrm{x}^{\mathrm{2}} \:+\mathrm{2x}+\mathrm{2}}\mathrm{dx}\:\mathrm{with}\lambda>\mathrm{0}\:\Rightarrow\mathrm{f}\left(\lambda\right)=\mathrm{Im}\left(\int_{−\infty} ^{+\infty} \:\frac{\mathrm{e}^{\mathrm{i}\lambda\mathrm{x}} }{\mathrm{x}^{\mathrm{2}} \:+\mathrm{2x}+\mathrm{2}}\mathrm{dx}\right) \\ $$$$\Phi\left(\mathrm{z}\right)=\frac{\mathrm{e}^{\mathrm{i}\lambda\mathrm{z}} }{\mathrm{z}^{\mathrm{2}} \:+\mathrm{2z}+\mathrm{2}}\:\:\mathrm{we}\:\mathrm{have}\:\mathrm{z}^{\mathrm{2}} \:+\mathrm{2z}+\mathrm{2}=\mathrm{0}\Rightarrow\left(\mathrm{z}+\mathrm{1}\right)^{\mathrm{2}} +\mathrm{1}=\mathrm{0}\:\Rightarrow \\ $$$$\left(\mathrm{z}+\mathrm{1}\right)^{\mathrm{2}} =−\mathrm{1}\:\Rightarrow\mathrm{z}+\mathrm{1}=\overset{−} {+}\mathrm{i}\:\Rightarrow\mathrm{z}\:=−\mathrm{1}\overset{−} {+}\mathrm{i}\:\mathrm{si}\:\mathrm{the}\:\mathrm{poles}\:\mathrm{of}\:\Phi\:\mathrm{are}\: \\ $$$$\mathrm{z}_{\mathrm{1}} =−\mathrm{1}+\mathrm{i}\:\mathrm{and}\:\mathrm{z}_{\mathrm{2}} =−\mathrm{1}−\mathrm{i} \\ $$$$\int_{\mathrm{R}} \Phi\left(\mathrm{z}\right)\mathrm{dz}\:=\mathrm{2i}\pi\mathrm{Res}\left(\Phi,\mathrm{z}_{\mathrm{1}} \right)=\mathrm{2i}\pi\:.\frac{\mathrm{e}^{\mathrm{i}\lambda\mathrm{z}_{\mathrm{1}} } }{\mathrm{2i}}\:=\pi\:\mathrm{e}^{\mathrm{i}\lambda\left(−\mathrm{1}+\mathrm{i}\right)} \\ $$$$=\pi\:\mathrm{e}^{−\mathrm{i}\lambda−\lambda} \:=\pi\mathrm{e}^{−\lambda} \left\{\mathrm{cos}\left(\lambda\right)−\mathrm{isin}\left(\lambda\right)\right\}\:\Rightarrow\mathrm{f}\left(\lambda\right)=−\pi\mathrm{e}^{−\lambda} \:\mathrm{sin}\left(\lambda\right) \\ $$$$\int_{−\infty} ^{+\infty} \:\frac{\mathrm{sinx}}{\mathrm{x}^{\mathrm{2}} \:+\mathrm{2x}+\mathrm{2}}\mathrm{dx}\:=\mathrm{f}\left(\mathrm{1}\right)=−\frac{\pi}{\mathrm{e}}\mathrm{sin}\left(\mathrm{1}\right) \\ $$

Facebook Tweet Pin

Evaluate-sinx-x-2-2x-2-dx-

Leave a Reply Cancel reply