Menu Close

how-that-tan2x-2tanx-1-tan-2-x-

Tinku Tara 0 Comments
Pin




Question Number 76495 by mathocean1 last updated on 27/Dec/19
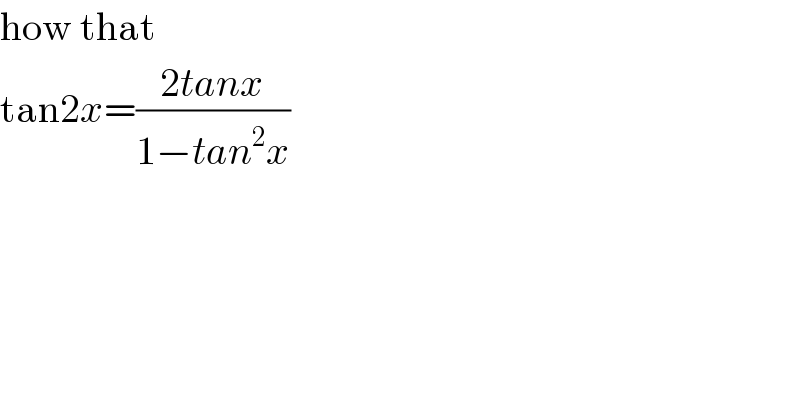
how that tan2x=((2tanx)/(1−tan^2 x))
$$\mathrm{how}\:\mathrm{that} \\ $$$$\mathrm{tan2}{x}=\frac{\mathrm{2}{tanx}}{\mathrm{1}−{tan}^{\mathrm{2}} {x}} \\ $$
Commented by mathmax by abdo last updated on 27/Dec/19
due to formula tan(2x)=tan(x+x) =((tanx+tanx)/(1−tanx.tanx)) =((2tanx)/(1−tan^2 x))
$${due}\:{to}\:{formula}\:\:{tan}\left(\mathrm{2}{x}\right)={tan}\left({x}+{x}\right)\:=\frac{{tanx}+{tanx}}{\mathrm{1}−{tanx}.{tanx}} \\ $$$$=\frac{\mathrm{2}{tanx}}{\mathrm{1}−{tan}^{\mathrm{2}} {x}} \\ $$
Commented by mathocean1 last updated on 27/Dec/19
thank you sir
$$\mathrm{thank}\:\mathrm{you}\:\mathrm{sir} \\ $$
Commented by mathmax by abdo last updated on 27/Dec/19
you are welcome.
$${you}\:{are}\:{welcome}. \\ $$
Commented by Kunal12588 last updated on 28/Dec/19
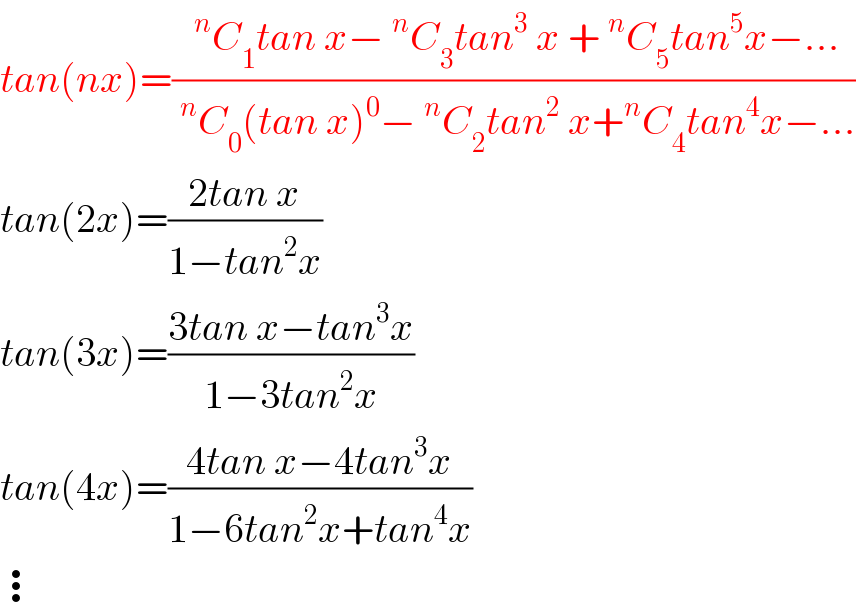
tan(nx)=((^n C_1 tan x−^n C_3 tan^3 x +^n C_5 tan^5 x−...)/(^n C_0 (tan x)^0 −^n C_2 tan^2 x+^n C_4 tan^4 x−...)) tan(2x)=((2tan x)/(1−tan^2 x)) tan(3x)=((3tan x−tan^3 x)/(1−3tan^2 x)) tan(4x)=((4tan x−4tan^3 x)/(1−6tan^2 x+tan^4 x)) ⋮
$${tan}\left({nx}\right)=\frac{\:^{{n}} {C}_{\mathrm{1}} {tan}\:{x}−\:^{{n}} {C}_{\mathrm{3}} {tan}^{\mathrm{3}} \:{x}\:+\:^{{n}} {C}_{\mathrm{5}} {tan}^{\mathrm{5}} {x}−…}{\:^{{n}} {C}_{\mathrm{0}} \left({tan}\:{x}\right)^{\mathrm{0}} −\:^{{n}} {C}_{\mathrm{2}} {tan}^{\mathrm{2}} \:{x}+^{{n}} {C}_{\mathrm{4}} {tan}^{\mathrm{4}} {x}−…} \\ $$$${tan}\left(\mathrm{2}{x}\right)=\frac{\mathrm{2}{tan}\:{x}}{\mathrm{1}−{tan}^{\mathrm{2}} {x}} \\ $$$${tan}\left(\mathrm{3}{x}\right)=\frac{\mathrm{3}{tan}\:{x}−{tan}^{\mathrm{3}} {x}}{\mathrm{1}−\mathrm{3}{tan}^{\mathrm{2}} {x}} \\ $$$${tan}\left(\mathrm{4}{x}\right)=\frac{\mathrm{4}{tan}\:{x}−\mathrm{4}{tan}^{\mathrm{3}} {x}}{\mathrm{1}−\mathrm{6}{tan}^{\mathrm{2}} {x}+{tan}^{\mathrm{4}} {x}} \\ $$$$\vdots \\ $$
Commented by john santu last updated on 28/Dec/19
wawww great sir short cut.
$${wawww}\:\:\:\:{great}\:{sir}\:{short}\:{cut}. \\ $$
Answered by $@ty@m123 last updated on 28/Dec/19
Alternative proof: tan 2x=((sin 2x)/(cos 2x)) =((2sin xcos x)/(cos^2 x−sin^2 x)) =(((2sin xcos x)÷cos^2 x)/((cos^2 x−sin^2 x)÷cos^2 x)) =((2tan x)/(1−tan^2 x))
$${Alternative}\:{proof}: \\ $$$$\mathrm{tan}\:\mathrm{2}{x}=\frac{\mathrm{sin}\:\mathrm{2}{x}}{\mathrm{cos}\:\mathrm{2}{x}} \\ $$$$=\frac{\mathrm{2sin}\:{x}\mathrm{cos}\:{x}}{\mathrm{cos}\:^{\mathrm{2}} {x}−\mathrm{sin}\:^{\mathrm{2}} {x}} \\ $$$$=\frac{\left(\mathrm{2sin}\:{x}\mathrm{cos}\:{x}\right)\boldsymbol{\div}\mathrm{cos}\:^{\mathrm{2}} {x}}{\left(\mathrm{cos}\:^{\mathrm{2}} {x}−\mathrm{sin}\:^{\mathrm{2}} {x}\right)\boldsymbol{\div}\mathrm{cos}\:^{\mathrm{2}} {x}} \\ $$$$=\frac{\mathrm{2tan}\:{x}}{\mathrm{1}−\mathrm{tan}\:^{\mathrm{2}} {x}} \\ $$

Pin

Leave a Reply

Your email address will not be published. Required fields are marked *