Question Number 10987 by Joel576 last updated on 05/Mar/17
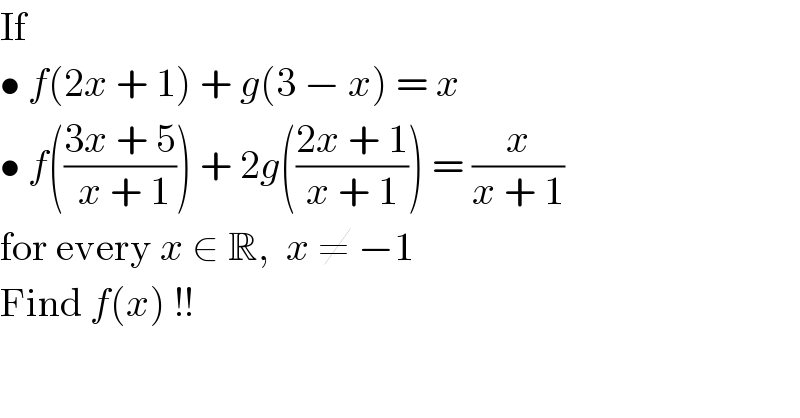
$$\mathrm{If}\: \\ $$$$\bullet\:{f}\left(\mathrm{2}{x}\:+\:\mathrm{1}\right)\:+\:{g}\left(\mathrm{3}\:−\:{x}\right)\:=\:{x} \\ $$$$\bullet\:{f}\left(\frac{\mathrm{3}{x}\:+\:\mathrm{5}}{\:{x}\:+\:\mathrm{1}}\right)\:+\:\mathrm{2}{g}\left(\frac{\mathrm{2}{x}\:+\:\mathrm{1}}{{x}\:+\:\mathrm{1}}\right)\:=\:\frac{{x}}{{x}\:+\:\mathrm{1}} \\ $$$$\mathrm{for}\:\mathrm{every}\:{x}\:\in\:\mathbb{R},\:\:{x}\:\neq\:−\mathrm{1} \\ $$$$\mathrm{Find}\:{f}\left({x}\right)\:!! \\ $$
Answered by ajfour last updated on 05/Mar/17

$$\frac{\left(\mathrm{3}{x}−\mathrm{7}\right)}{\mathrm{2}}\:\:\:{is}\:{it}?! \\ $$
Commented by ajfour last updated on 05/Mar/17
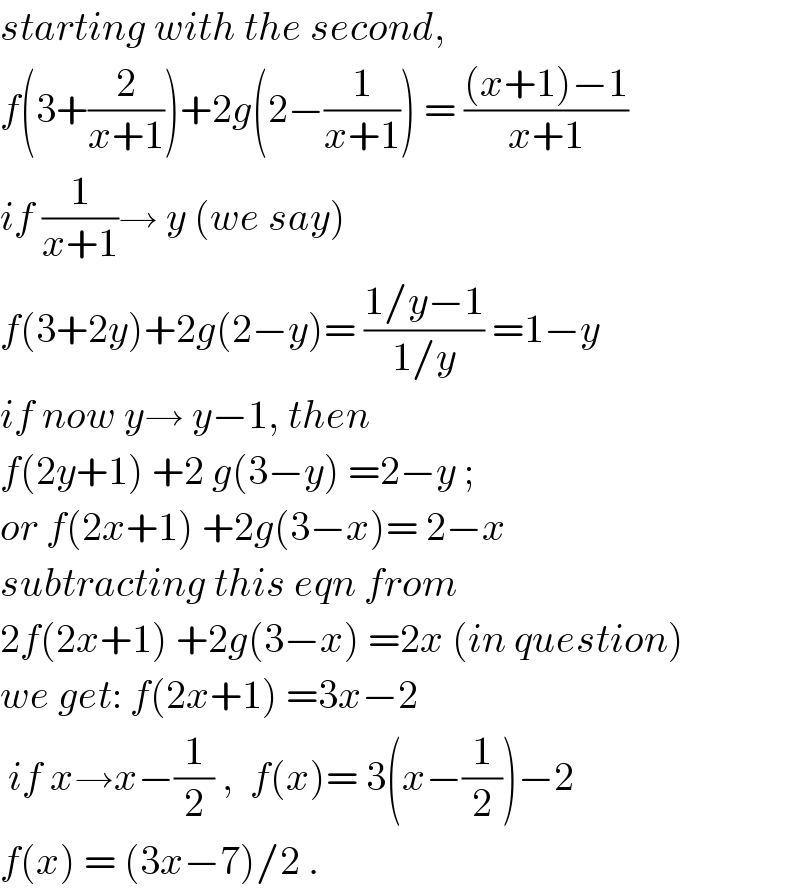
$${starting}\:{with}\:{the}\:{second},\: \\ $$$${f}\left(\mathrm{3}+\frac{\mathrm{2}}{{x}+\mathrm{1}}\right)+\mathrm{2}{g}\left(\mathrm{2}−\frac{\mathrm{1}}{{x}+\mathrm{1}}\right)\:=\:\frac{\left({x}+\mathrm{1}\right)−\mathrm{1}}{{x}+\mathrm{1}} \\ $$$${if}\:\frac{\mathrm{1}}{{x}+\mathrm{1}}\rightarrow\:{y}\:\left({we}\:{say}\right) \\ $$$${f}\left(\mathrm{3}+\mathrm{2}{y}\right)+\mathrm{2}{g}\left(\mathrm{2}−{y}\right)=\:\frac{\mathrm{1}/{y}−\mathrm{1}}{\mathrm{1}/{y}}\:=\mathrm{1}−{y} \\ $$$${if}\:{now}\:{y}\rightarrow\:{y}−\mathrm{1},\:{then} \\ $$$${f}\left(\mathrm{2}{y}+\mathrm{1}\right)\:+\mathrm{2}\:{g}\left(\mathrm{3}−{y}\right)\:=\mathrm{2}−{y}\:; \\ $$$${or}\:{f}\left(\mathrm{2}{x}+\mathrm{1}\right)\:+\mathrm{2}{g}\left(\mathrm{3}−{x}\right)=\:\mathrm{2}−{x} \\ $$$${subtracting}\:{this}\:{eqn}\:{from} \\ $$$$\mathrm{2}{f}\left(\mathrm{2}{x}+\mathrm{1}\right)\:+\mathrm{2}{g}\left(\mathrm{3}−{x}\right)\:=\mathrm{2}{x}\:\left({in}\:{question}\right) \\ $$$${we}\:{get}:\:{f}\left(\mathrm{2}{x}+\mathrm{1}\right)\:=\mathrm{3}{x}−\mathrm{2} \\ $$$$\:{if}\:{x}\rightarrow{x}−\frac{\mathrm{1}}{\mathrm{2}}\:,\:\:{f}\left({x}\right)=\:\mathrm{3}\left({x}−\frac{\mathrm{1}}{\mathrm{2}}\right)−\mathrm{2} \\ $$$${f}\left({x}\right)\:=\:\left(\mathrm{3}{x}−\mathrm{7}\right)/\mathrm{2}\:. \\ $$
Commented by Joel576 last updated on 08/Mar/17

$${thank}\:{you}\:{very}\:{much} \\ $$
