Question Number 142115 by mathmax by abdo last updated on 26/May/21
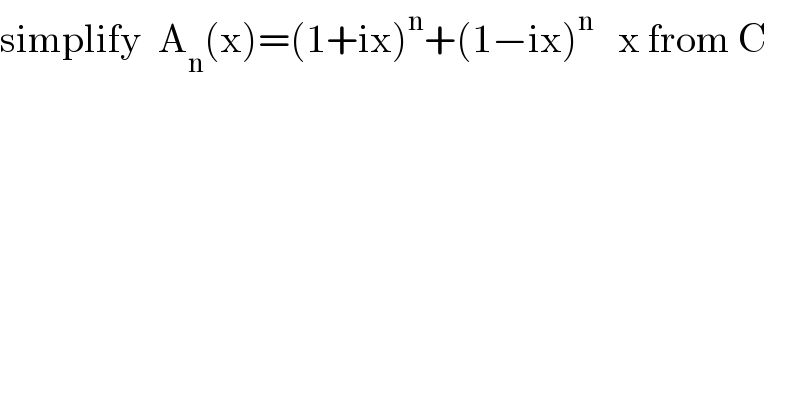
$$\mathrm{simplify}\:\:\mathrm{A}_{\mathrm{n}} \left(\mathrm{x}\right)=\left(\mathrm{1}+\mathrm{ix}\right)^{\mathrm{n}} +\left(\mathrm{1}−\mathrm{ix}\right)^{\mathrm{n}} \:\:\:\mathrm{x}\:\mathrm{from}\:\mathrm{C} \\ $$
Answered by mathmax by abdo last updated on 27/May/21
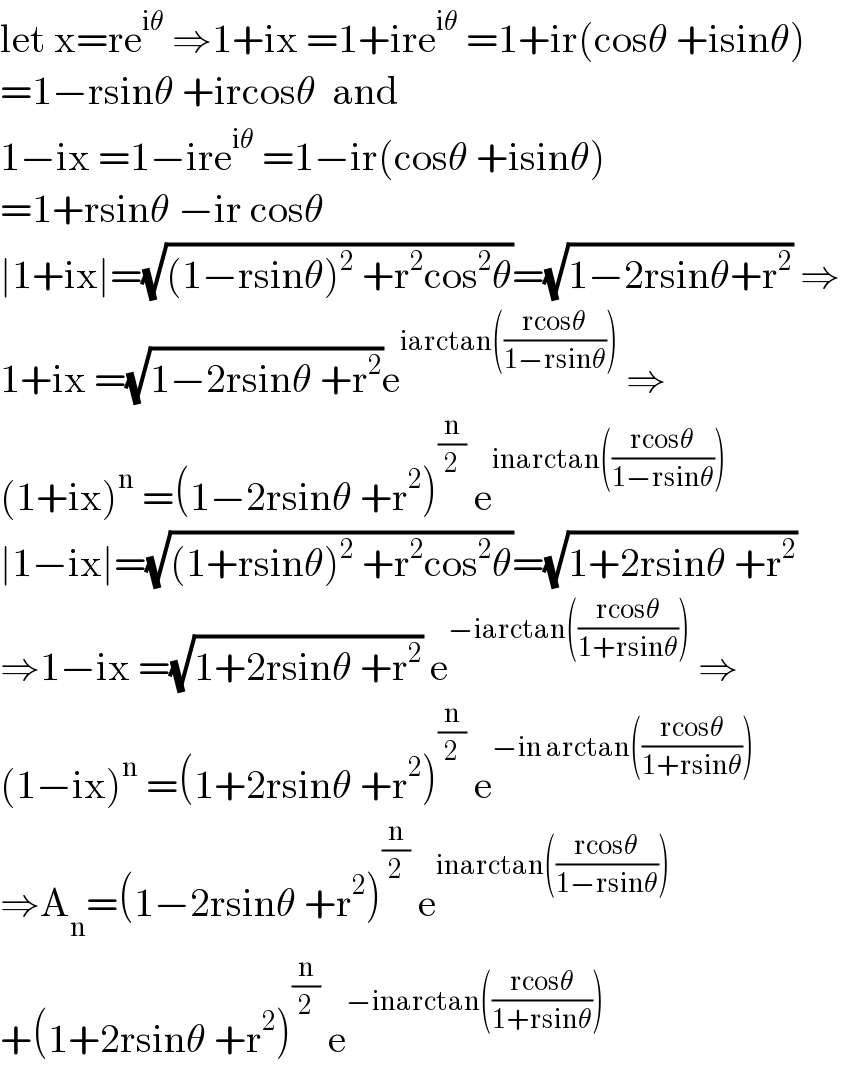
$$\mathrm{let}\:\mathrm{x}=\mathrm{re}^{\mathrm{i}\theta} \:\Rightarrow\mathrm{1}+\mathrm{ix}\:=\mathrm{1}+\mathrm{ire}^{\mathrm{i}\theta} \:=\mathrm{1}+\mathrm{ir}\left(\mathrm{cos}\theta\:+\mathrm{isin}\theta\right) \\ $$$$=\mathrm{1}−\mathrm{rsin}\theta\:+\mathrm{ircos}\theta\:\:\mathrm{and} \\ $$$$\mathrm{1}−\mathrm{ix}\:=\mathrm{1}−\mathrm{ire}^{\mathrm{i}\theta} \:=\mathrm{1}−\mathrm{ir}\left(\mathrm{cos}\theta\:+\mathrm{isin}\theta\right) \\ $$$$=\mathrm{1}+\mathrm{rsin}\theta\:−\mathrm{ir}\:\mathrm{cos}\theta \\ $$$$\mid\mathrm{1}+\mathrm{ix}\mid=\sqrt{\left(\mathrm{1}−\mathrm{rsin}\theta\right)^{\mathrm{2}} \:+\mathrm{r}^{\mathrm{2}} \mathrm{cos}^{\mathrm{2}} \theta}=\sqrt{\mathrm{1}−\mathrm{2rsin}\theta+\mathrm{r}^{\mathrm{2}} }\:\Rightarrow \\ $$$$\mathrm{1}+\mathrm{ix}\:=\sqrt{\mathrm{1}−\mathrm{2rsin}\theta\:+\mathrm{r}^{\mathrm{2}} }\mathrm{e}^{\mathrm{iarctan}\left(\frac{\mathrm{rcos}\theta}{\mathrm{1}−\mathrm{rsin}\theta}\right)} \:\Rightarrow \\ $$$$\left(\mathrm{1}+\mathrm{ix}\right)^{\mathrm{n}} \:=\left(\mathrm{1}−\mathrm{2rsin}\theta\:+\mathrm{r}^{\mathrm{2}} \right)^{\frac{\mathrm{n}}{\mathrm{2}}} \:\mathrm{e}^{\mathrm{inarctan}\left(\frac{\mathrm{rcos}\theta}{\mathrm{1}−\mathrm{rsin}\theta}\right)} \\ $$$$\mid\mathrm{1}−\mathrm{ix}\mid=\sqrt{\left(\mathrm{1}+\mathrm{rsin}\theta\right)^{\mathrm{2}} \:+\mathrm{r}^{\mathrm{2}} \mathrm{cos}^{\mathrm{2}} \theta}=\sqrt{\mathrm{1}+\mathrm{2rsin}\theta\:+\mathrm{r}^{\mathrm{2}} } \\ $$$$\Rightarrow\mathrm{1}−\mathrm{ix}\:=\sqrt{\mathrm{1}+\mathrm{2rsin}\theta\:+\mathrm{r}^{\mathrm{2}} }\:\mathrm{e}^{−\mathrm{iarctan}\left(\frac{\mathrm{rcos}\theta}{\mathrm{1}+\mathrm{rsin}\theta}\right)} \:\Rightarrow \\ $$$$\left(\mathrm{1}−\mathrm{ix}\right)^{\mathrm{n}} \:=\left(\mathrm{1}+\mathrm{2rsin}\theta\:+\mathrm{r}^{\mathrm{2}} \right)^{\frac{\mathrm{n}}{\mathrm{2}}} \:\mathrm{e}^{−\mathrm{in}\:\mathrm{arctan}\left(\frac{\mathrm{rcos}\theta}{\mathrm{1}+\mathrm{rsin}\theta}\right)} \\ $$$$\Rightarrow\mathrm{A}_{\mathrm{n}} =\left(\mathrm{1}−\mathrm{2rsin}\theta\:+\mathrm{r}^{\mathrm{2}} \right)^{\frac{\mathrm{n}}{\mathrm{2}}} \:\mathrm{e}^{\mathrm{inarctan}\left(\frac{\mathrm{rcos}\theta}{\mathrm{1}−\mathrm{rsin}\theta}\right)} \\ $$$$+\left(\mathrm{1}+\mathrm{2rsin}\theta\:+\mathrm{r}^{\mathrm{2}} \right)^{\frac{\mathrm{n}}{\mathrm{2}}} \:\mathrm{e}^{−\mathrm{inarctan}\left(\frac{\mathrm{rcos}\theta}{\mathrm{1}+\mathrm{rsin}\theta}\right)} \\ $$
Answered by mathmax by abdo last updated on 27/May/21
![another way A_n (x)=Σ_(k=0) ^n C_n ^k (ix)^k +Σ_(k=0) ^n C_n ^k (−ix)^k =Σ_(k=0) ^n C_n ^k (i^k +(−i)^k )x^k = Σ_(p=0) ^([(n/2)]) C_n ^(2p) 2(−1)^p x^(2p) =2 Σ_(p=0) ^([(n/2)]) C_n ^(2p) (−1)^p x^(2p)](https://www.tinkutara.com/question/Q142161.png)
$$\mathrm{another}\:\mathrm{way} \\ $$$$\mathrm{A}_{\mathrm{n}} \left(\mathrm{x}\right)=\sum_{\mathrm{k}=\mathrm{0}} ^{\mathrm{n}} \mathrm{C}_{\mathrm{n}} ^{\mathrm{k}} \left(\mathrm{ix}\right)^{\mathrm{k}} \:+\sum_{\mathrm{k}=\mathrm{0}} ^{\mathrm{n}} \:\mathrm{C}_{\mathrm{n}} ^{\mathrm{k}} \left(−\mathrm{ix}\right)^{\mathrm{k}} \\ $$$$=\sum_{\mathrm{k}=\mathrm{0}} ^{\mathrm{n}} \:\mathrm{C}_{\mathrm{n}} ^{\mathrm{k}} \left(\mathrm{i}^{\mathrm{k}} \:+\left(−\mathrm{i}\right)^{\mathrm{k}} \right)\mathrm{x}^{\mathrm{k}} \\ $$$$=\:\sum_{\mathrm{p}=\mathrm{0}} ^{\left[\frac{\mathrm{n}}{\mathrm{2}}\right]} \:\mathrm{C}_{\mathrm{n}} ^{\mathrm{2p}} \:\mathrm{2}\left(−\mathrm{1}\right)^{\mathrm{p}} \:\mathrm{x}^{\mathrm{2p}} \:\:=\mathrm{2}\:\sum_{\mathrm{p}=\mathrm{0}} ^{\left[\frac{\mathrm{n}}{\mathrm{2}}\right]} \:\mathrm{C}_{\mathrm{n}} ^{\mathrm{2p}} \left(−\mathrm{1}\right)^{\mathrm{p}} \:\mathrm{x}^{\mathrm{2p}} \\ $$
