Question Number 142120 by bekzodjumayev last updated on 26/May/21
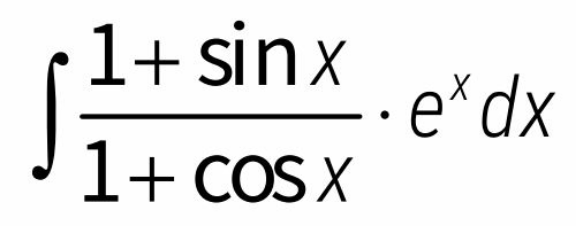
Commented by bekzodjumayev last updated on 26/May/21

$${Help} \\ $$
Commented by Avijit007 last updated on 26/May/21

$${got}\:{it} \\ $$
Answered by som(math1967) last updated on 26/May/21
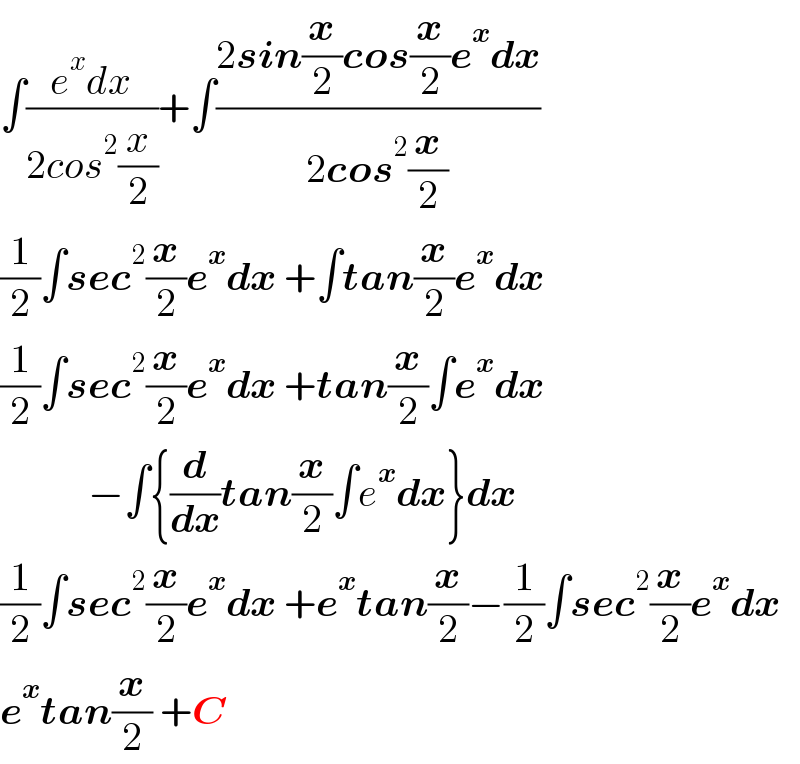
$$\int\frac{{e}^{{x}} {dx}}{\mathrm{2}{cos}^{\mathrm{2}} \frac{{x}}{\mathrm{2}}}+\int\frac{\mathrm{2}\boldsymbol{{sin}}\frac{\boldsymbol{{x}}}{\mathrm{2}}\boldsymbol{{cos}}\frac{\boldsymbol{{x}}}{\mathrm{2}}\boldsymbol{{e}}^{\boldsymbol{{x}}} \boldsymbol{{dx}}}{\mathrm{2}\boldsymbol{{cos}}^{\mathrm{2}} \frac{\boldsymbol{{x}}}{\mathrm{2}}} \\ $$$$\frac{\mathrm{1}}{\mathrm{2}}\int\boldsymbol{{sec}}^{\mathrm{2}} \frac{\boldsymbol{{x}}}{\mathrm{2}}\boldsymbol{{e}}^{\boldsymbol{{x}}} \boldsymbol{{dx}}\:+\int\boldsymbol{{tan}}\frac{\boldsymbol{{x}}}{\mathrm{2}}\boldsymbol{{e}}^{\boldsymbol{{x}}} \boldsymbol{{dx}} \\ $$$$\frac{\mathrm{1}}{\mathrm{2}}\int\boldsymbol{{sec}}^{\mathrm{2}} \frac{\boldsymbol{{x}}}{\mathrm{2}}\boldsymbol{{e}}^{\boldsymbol{{x}}} \boldsymbol{{dx}}\:+\boldsymbol{{tan}}\frac{\boldsymbol{{x}}}{\mathrm{2}}\int\boldsymbol{{e}}^{\boldsymbol{{x}}} \boldsymbol{{dx}} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:−\int\left\{\frac{\boldsymbol{{d}}}{\boldsymbol{{dx}}}\boldsymbol{{tan}}\frac{\boldsymbol{{x}}}{\mathrm{2}}\int{e}^{\boldsymbol{{x}}} \boldsymbol{{dx}}\right\}\boldsymbol{{dx}} \\ $$$$\frac{\mathrm{1}}{\mathrm{2}}\int\boldsymbol{{sec}}^{\mathrm{2}} \frac{\boldsymbol{{x}}}{\mathrm{2}}\boldsymbol{{e}}^{\boldsymbol{{x}}} \boldsymbol{{dx}}\:+\boldsymbol{{e}}^{\boldsymbol{{x}}} \boldsymbol{{tan}}\frac{\boldsymbol{{x}}}{\mathrm{2}}−\frac{\mathrm{1}}{\mathrm{2}}\int\boldsymbol{{sec}}^{\mathrm{2}} \frac{\boldsymbol{{x}}}{\mathrm{2}}\boldsymbol{{e}}^{\boldsymbol{{x}}} \boldsymbol{{dx}}\: \\ $$$$\boldsymbol{{e}}^{\boldsymbol{{x}}} \boldsymbol{{tan}}\frac{\boldsymbol{{x}}}{\mathrm{2}}\:+\boldsymbol{{C}} \\ $$
Commented by bekzodjumayev last updated on 26/May/21

$${Thank}\:{you} \\ $$
Answered by Avijit007 last updated on 26/May/21
![The given integral is, ∫((1+sin x)/(1+cos x))e^x .dx =∫[e^x {(1/(1+cosx))+((sinx)/(1+sinx))}].dx =∫[e^x {(1/(2cos^2 ((x/2))))+((2sin((x/2))cos((x/2)))/(2cos^2 ((x/2))))].dx =∫[e^x {(1/2)sec^2 ((x/2))+tan((x/2))].dx ⇓ ⇓ f ′(x) f(x) ∵ we know that, ∫e^x {f ′(x)+ f(x)} ⇒e^x f(x)+c = e^x .tan((x/2))+c {ANS} by Avijit](https://www.tinkutara.com/question/Q142128.png)
$${The}\:{given}\:{integral}\:{is}, \\ $$$$\int\frac{\mathrm{1}+{sin}\:{x}}{\mathrm{1}+{cos}\:{x}}{e}^{{x}} .{dx} \\ $$$$=\int\left[{e}^{{x}} \left\{\frac{\mathrm{1}}{\mathrm{1}+{cosx}}+\frac{{sinx}}{\mathrm{1}+{sinx}}\right\}\right].{dx} \\ $$$$=\int\left[{e}^{{x}} \left\{\frac{\mathrm{1}}{\mathrm{2}{cos}^{\mathrm{2}} \left(\frac{{x}}{\mathrm{2}}\right)}+\frac{\mathrm{2}{sin}\left(\frac{{x}}{\mathrm{2}}\right){cos}\left(\frac{{x}}{\mathrm{2}}\right)}{\mathrm{2}{cos}^{\mathrm{2}} \left(\frac{{x}}{\mathrm{2}}\right)}\right].{dx}\right. \\ $$$$=\int\left[{e}^{{x}} \left\{\frac{\mathrm{1}}{\mathrm{2}}{sec}^{\mathrm{2}} \left(\frac{{x}}{\mathrm{2}}\right)+{tan}\left(\frac{{x}}{\mathrm{2}}\right)\right].{dx}\right. \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\Downarrow\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\Downarrow \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:{f}\:'\left({x}\right)\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:{f}\left({x}\right) \\ $$$$\because\:{we}\:{know}\:{that},\:\int{e}^{{x}} \:\left\{{f}\:'\left({x}\right)+\:{f}\left({x}\right)\right\} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\Rightarrow{e}^{{x}} {f}\left({x}\right)+{c} \\ $$$$=\:{e}^{{x}} .{tan}\left(\frac{{x}}{\mathrm{2}}\right)+{c}\:\:\:\:\:\:\left\{\mathcal{ANS}\right\}\:\: \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:{by}\:{Avijit} \\ $$$$ \\ $$
