Question Number 76586 by Master last updated on 28/Dec/19

Commented by Master last updated on 28/Dec/19

Commented by Tony Lin last updated on 28/Dec/19

Commented by john santu last updated on 28/Dec/19
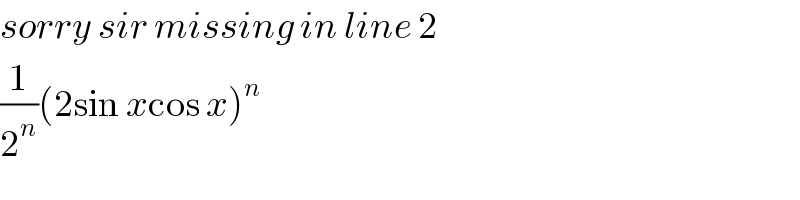
Commented by Tony Lin last updated on 28/Dec/19

Commented by Master last updated on 28/Dec/19
Commented by Master last updated on 28/Dec/19

Commented by MJS last updated on 28/Dec/19
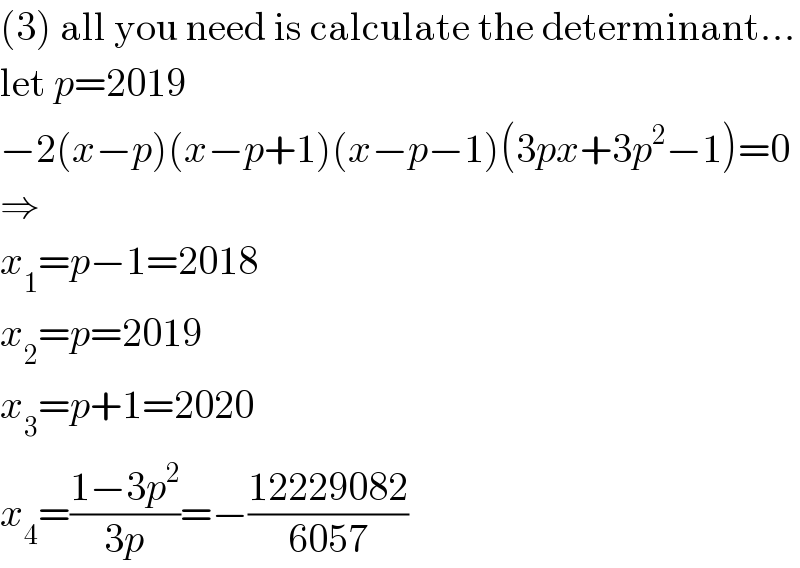
Answered by benjo 1/2 santuyy last updated on 29/Dec/19

