Question Number 142282 by pete last updated on 29/May/21
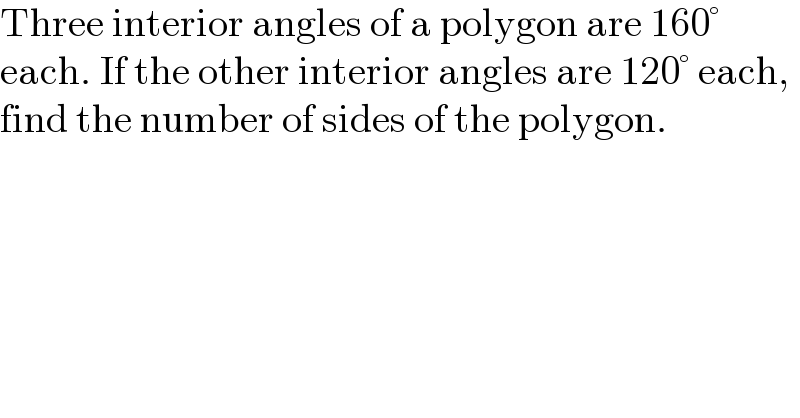
$$\mathrm{Three}\:\mathrm{interior}\:\mathrm{angles}\:\mathrm{of}\:\mathrm{a}\:\mathrm{polygon}\:\mathrm{are}\:\mathrm{160}° \\ $$$$\mathrm{each}.\:\mathrm{If}\:\mathrm{the}\:\mathrm{other}\:\mathrm{interior}\:\mathrm{angles}\:\mathrm{are}\:\mathrm{120}°\:\mathrm{each}, \\ $$$$\mathrm{find}\:\mathrm{the}\:\mathrm{number}\:\mathrm{of}\:\mathrm{sides}\:\mathrm{of}\:\mathrm{the}\:\mathrm{polygon}. \\ $$
Answered by som(math1967) last updated on 29/May/21

$$\boldsymbol{{let}}\:\boldsymbol{{no}}\:\boldsymbol{{of}}\:\boldsymbol{{sides}}=\boldsymbol{{n}} \\ $$$$\mathrm{3}×\mathrm{160}+\left(\boldsymbol{{n}}−\mathrm{3}\right)×\mathrm{120}=\left(\boldsymbol{{n}}−\mathrm{2}\right)×\mathrm{180} \\ $$$$\mathrm{480}−\mathrm{360}+\mathrm{120}\boldsymbol{{n}}=\mathrm{180}\boldsymbol{{n}}−\mathrm{360} \\ $$$$\mathrm{60}\boldsymbol{{n}}=\mathrm{480}\Rightarrow\boldsymbol{{n}}=\mathrm{7} \\ $$$$\boldsymbol{{no}}\:\boldsymbol{{of}}\:\boldsymbol{{sides}}=\mathrm{7} \\ $$
Commented by pete last updated on 31/May/21

$$\mathrm{thank}\:\mathrm{you}\:\mathrm{very}\:\mathrm{much}\:\mathrm{sir} \\ $$
