Question-76913

Question Number 76913 by Ajao yinka last updated on 31/Dec/19

Answered by ~blr237~ last updated on 01/Jan/20

let it be A 1+x+....+x^(100) =((1−x^(101) )/(1−x)) A=∫_0 ^1 ((x^(49) (1−x))/(1−x^(101) ))dx=∫_0 ^1 x^(49) (1−x)Σ_(n=0) ^∞ x^(101n) dx A=Σ_(n=0) ^∞ ∫_0 ^1 x^(101n+49) (1−x)dx =Σ_(n=0) ^∞ B(101n+50,2) =Σ_(n=0) ^∞ ((Γ(101n+50)Γ(2))/(Γ(101n+52))) =Σ_(n=0) ^∞ (1/((101n+51)(101n+50))) =(1/((101)^2 )) Σ_(n=0) ^∞ (1/((n+((51)/(101)))(n+((50)/(101))))) = (1/(101^2 )) × ((ψ(((51)/(101)))−ψ(((50)/(101))))/(((51)/(101))−((50)/(101)))) =((ψ(((51)/(101)))−ψ(((50)/(101))))/(101)) with ψ=((Γ′)/Γ)

$$\mathrm{let}\:\mathrm{it}\:\mathrm{be}\:\mathrm{A} \\ $$$$\mathrm{1}+\mathrm{x}+….+\mathrm{x}^{\mathrm{100}} =\frac{\mathrm{1}−\mathrm{x}^{\mathrm{101}} }{\mathrm{1}−\mathrm{x}} \\ $$$$\mathrm{A}=\int_{\mathrm{0}} ^{\mathrm{1}} \frac{\mathrm{x}^{\mathrm{49}} \left(\mathrm{1}−\mathrm{x}\right)}{\mathrm{1}−\mathrm{x}^{\mathrm{101}} }\mathrm{dx}=\int_{\mathrm{0}} ^{\mathrm{1}} \mathrm{x}^{\mathrm{49}} \left(\mathrm{1}−\mathrm{x}\right)\underset{\mathrm{n}=\mathrm{0}} {\overset{\infty} {\sum}}\mathrm{x}^{\mathrm{101n}} \mathrm{dx} \\ $$$$\mathrm{A}=\underset{\mathrm{n}=\mathrm{0}} {\overset{\infty} {\sum}}\:\int_{\mathrm{0}} ^{\mathrm{1}} \mathrm{x}^{\mathrm{101n}+\mathrm{49}} \left(\mathrm{1}−\mathrm{x}\right)\mathrm{dx} \\ $$$$=\underset{\mathrm{n}=\mathrm{0}} {\overset{\infty} {\sum}}\mathrm{B}\left(\mathrm{101n}+\mathrm{50},\mathrm{2}\right) \\ $$$$=\underset{\mathrm{n}=\mathrm{0}} {\overset{\infty} {\sum}}\frac{\Gamma\left(\mathrm{101n}+\mathrm{50}\right)\Gamma\left(\mathrm{2}\right)}{\Gamma\left(\mathrm{101n}+\mathrm{52}\right)} \\ $$$$=\underset{\mathrm{n}=\mathrm{0}} {\overset{\infty} {\sum}}\frac{\mathrm{1}}{\left(\mathrm{101n}+\mathrm{51}\right)\left(\mathrm{101n}+\mathrm{50}\right)}\:=\frac{\mathrm{1}}{\left(\mathrm{101}\right)^{\mathrm{2}} }\:\underset{\mathrm{n}=\mathrm{0}} {\overset{\infty} {\sum}}\:\frac{\mathrm{1}}{\left(\mathrm{n}+\frac{\mathrm{51}}{\mathrm{101}}\right)\left(\mathrm{n}+\frac{\mathrm{50}}{\mathrm{101}}\right)}\: \\ $$$$=\:\frac{\mathrm{1}}{\mathrm{101}^{\mathrm{2}} }\:×\:\frac{\psi\left(\frac{\mathrm{51}}{\mathrm{101}}\right)−\psi\left(\frac{\mathrm{50}}{\mathrm{101}}\right)}{\frac{\mathrm{51}}{\mathrm{101}}−\frac{\mathrm{50}}{\mathrm{101}}}\:=\frac{\psi\left(\frac{\mathrm{51}}{\mathrm{101}}\right)−\psi\left(\frac{\mathrm{50}}{\mathrm{101}}\right)}{\mathrm{101}}\:\:\:\mathrm{with}\:\psi=\frac{\Gamma'}{\Gamma}\: \\ $$$$ \\ $$

Commented by jagoll last updated on 01/Jan/20

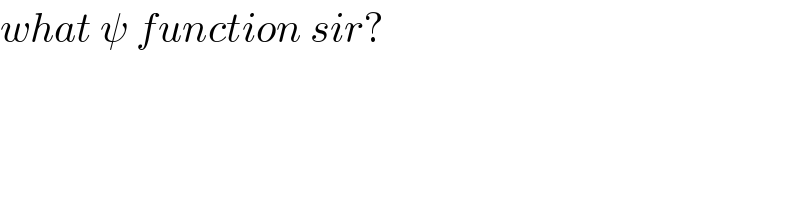
$${what}\:\psi\:{function}\:{sir}? \\ $$

Commented by 20092104 last updated on 10/Feb/20

digamma function ψ(x)=(d/dx)ln(Γ(x))=((Γ′(x))/(Γ(x)))

$${digamma}\:{function} \\ $$$$\psi\left({x}\right)=\frac{{d}}{{dx}}\mathrm{ln}\left(\Gamma\left({x}\right)\right)=\frac{\Gamma'\left({x}\right)}{\Gamma\left({x}\right)} \\ $$

Facebook Tweet Pin

Leave a Reply Cancel reply