Question Number 142679 by Gbenga last updated on 03/Jun/21

$${how}\:{to}\:{get}\:{exert}\:{value}\:{of}\:{a}\:{lambert}\:{w}\:{function}\:{question}\:{without}\:{wolfram}\:{alpha} \\ $$
Commented by Dwaipayan Shikari last updated on 03/Jun/21
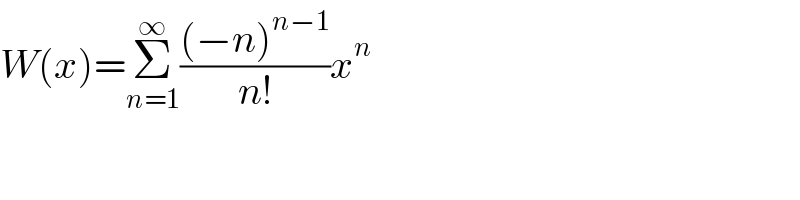
$${W}\left({x}\right)=\underset{{n}=\mathrm{1}} {\overset{\infty} {\sum}}\frac{\left(−{n}\right)^{{n}−\mathrm{1}} }{{n}!}{x}^{{n}} \\ $$
Commented by Gbenga last updated on 03/Jun/21

$${example}\:{please} \\ $$
Commented by Dwaipayan Shikari last updated on 03/Jun/21

$${x}^{{x}} =\mathrm{2} \\ $$$${xlog}\left({x}\right)={log}\left(\mathrm{2}\right) \\ $$$${e}^{{log}\left({x}\right)} {log}\left({x}\right)={log}\left(\mathrm{2}\right) \\ $$$${log}\left({x}\right)={W}_{\mathrm{0}} \left({log}\left(\mathrm{2}\right)\right) \\ $$$${x}={exp}\left({W}_{\mathrm{0}} \left({log}\left(\mathrm{2}\right)\right)\right) \\ $$$${x}={exp}\left({log}\left(\mathrm{2}\right)−{log}^{\mathrm{2}} \left(\mathrm{2}\right)+\frac{\mathrm{3}}{\mathrm{2}}{log}^{\mathrm{3}} \left(\mathrm{2}\right)−…\right) \\ $$
Commented by Gbenga last updated on 03/Jun/21
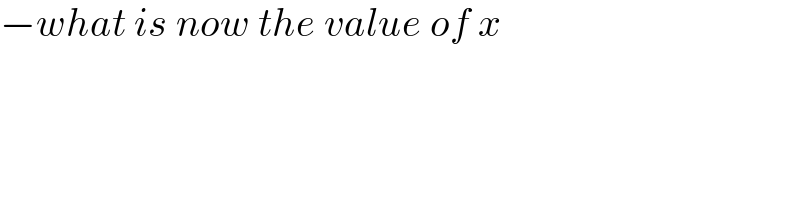
$$−{what}\:{is}\:{now}\:{the}\:{value}\:{of}\:{x} \\ $$
Commented by Gbenga last updated on 03/Jun/21

$$\mathrm{4}^{{x}=\mathrm{4}{x}} \\ $$
