0-t-n-2-costdt-

Question Number 144432 by ArielVyny last updated on 25/Jun/21

$$\int_{\mathrm{0}} ^{\propto} {t}^{{n}−\mathrm{2}} {costdt} \\ $$

Answered by Dwaipayan Shikari last updated on 25/Jun/21

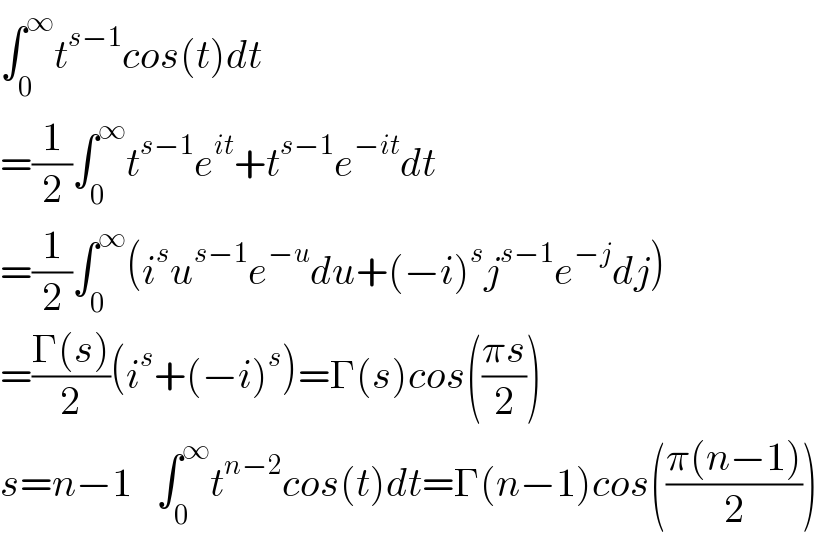
∫_0 ^∞ t^(s−1) cos(t)dt =(1/2)∫_0 ^∞ t^(s−1) e^(it) +t^(s−1) e^(−it) dt =(1/2)∫_0 ^∞ (i^s u^(s−1) e^(−u) du+(−i)^s j^(s−1) e^(−j) dj) =((Γ(s))/2)(i^s +(−i)^s )=Γ(s)cos(((πs)/2)) s=n−1 ∫_0 ^∞ t^(n−2) cos(t)dt=Γ(n−1)cos(((π(n−1))/2))

$$\int_{\mathrm{0}} ^{\infty} {t}^{{s}−\mathrm{1}} {cos}\left({t}\right){dt} \\ $$$$=\frac{\mathrm{1}}{\mathrm{2}}\int_{\mathrm{0}} ^{\infty} {t}^{{s}−\mathrm{1}} {e}^{{it}} +{t}^{{s}−\mathrm{1}} {e}^{−{it}} {dt}\:\:\: \\ $$$$=\frac{\mathrm{1}}{\mathrm{2}}\int_{\mathrm{0}} ^{\infty} \left({i}^{{s}} {u}^{{s}−\mathrm{1}} {e}^{−{u}} {du}+\left(−{i}\right)^{{s}} {j}^{{s}−\mathrm{1}} {e}^{−{j}} {dj}\right) \\ $$$$=\frac{\Gamma\left({s}\right)}{\mathrm{2}}\left({i}^{{s}} +\left(−{i}\right)^{{s}} \right)=\Gamma\left({s}\right){cos}\left(\frac{\pi{s}}{\mathrm{2}}\right) \\ $$$${s}={n}−\mathrm{1}\:\:\:\int_{\mathrm{0}} ^{\infty} {t}^{{n}−\mathrm{2}} {cos}\left({t}\right){dt}=\Gamma\left({n}−\mathrm{1}\right){cos}\left(\frac{\pi\left({n}−\mathrm{1}\right)}{\mathrm{2}}\right) \\ $$

Facebook Tweet Pin

0-t-n-2-costdt-

Leave a Reply Cancel reply