∫_( 0) ^( a/2) x^2 (a^2 − x^2 )^(3/2) dx


Question and Answers Forum

All Questions Topic List
Integration Questions
Previous in All Question Next in All Question
Previous in Integration Next in Integration
Question Number 17603 by tawa tawa last updated on 08/Jul/17

$\int_{0}^{a / 2} x^{2} {(a^{2} - x^{2})}^{\frac{3}{2}} dx$
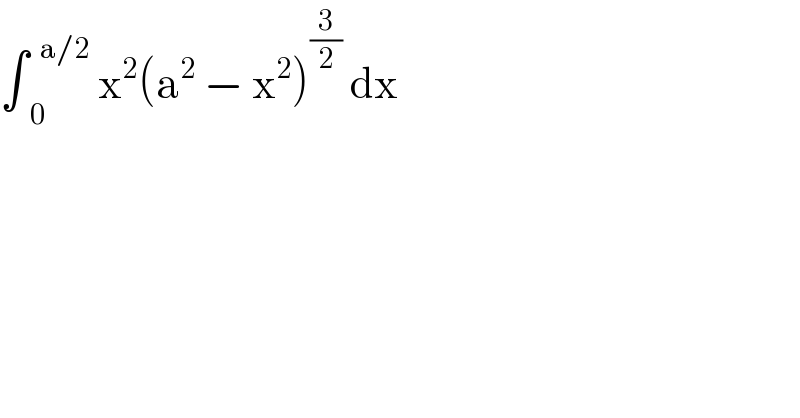
	Answered by alex041103 last updated on 08/Jul/17

	$I = \int_{0}^{a / 2} x^{2} {(a^{2} - x^{2})}^{\frac{3}{2}} dx$ $L e t x = a s i n θ (i . e . θ = {s i n}^{- 1} (\frac{x}{a}))$ $\Rightarrow d x = a c o s θ$ $W e u s e 1 - {s i n}^{2} θ = {c o s}^{2} θ a n d$ $θ = {s i n}^{- 1} (\frac{x}{a}) t o c h a n g e t h e l i m i t s o f$ $i n t e g r a t i o n :$ $I = a^{6} \underset{0}{\int^{π / 6}} {s i n}^{2} θ {c o s}^{4} θ d θ$ $T h e n w e s u b s t i t u t e {s i n}^{2} θ = 1 - {c o s}^{2} θ$ $I = a^{6} [\underset{0}{\int^{π / 6}} {c o s}^{4} θ d θ - \underset{0}{\int^{π / 6}} {c o s}^{6} θ d θ]$ $L e t I_{n} = \underset{0}{\int^{π / 6}} {c o s}^{n} θ d θ$ $\Rightarrow I = a^{6} (I_{4} - I_{6})$ $I_{n} = \underset{0}{\int^{π / 6}} {c o s}^{n - 1} θ c o s θ d θ$ $T h e n w e u s e i n t e g r a t i o n b y p a r t s :$ $u = {c o s}^{n - 1} θ d v = c o s θ d θ$ $d u = (n - 1) (- s i n θ) {c o s}^{n - 2} θ v = s i n θ$ $\underset{a}{\int^{b}} u d v = {[u v]}_{a}^{b} - \underset{a}{\int^{b}} v d u$ $I_{n} = {[{c o s}^{n - 1} θ s i n θ]}_{0}^{π / 6} + (n - 1) \underset{0}{\int^{π / 6}} {s i n}^{2} θ {c o s}^{n - 2} θ d θ$ $W e k n o w t h a t {s i n}^{2} θ = 1 - {c o s}^{2} θ$ $\Rightarrow I_{n} = \frac{3^{\frac{1}{2} (n - 1)}}{2^{n}} + (n - 1) (\underset{0}{\int^{π / 6}} {c o s}^{n - 2} θ d θ - \underset{0}{\int^{π / 6}} {c o s}^{n} θ d θ)$ $B u t$ $I_{n} = \underset{0}{\int^{π / 6}} {c o s}^{n} θ d θ$ $I_{n - 2} = \underset{0}{\int^{π / 6}} {c o s}^{n - 2} θ d θ$ $\Rightarrow I_{n} = \frac{3^{\frac{n - 1}{2}}}{2^{n}} + (n - 1) I_{n - 2} - (n - 1) I_{n}$ $i . e . I_{n} = \frac{3^{\frac{n - 1}{2}}}{n 2^{n}} + \frac{n - 1}{n} I_{n - 2}$ $W e u s e t h e f o r m u l a f o r I = a^{6} (I_{6 - 2} - I_{6})$ $a n d w e g e t$ $I = a^{6} (I_{6 - 2} - (\frac{3 \sqrt{3}}{2^{7}} + \frac{5}{6} I_{6 - 2}))$ $= a^{6} (\frac{1}{6} I_{4} - \frac{3 \sqrt{3}}{2^{7}})$ $N o w w d u s e t h e f o r m u l a f o r I_{n} t w i c e$ $t o e v a l u a t e I_{4} (i . e . e x p r e s s i n g I_{4} i n t e r m s o f I_{0}) a n d w e g e t$ $I_{4} = \frac{3 \sqrt{3}}{2^{6}} + \frac{3 \sqrt{3}}{2^{5}} + \frac{π}{2^{4}}$ $N o w w e f i n a l y g e t f o r I :$ $I = \underset{0}{\int^{a / 2}} x^{2} {(a^{2} - x^{2})}^{3 / 2} d x = \frac{a^{6} π}{96}$
	Commented by tawa tawa last updated on 08/Jul/17

	$God bless you sir . i really appreciate .$
	Answered by Arnab Maiti last updated on 13/Jul/17
	$let I =∫_0 ^( (a/2)) x^2 (a^2 −x^2 )^(3/2) dx put x=a sinθ ∴ dx= a cosθ dθ ∴ I=∫_0 ^( (π/6)) a^3 sin^2 θ{a^2 (1−sin^2 θ)}^(3/2) cosθ dθ =a^6 ∫_0 ^(π/6) sin^2 θ cos^4 θ dθ =a^6 (1/8)∫_0 ^( (π/6)) (2sinθ cosθ)^2 2cos^2 θ dθ =a^6 ×(1/8)∫_0 ^(π/6) sin^2 2θ (1+cos2θ)dθ =a^6 ×(1/8)[∫_0 ^(π/6) sin^2 2θ dθ+∫_0 ^(π/6) sin^2 2θ cos2θ dθ] =a^6 /8×[(1/2)∫_0 ^(π/6) (1−cos4θ)dθ+(1/2)∫_0 ^((√3)/2) (sin 2θ)^2 d(sin 2θ)] =(a^6 /(16))[ θ−((sin 4θ)/4)]_0 ^(π/6) +(a^6 /(16))[(((p)^3 )/3)]_0 ^((√3)/2) let sin2θ=p =(a^6 /(16))[{(π/6)−((√3)/8)}+((3(√3))/(3×8))] =(a^6 /(16))×(π/6)=((πa^6 )/(96))$
	$let I = \int_{0}^{\frac{a}{2}} x^{2} {(a^{2} - x^{2})}^{\frac{3}{2}} dx$ $put x = a \sin θ ∴ dx = a \cos θ d θ$ $∴ I = \int_{0}^{\frac{π}{6}} a^{3} \sin^{2} θ {a^{2} (1 - \sin^{2} θ)}^{\frac{3}{2}} \cos θ d θ$ $= a^{6} \int_{0}^{\frac{π}{6}} \sin^{2} θ \cos^{4} θ d θ$ $= a^{6} \frac{1}{8} \int_{0}^{\frac{π}{6}} {(2 \sin θ \cos θ)}^{2} {2 \cos}^{2} θ d θ$ $= a^{6} \times \frac{1}{8} \int_{0}^{\frac{π}{6}} \sin^{2} 2 θ (1 + \cos 2 θ) d θ$ $= a^{6} \times \frac{1}{8} [\int_{0}^{\frac{π}{6}} \sin^{2} 2 θ d θ + \int_{0}^{\frac{π}{6}} \sin^{2} 2 θ \cos 2 θ d θ]$ $= a^{6} / 8 \times [\frac{1}{2} \int_{0}^{\frac{π}{6}} (1 - \cos 4 θ) d θ + \frac{1}{2} \int_{0}^{\frac{\sqrt{3}}{2}} {(\sin 2 θ)}^{2} d (\sin 2 θ)]$ $= \frac{a^{6}}{16} {[θ - \frac{\sin 4 θ}{4}]}_{0}^{\frac{π}{6}} + \frac{a^{6}}{16} {[\frac{{(p)}^{3}}{3}]}_{0}^{\frac{\sqrt{3}}{2}} let \sin 2 θ = p$ $= \frac{a^{6}}{16} [{\frac{π}{6} - \frac{\sqrt{3}}{8}} + \frac{3 \sqrt{3}}{3 \times 8}]$ $= \frac{a^{6}}{16} \times \frac{π}{6} = \frac{π a^{6}}{96}$
	Commented by tawa tawa last updated on 11/Jul/17

	$God bless you sir .$
	Commented by alex041103 last updated on 11/Jul/17
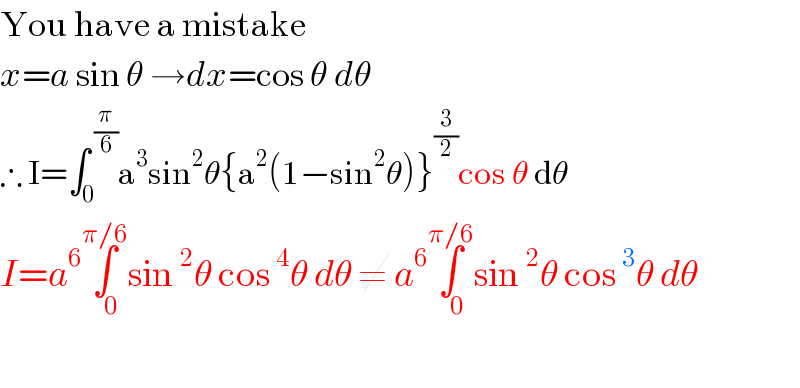
	$You have a mistake x=a sin θ →dx=cos θ dθ ∴ I=∫_0 ^( (π/6)) a^3 sin^2 θ{a^2 (1−sin^2 θ)}^(3/2) cos θ dθ I=a^6 ∫_( 0) ^(π/6) sin^2 θ cos^4 θ dθ ≠ a^6 ∫_( 0) ^(π/6) sin^2 θ cos^3 θ dθ$
	$You have a mistake$ $x = a \sin θ \to d x = \cos θ d θ$ $∴ I = \int_{0}^{\frac{π}{6}} a^{3} \sin^{2} θ {a^{2} (1 - \sin^{2} θ)}^{\frac{3}{2}} \cos θ d θ$ $I = a^{6} \underset{0}{\int^{π / 6}} \sin^{2} θ \cos^{4} θ d θ \neq a^{6} \underset{0}{\int^{π / 6}} \sin^{2} θ \cos^{3} θ d θ$
	Commented by Arnab Maiti last updated on 12/Jul/17

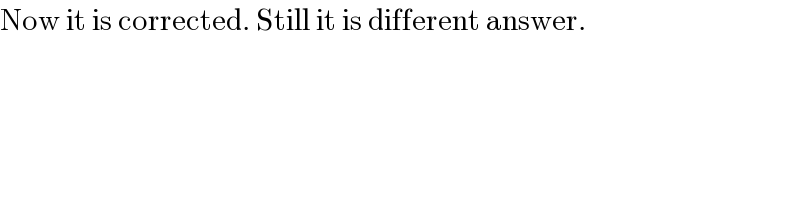
	$Now it is corrected . Still it is different answer .$
	Commented by alex041103 last updated on 13/Jul/17

	$Y o u n o w h a v e a n o t h e r m i s t a k e$ $A t l a s t l i n e s w h e r e y o u f i n i s h t h e$ $i n t e g r a t i o n y o u s e t {s i n}^{2} 2 θ = \frac{1 - c o s 2 θ}{2}$ $w h i c h i s n o t t r u e$ $\frac{1 - c o s 2 θ}{2} = {s i n}^{2} θ \neq {s i n}^{2} 2 θ$
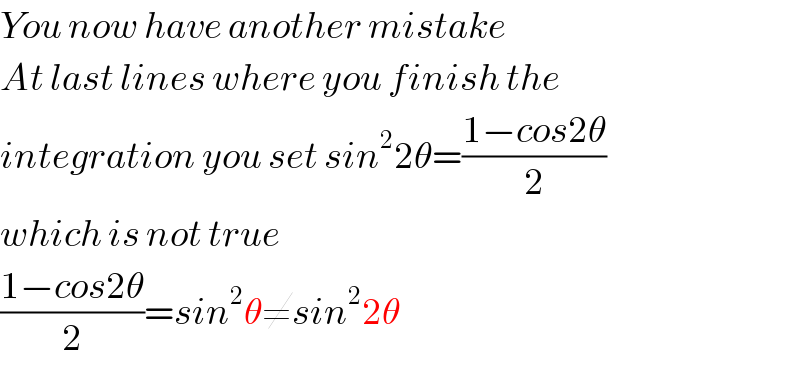
	Commented by Arnab Maiti last updated on 13/Jul/17

	$The answer is indentical now .$ $Thank you!!$
Terms of Service
Privacy Policy
Contact: info@tinkutara.com

Question and Answers Forum