∫_0 ^(π/4) ((tsint)/(1+cos^2 t))dt=∫_0 ^(π/4) ((tcost)/(1+sin^2 t))dt true or false ??


Question and Answers Forum

All Questions Topic List
Integration Questions
Previous in All Question Next in All Question
Previous in Integration Next in Integration
Question Number 149023 by ArielVyny last updated on 02/Aug/21

$\int_{0}^{\frac{π}{4}} \frac{t s i n t}{1 + {c o s}^{2} t} d t = {\int^{\frac{π}{4}}}_{0} \frac{t c o s t}{1 + {s i n}^{2} t} d t$ $t r u e o r f a l s e ? ?$
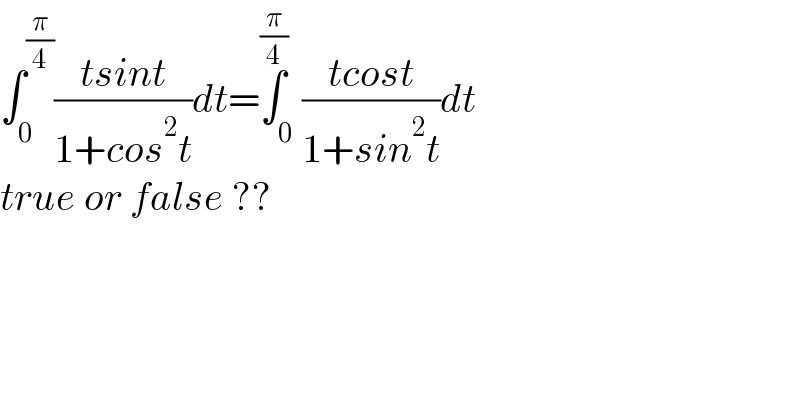
	Answered by mindispower last updated on 02/Aug/21

	$\frac{s i n (t)}{1 + {c o s}^{2} (t)} < \frac{c o s (t)}{1 + {s i n}^{2} (t)}, \forall t \in [0, \frac{π}{4} [$ $p r o f f c o s (t) > s i n (t) ⩾ 0 \Rightarrow$ $\frac{1}{1 + {c o s}^{2} (t)} < \frac{1}{1 + {s i n}^{2} (t)} \Rightarrow \frac{s i n (t)}{1 + {c o s}^{2} (t)} < \frac{c o s (t)}{1 + {s i n}^{2} (t)}$ $\Rightarrow 0 ⩽ t \frac{s i n (t)}{1 + {c o s}^{2} (t)} < \frac{t c o s (t)}{1 + {s i n}^{2} (t)}$ $\int_{0}^{\frac{π}{4}} \frac{t s i n (t)}{1 + {c o s}^{2} (t)} d t < \int_{0}^{\frac{π}{4}} \frac{t c o s (t)}{1 + {s i n}^{2} (t)} d t$
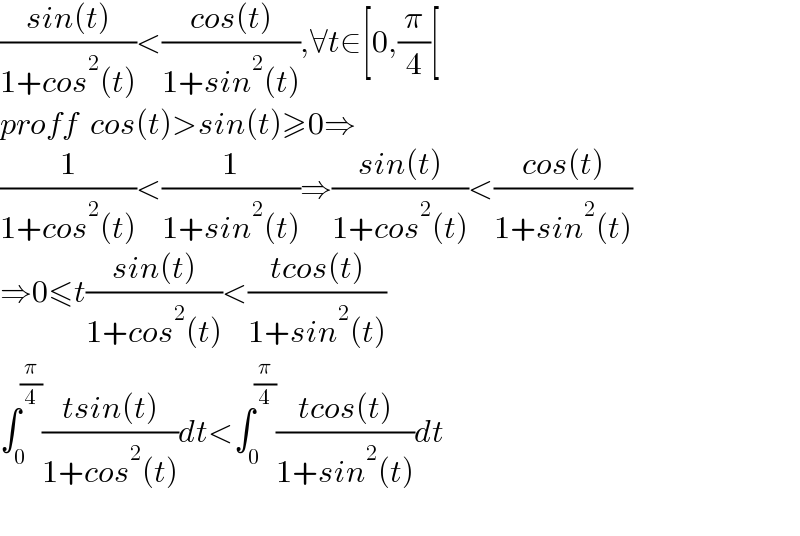
	Commented by mindispower last updated on 03/Aug/21

	$y e s w i t h e s p e c i a l f u n c t i o n$
	Commented by ArielVyny last updated on 02/Aug/21

	$s i r y o u c a n f i n d t h e v a l u e o f t h i s i n t e g r a l ?$
	Commented by ArielVyny last updated on 06/Aug/21

	$y o u c a n s o l v e i t p l e a s e$
Terms of Service
Privacy Policy
Contact: info@tinkutara.com

Question and Answers Forum