
Question and Answers Forum
Question Number 101833 by bobhans last updated on 05/Jul/20
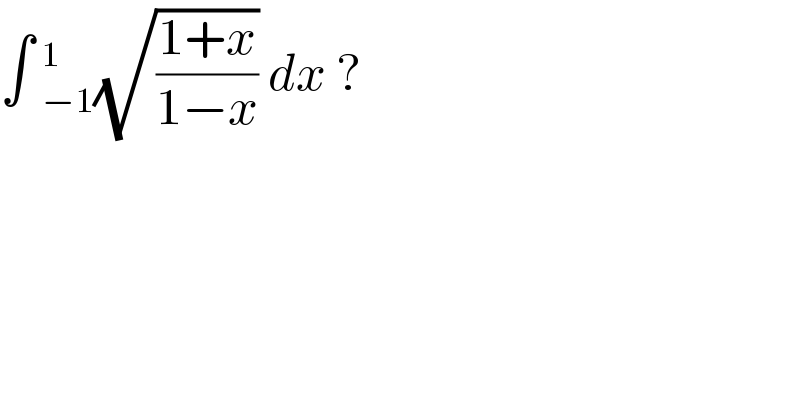
Answered by Dwaipayan Shikari last updated on 05/Jul/20
![∫_(−1) ^1 ((1+x)/(√(1−x^2 )))dx=∫_(−1) ^1 (1/(√(1−x^2 )))+(x/(√(1−x^2 )))=[sin^(−1) x]_(−1) ^1 −(1/2)∫_(−1) ^1 ((−2x)/(√(1−x^2 )))dx =π−(1/2)[(√(1−x^2 ))]_(−1) ^1 =π+0=π](Q101862.png)
Answered by john santu last updated on 05/Jul/20
![set (√((1+x)/(1−x))) = s →dx = ((4s)/((s^2 +1)^2 )) ds I = ∫_0 ^∞ ((4s^2 )/((s^2 +1)^2 )) ds [ by parts ] { ((u = s)),((dv = ((4s ds)/((s^2 +1)^2 )))) :} I= −2.∣(s/(s^2 +1)) ∣_0 ^∞ −∫−((2 ds)/(s^2 +1)) I = 0+[ 2 arctan (s) ]_0 ^∞ I = 2((π/2)) = π (JS ⊛)](Q101834.png)
Answered by mathmax by abdo last updated on 05/Jul/20
![A =∫_(−1) ^1 (√((1+x)/(1−x)))dx changement x =cost give A =−∫_0 ^π (√((1+cost)/(1−cost)))(−sint)dt =∫_0 ^π (√((2cos^2 ((t/2)))/(2sin^2 ((t/2)))))sint dt =∫_0 ^π ((cos((t/2)))/(sin((t/2)))) ×2sin((t/2))cos((t/2))dt =2∫_0 ^(π ) cos^2 ((t/2))dt =∫_0 ^π (1+cost)dt =π +∫_0 ^π cost dt =π +[sint]_0 ^π =π+0 ⇒A =π](Q101896.png)
| ||
Question and Answers Forum | ||
Question Number 101833 by bobhans last updated on 05/Jul/20 | ||
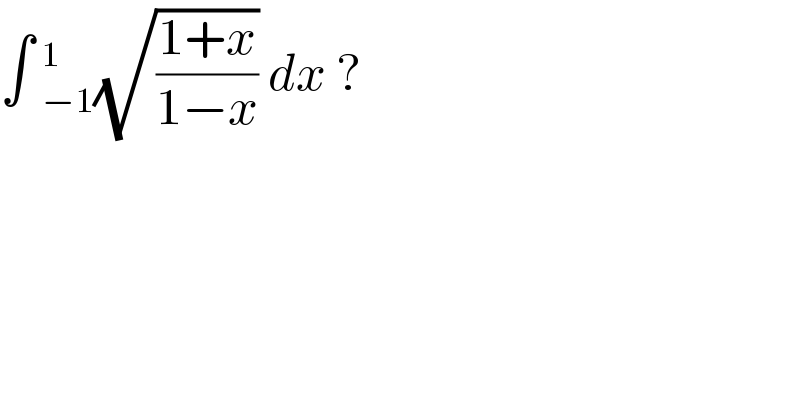 | ||
Answered by Dwaipayan Shikari last updated on 05/Jul/20 | ||
![∫_(−1) ^1 ((1+x)/(√(1−x^2 )))dx=∫_(−1) ^1 (1/(√(1−x^2 )))+(x/(√(1−x^2 )))=[sin^(−1) x]_(−1) ^1 −(1/2)∫_(−1) ^1 ((−2x)/(√(1−x^2 )))dx =π−(1/2)[(√(1−x^2 ))]_(−1) ^1 =π+0=π](Q101862.png) | ||
| ||
Answered by john santu last updated on 05/Jul/20 | ||
![set (√((1+x)/(1−x))) = s →dx = ((4s)/((s^2 +1)^2 )) ds I = ∫_0 ^∞ ((4s^2 )/((s^2 +1)^2 )) ds [ by parts ] { ((u = s)),((dv = ((4s ds)/((s^2 +1)^2 )))) :} I= −2.∣(s/(s^2 +1)) ∣_0 ^∞ −∫−((2 ds)/(s^2 +1)) I = 0+[ 2 arctan (s) ]_0 ^∞ I = 2((π/2)) = π (JS ⊛)](Q101834.png) | ||
| ||
Answered by mathmax by abdo last updated on 05/Jul/20 | ||
![A =∫_(−1) ^1 (√((1+x)/(1−x)))dx changement x =cost give A =−∫_0 ^π (√((1+cost)/(1−cost)))(−sint)dt =∫_0 ^π (√((2cos^2 ((t/2)))/(2sin^2 ((t/2)))))sint dt =∫_0 ^π ((cos((t/2)))/(sin((t/2)))) ×2sin((t/2))cos((t/2))dt =2∫_0 ^(π ) cos^2 ((t/2))dt =∫_0 ^π (1+cost)dt =π +∫_0 ^π cost dt =π +[sint]_0 ^π =π+0 ⇒A =π](Q101896.png) | ||
| ||