Math Question and Answers


Question and Answers Forum

All Questions Topic List
Mechanics Questions
Previous in All Question Next in All Question
Previous in Mechanics Next in Mechanics
Question Number 100928 by ajfour last updated on 29/Jun/20

Commented by ajfour last updated on 29/Jun/20

$F i n d r i n t e r m s o f R .$
Commented by ajfour last updated on 30/Jun/20

$I t s p h y s i c s p l u s m a t h s, S i r .$
	Answered by bramlex last updated on 29/Jun/20

	Commented by bramlex last updated on 29/Jun/20

	$x^{2} + {(R - r)}^{2} = {(R + r)}^{2}$ $x^{2} - 2 r R = 2 r R \to x = 2 \sqrt{r R}$ $(1) {(x - R)}^{2} + {(y - R)}^{2} = R^{2}$ $\to x^{2} + y^{2} - 2 R x - 2 R y + R^{2} = 0$ $(2) (x - {(2 \sqrt{r R} + r)}^{2} + {(y - r)}^{2} = r^{2}$ $\to x^{2} - 2 (r + 2 \sqrt{r R}) x + {(r + 2 \sqrt{r R})}^{2} + y^{2} - 2 r y = 0$ $. . .$
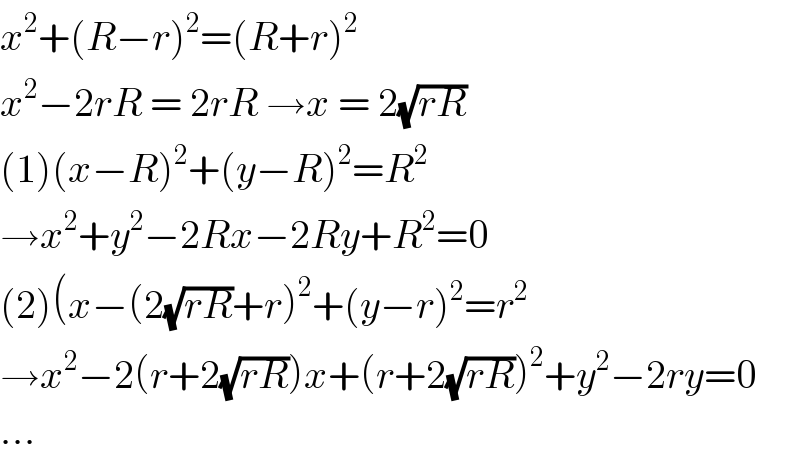
	Answered by mr W last updated on 29/Jun/20

	Commented by mr W last updated on 29/Jun/20

	$a t p o s i t i o n θ :$ $\frac{1}{2} {m u}^{2} = R (1 - \cos θ) m g$ $\Rightarrow u = \sqrt{2 R (1 - \cos θ) g}$ $m g \cos θ - N = \frac{{m u}^{2}}{R}$ $N = 0 w h e n c o n t a c t g e t s l o s t$ $m g \cos θ = \frac{m \times 2 R (1 - \cos θ) g}{R}$ $\Rightarrow \cos θ = \frac{2}{3}$ $\Rightarrow u = \sqrt{\frac{2 R g}{3}}$ $t r a c k o f m a s s m a f t e r p o i n t B :$ $x = R \sin θ + u \cos θ t$ $\Rightarrow x = \frac{\sqrt{5} R}{3} + \frac{2}{3} \sqrt{\frac{2 R g}{3}} t$ $y = R (1 + \cos θ) - u \sin θ t - \frac{1}{2} {g t}^{2}$ $\Rightarrow y = \frac{5 R}{3} - \frac{\sqrt{5}}{3} \sqrt{\frac{2 R g}{3}} t - \frac{1}{2} {g t}^{2}$ $D (\sqrt{{(R + r)}^{2} - {(R - r)}^{2}}, r) \equiv D (2 \sqrt{R r}, r)$ $l e t Φ = {D C}^{2}$ $Φ = {(x - 2 \sqrt{R r})}^{2} + {(y - r)}^{2}$ $Φ = {(\frac{\sqrt{5} R}{3} + \frac{2}{3} \sqrt{\frac{2 R g}{3}} t - 2 \sqrt{R r})}^{2} + {(\frac{5 R}{3} - \frac{\sqrt{5}}{3} \sqrt{\frac{2 R g}{3}} t - \frac{1}{2} {g t}^{2} - r)}^{2}$ $\frac{d Φ}{d t} = 2 (\frac{\sqrt{5} R}{3} + \frac{2}{3} \sqrt{\frac{2 R g}{3}} t - 2 \sqrt{R r}) (\frac{2}{3} \sqrt{\frac{2 R g}{3}}) + 2 (\frac{5 R}{3} - \frac{\sqrt{5}}{3} \sqrt{\frac{2 R g}{3}} t - \frac{1}{2} {g t}^{2} - r) (- \frac{\sqrt{5}}{3} \sqrt{\frac{2 R g}{3}} - g t) = 0$ $\Rightarrow 12 (\sqrt{30} + 4 \sqrt{\frac{g}{R}} t - 6 \sqrt{6} \sqrt{\frac{r}{R}}) = (30 - 2 \sqrt{30} \sqrt{\frac{g}{R}} t - 9 {(\sqrt{\frac{g}{R}} t)}^{2} - 18 \frac{r}{R}) (\sqrt{30} + 9 \sqrt{\frac{g}{R}} t)$ $Φ_{m i n} = r^{2}$ $\Rightarrow {(\frac{\sqrt{5}}{3} + \frac{2 \sqrt{6}}{9} \sqrt{\frac{g}{R}} t - 2 \sqrt{\frac{r}{R}})}^{2} + {(\frac{5}{3} - \frac{\sqrt{30}}{9} \sqrt{\frac{g}{R}} t - \frac{1}{2} {(\sqrt{\frac{g}{R}} t)}^{2} - \frac{r}{R})}^{2} = {(\frac{r}{R})}^{2}$ $l e t δ = \sqrt{\frac{g}{R}} t, λ = \sqrt{\frac{r}{R}}$ $\Rightarrow 12 (\sqrt{30} + 4 δ - 6 \sqrt{6} λ) = (30 - 2 \sqrt{30} δ - 9 δ^{2} - 18 λ^{2}) (\sqrt{30} + 9 δ) . . . (i)$ $\Rightarrow 4 {(3 \sqrt{5} + 2 \sqrt{6} δ - 18 λ)}^{2} + {(30 - 2 \sqrt{30} δ - 9 δ^{2} - 18 λ^{2})}^{2} = 324 λ^{4} . . . (i i)$ $w e g e t f r o m (i) a n d (i i) :$ $λ \approx 0.53716$ $\Rightarrow r = λ^{2} R \approx 0.2885 R$
	Commented by mr W last updated on 29/Jun/20

	Commented by ajfour last updated on 29/Jun/20

	${h a v e n}^{'} t b e e n t h r o u g h a l l o f i t s i r, b u t$ $r e a l l y l o o k s s u p e r b s o l u t i o n, i w a n t$ $t o t r y o n m y o w n f o r a l i t t l e w h i l e . . .$
	Commented by ajfour last updated on 29/Jun/20

	$c a n y o u h e l p m e s i r, w i t h t h e e q u a t i o n$ $o f p a r a b o l a w i t h s h o w n a x e s . . ?$
	Commented by mr W last updated on 30/Jun/20

	Commented by ajfour last updated on 02/Jul/20
	$x=Rsin θ+(ucos θ)t y=R(1+cos θ)−(usin θ)t−((gt^2 )/2) As cos θ=(2/3) , sin θ=((√5)/3) , u^2 =((2Rg)/3) y=((5R)/3)−(((u(√5))/3))(((x−((R(√5))/3)))/((((2u)/3))))−(g/2)(((x−((R(√5))/3))^2 )/((((2u)/3))^2 )) y=((5R)/3)−((√5)/2)(x−((R(√5))/3))−((27)/(16R))(x−((R(√5))/3))^2 (dy/dx)=−((√5)/2)−((27)/(8R))(x−((R(√5))/3))=0 ⇒ x_0 =((R(√5))/3)−((8R)/(27))(((√5)/2)) = ((5(√5)R)/(27)) y_0 =((5R)/3)−((√5)/2)(((5(√5)R)/(27))−((9R(√5))/(27))) −((27)/(16R))(((80R^2 )/(27×27))) ⇒ y_0 =((5R)/3)+((10R)/(27))−((5R)/(27))=((50R)/(27)) < 2R Thus eq. of parabola is y=((50R)/(27))−((27)/(16R))(x−((5(√5)R)/(27)))^2 (dy/dx)=−((27)/(8R))(x−((5(√5)R)/(27))) say this parabola touches the smaller circle at P (h,k) and let center of smaller circle is C(2(√(Rr)), r); then h=2(√(Rr))+rcos 𝛃 k=r+rsin 𝛃 cot β=((27)/(8R))(h−((5(√5)R)/(27)))=((h−2(√(Rr)))/(k−r)) ⇒ ((27)/8)z=(((z+((5(√5))/(27)))−2(√s))/(((50)/(27))−((27)/(16))z^2 −s)) ......(i) k=((50R)/(27))−((27)/(16R))(h−((5(√5)R)/(27)))^2 = r+(r/((1+[((27)/(8R))(h−((5(√5)R)/(27)))]^2 ))^(1/) ) ⇒ ((50)/(27))−((27)/(16))z^2 =s+(s/((1+(((27)/(16))z)^2 ))^(1/) ) .....(ii) ⇒ s=(((((50)/(27))−((27)/(16))z^2 ))/(1+(1/((1+(((27z)/(16)))^2 ))^(1/) ))) ; Now ⇒ ((27)/8)z=(((z+((5(√5))/(27)))−2{(((((50)/(27))−((27)/(16))z^2 ))/(1+(1/((1+(((27z)/(16)))^2 ))^(1/) )))}^(1/2) )/(((50)/(27))−((27)/(16))z^2 −((((((50)/(27))−((27)/(16))z^2 ))/(1+(1/((1+(((27z)/(16)))^2 ))^(1/) )))))) ......(A) z is obtained from above eq. Then s=(r/R) = (((((50)/(27))−((27)/(16))z^2 ))/(1+(1/((1+(((27z)/(16)))^2 ))^(1/) ))) ■$
	$x = R \sin θ + (u \cos θ) t$ $y = R (1 + \cos θ) - (u \sin θ) t - \frac{{g t}^{2}}{2}$ $A s \cos θ = \frac{2}{3}, \sin θ = \frac{\sqrt{5}}{3}, u^{2} = \frac{2 R g}{3}$ $y = \frac{5 R}{3} - (\frac{u \sqrt{5}}{3}) \frac{(x - \frac{R \sqrt{5}}{3})}{(\frac{2 u}{3})} - \frac{g}{2} \frac{{(x - \frac{R \sqrt{5}}{3})}^{2}}{{(\frac{2 u}{3})}^{2}}$ $y = \frac{5 R}{3} - \frac{\sqrt{5}}{2} (x - \frac{R \sqrt{5}}{3}) - \frac{27}{16 R} {(x - \frac{R \sqrt{5}}{3})}^{2}$ $\frac{d y}{d x} = - \frac{\sqrt{5}}{2} - \frac{27}{8 R} (x - \frac{R \sqrt{5}}{3}) = 0$ $\Rightarrow x_{0} = \frac{R \sqrt{5}}{3} - \frac{8 R}{27} (\frac{\sqrt{5}}{2}) = \frac{5 \sqrt{5} R}{27}$ $y_{0} = \frac{5 R}{3} - \frac{\sqrt{5}}{2} (\frac{5 \sqrt{5} R}{27} - \frac{9 R \sqrt{5}}{27})$ $- \frac{27}{16 R} (\frac{80 R^{2}}{27 \times 27})$ $\Rightarrow y_{0} = \frac{5 R}{3} + \frac{10 R}{27} - \frac{5 R}{27} = \frac{50 R}{27} < 2 R$ $T h u s e q . o f p a r a b o l a i s$ $y = \frac{50 R}{27} - \frac{27}{16 R} {(x - \frac{5 \sqrt{5} R}{27})}^{2}$ $\frac{d y}{d x} = - \frac{27}{8 R} (x - \frac{5 \sqrt{5} R}{27})$ $s a y t h i s p a r a b o l a t o u c h e s t h e s m a l l e r$ $c i r c l e a t P (h, k) a n d l e t c e n t e r o f$ $s m a l l e r c i r c l e i s C (2 \sqrt{R r}, r); t h e n$ $h = 2 \sqrt{R r} + r \cos β$ $k = r + r \sin β$ $\cot β = \frac{27}{8 R} (h - \frac{5 \sqrt{5} R}{27}) = \frac{h - 2 \sqrt{R r}}{k - r}$ $\Rightarrow \frac{27}{8} z = \frac{(z + \frac{5 \sqrt{5}}{27}) - 2 \sqrt{s}}{\frac{50}{27} - \frac{27}{16} z^{2} - s} . . . . . . (i)$ $k = \frac{50 R}{27} - \frac{27}{16 R} {(h - \frac{5 \sqrt{5} R}{27})}^{2}$ $= r + \frac{r}{\sqrt[]{1 + {[\frac{27}{8 R} (h - \frac{5 \sqrt{5} R}{27})]}^{2}}}$ $\Rightarrow \frac{50}{27} - \frac{27}{16} z^{2} = s + \frac{s}{\sqrt[]{1 + {(\frac{27}{16} z)}^{2}}} . . . . . (i i)$ $\Rightarrow s = \frac{(\frac{50}{27} - \frac{27}{16} z^{2})}{1 + \frac{1}{\sqrt[]{1 + {(\frac{27 z}{16})}^{2}}}}; N o w$ $\Rightarrow \frac{27}{8} z = \frac{(z + \frac{5 \sqrt{5}}{27}) - 2 {\frac{(\frac{50}{27} - \frac{27}{16} z^{2})}{1 + \frac{1}{\sqrt[]{1 + {(\frac{27 z}{16})}^{2}}}}}^{1 / 2}}{\frac{50}{27} - \frac{27}{16} z^{2} - (\frac{(\frac{50}{27} - \frac{27}{16} z^{2})}{1 + \frac{1}{\sqrt[]{1 + {(\frac{27 z}{16})}^{2}}}})} . . . . . . (A)$ $z i s o b t a i n e d f r o m a b o v e e q .$ $T h e n s = \frac{r}{R} = \frac{(\frac{50}{27} - \frac{27}{16} z^{2})}{1 + \frac{1}{\sqrt[]{1 + {(\frac{27 z}{16})}^{2}}}} ◼$
	Commented by ajfour last updated on 02/Jul/20

	Commented by mr W last updated on 02/Jul/20

	$b e a u t i f u l l y s o l v e d!$
Terms of Service
Privacy Policy
Contact: info@tinkutara.com

Question and Answers Forum