Given a=^3 (√(7+(√(50)))),b=^3 (√(7−(√(50)))).Prove that a^7 +b^7 is an even number.


Question and Answers Forum

All Questions Topic List
Algebra Questions
Previous in All Question Next in All Question
Previous in Algebra Next in Algebra
Question Number 101052 by 1549442205 last updated on 30/Jun/20

$Given a =^{3} \sqrt{7 + \sqrt{50}}, b =^{3} \sqrt{7 - \sqrt{50}} . Prove$ $that a^{7} + b^{7} is an even number .$
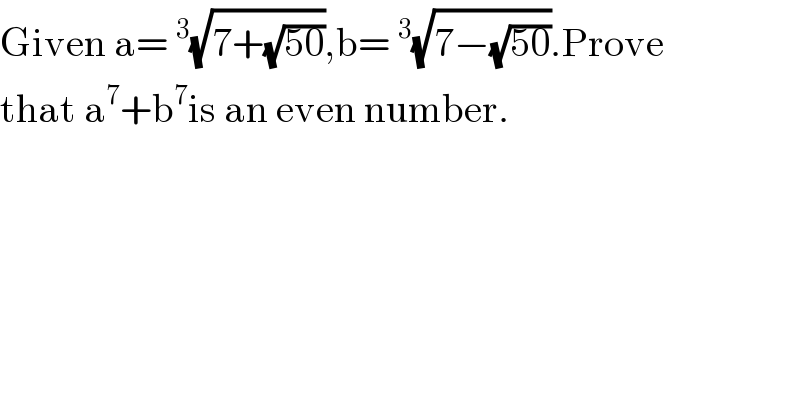
	Answered by MJS last updated on 30/Jun/20

	$a = \sqrt[3]{7 + 5 \sqrt{2}} = 1 + \sqrt{2}$ $b = \sqrt[3]{7 - 5 \sqrt{2}} = 1 - \sqrt{2}$ $we can show that {(p + q \sqrt{r})}^{n} + {(p - q \sqrt{r})}^{n}$ $with p, q \in Z and r, n \in N must always be$ $an even number$ $α = p; β = q \sqrt{r}$ ${(α + β)}^{n} + {(α - β)}^{n} leads to cancelling out$ $some terms and doubling the remaining$ $terms \Rightarrow {(α + β)}^{n} + {(α - β)}^{n} = 2 k$ $I {haven}^{'} t got the time to type the proof but$ ${it}^{'} s obvious$
	Commented by 1549442205 last updated on 01/Jul/20

	$Thank you sir a lot . You are welcome .$
	Commented by 1549442205 last updated on 30/Jun/20

	$Thank you sir a lot . Please, I get permission$ $to submit a way as follows :$ $i) If n is odd then A = {(p + q \sqrt{r})}^{2 k + 1} + {(p - q \sqrt{r})}^{2 k + 1}$ $= 2 p [{(p + q \sqrt{r})}^{2 k} - {(p + q)}^{2 k - 1} (p - q \sqrt{r}) +$ $. . . {(p - q \sqrt{r})}^{2 k}] \Rightarrow A is even$ $ii) If n = 2 k then A = {[{(p + q \sqrt{r})}^{k} + {(p - q \sqrt{r})}^{k}]}^{2}$ $+ for k = 1 \Rightarrow A = {2 p}^{2} + {2 q}^{2} r \Rightarrow A is even$ $+ Suppose A is even \forall n ⩽ 2 k i . e$ $A_{n} = {(p + q \sqrt{r})}^{n} + {(p - q \sqrt{r})}^{n} is even \forall n ⩽ 2 k$ $Then A_{2 n + 2} = {[{(p + q \sqrt{r})}^{k + 1} + {(p - q \sqrt{r})}^{k + 1}]}^{2}$ $- 2 {(p^{2} - q^{2} r)}^{k + 1} = {(2 M)}^{2} - 2 {(p^{2} - q^{2} r)}^{k + 1} = 2 Q$ $which shows that^{″} A is {even}^{″} true for \forall n = 2 k$ $F rom i) and ii) follows A is even \forall n$ $Sir, is it all right ? Thank you sir!$
	Commented by MJS last updated on 30/Jun/20

	$looks right to me$
	Answered by 1549442205 last updated on 03/Jul/20

	$ab =^{3} \sqrt{(7 + \sqrt{50}) (7 - \sqrt{50})} = - 1 . Hence,$ $a^{3} + b^{3} = 14 = {(a + b)}^{3} - 3 ab (a + b)$ $= {(a + b)}^{3} + 3 (a + b) \Rightarrow {(a + b)}^{3} + 3 (a + b) - 14 = 0$ $\Leftrightarrow (a + b - 2) [{(a + b)}^{2} + 2 (a + b) + 7] = 0$ $\Rightarrow a + b = 2 . From$ $a^{7} + b^{7} = (a + b) (a^{6} - a^{5} b + a^{4} b^{2} - a^{3} b^{3} + a^{2} b^{4} - {ab}^{5} + b^{6})$ $= 2 (a^{6} - a^{5} b + a^{4} b^{2} - a^{3} b^{3} + a^{2} b^{4} - {ab}^{5} + b^{6})$ $which shows that a^{7} + b^{7} is odd number (q . e . d)$ $other way :$ $a^{4} + b^{4} = {(a^{2} + b^{2})}^{2} - 2 a^{2} b^{2} = {[{(a + b)}^{2} - 2 ab]}^{2} - 2 a^{2} b^{2}$ $= {(2^{2} - 2 (- 1))}^{2} - 2 \times 1 = 6^{2} - 2 = 34$ $a^{7} + b^{7} = (a^{3} + b^{3}) (a^{4} + b^{4}) - a^{3} b^{3} (a + b)$ $= 14 \times 34 - {(- 1)}^{3} \times 2 = 478, so a^{7} + b^{7}$ $is a evev number (q . e . d)$
Terms of Service
Privacy Policy
Contact: info@tinkutara.com

Question and Answers Forum