Question and Answers Forum

All Questions      Topic List

Geometry Questions

Previous in All Question      Next in All Question      

Previous in Geometry      Next in Geometry      

Question Number 102769 by ajfour last updated on 11/Jul/20

Commented by bramlex last updated on 11/Jul/20

Commented by bramlex last updated on 11/Jul/20

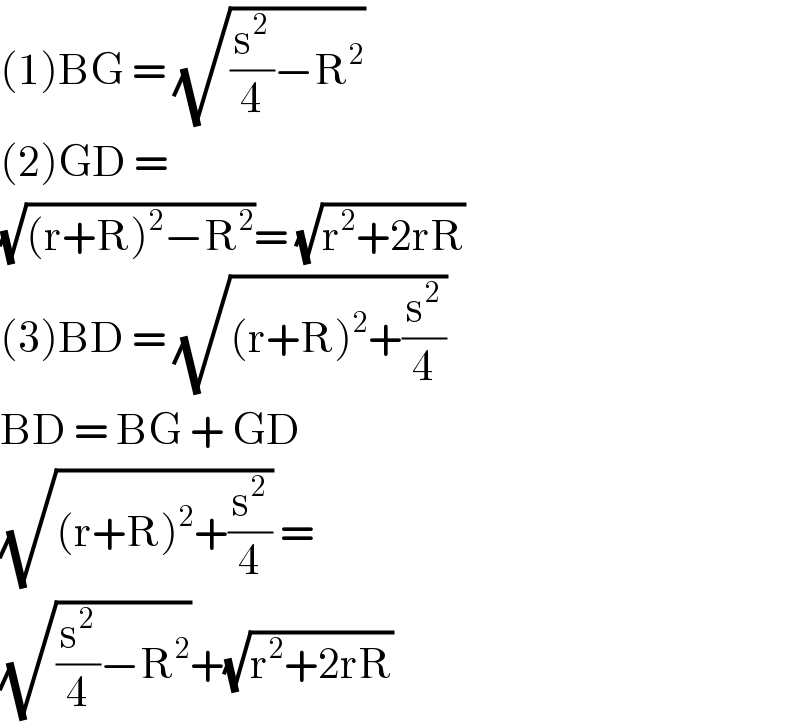
(1)BG = (√((s^2 /4)−R^2 )) (2)GD = (√((r+R)^2 −R^2 ))= (√(r^2 +2rR)) (3)BD = (√((r+R)^2 +(s^2 /4))) BD = BG + GD (√((r+R)^2 +(s^2 /4))) = (√((s^2 /4)−R^2 ))+(√(r^2 +2rR))

$$\left(\mathrm{1}\right)\mathrm{BG}\:=\:\sqrt{\frac{\mathrm{s}^{\mathrm{2}} }{\mathrm{4}}−\mathrm{R}^{\mathrm{2}} } \\ $$$$\left(\mathrm{2}\right)\mathrm{GD}\:= \\ $$$$\sqrt{\left(\mathrm{r}+\mathrm{R}\right)^{\mathrm{2}} −\mathrm{R}^{\mathrm{2}} }=\:\sqrt{\mathrm{r}^{\mathrm{2}} +\mathrm{2rR}} \\ $$$$\left(\mathrm{3}\right)\mathrm{BD}\:=\:\sqrt{\left(\mathrm{r}+\mathrm{R}\right)^{\mathrm{2}} +\frac{\mathrm{s}^{\mathrm{2}} }{\mathrm{4}}} \\ $$$$\mathrm{BD}\:=\:\mathrm{BG}\:+\:\mathrm{GD} \\ $$$$\sqrt{\left(\mathrm{r}+\mathrm{R}\right)^{\mathrm{2}} +\frac{\mathrm{s}^{\mathrm{2}} }{\mathrm{4}}}\:= \\ $$$$\sqrt{\frac{\mathrm{s}^{\mathrm{2}} }{\mathrm{4}}−\mathrm{R}^{\mathrm{2}} }+\sqrt{\mathrm{r}^{\mathrm{2}} +\mathrm{2rR}}\: \\ $$

Commented by ajfour last updated on 11/Jul/20

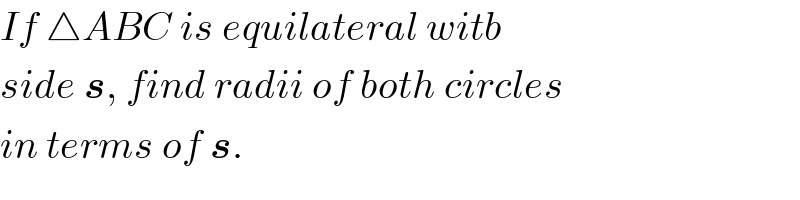
If △ABC is equilateral witb side s, find radii of both circles in terms of s.

$${If}\:\bigtriangleup{ABC}\:{is}\:{equilateral}\:{witb} \\ $$$${side}\:\boldsymbol{{s}},\:{find}\:{radii}\:{of}\:{both}\:{circles} \\ $$$${in}\:{terms}\:{of}\:\boldsymbol{{s}}. \\ $$

Commented by bramlex last updated on 11/Jul/20

Commented by bramlex last updated on 11/Jul/20

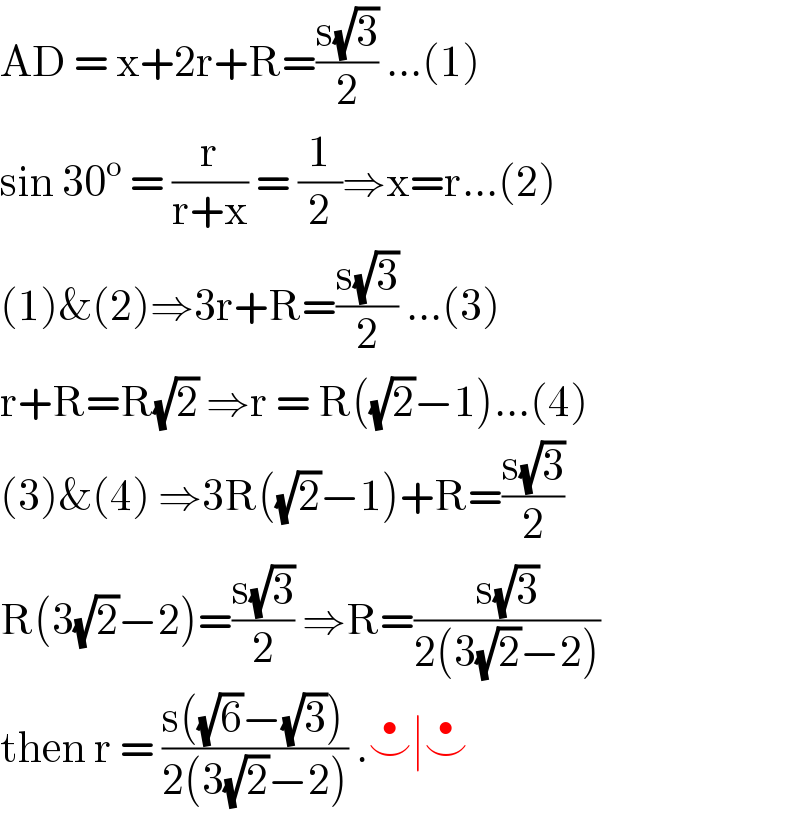
AD = x+2r+R=((s(√3))/2) ...(1) sin 30^o = (r/(r+x)) = (1/2)⇒x=r...(2) (1)&(2)⇒3r+R=((s(√3))/2) ...(3) r+R=R(√2) ⇒r = R((√2)−1)...(4) (3)&(4) ⇒3R((√2)−1)+R=((s(√3))/2) R(3(√2)−2)=((s(√3))/2) ⇒R=((s(√3))/(2(3(√2)−2))) then r = ((s((√6)−(√3)))/(2(3(√2)−2))) .⌣^• ∣⌣^•

$$\mathrm{AD}\:=\:\mathrm{x}+\mathrm{2r}+\mathrm{R}=\frac{\mathrm{s}\sqrt{\mathrm{3}}}{\mathrm{2}}\:...\left(\mathrm{1}\right) \\ $$$$\mathrm{sin}\:\mathrm{30}^{\mathrm{o}} \:=\:\frac{\mathrm{r}}{\mathrm{r}+\mathrm{x}}\:=\:\frac{\mathrm{1}}{\mathrm{2}}\Rightarrow\mathrm{x}=\mathrm{r}...\left(\mathrm{2}\right) \\ $$$$\left(\mathrm{1}\right)\&\left(\mathrm{2}\right)\Rightarrow\mathrm{3r}+\mathrm{R}=\frac{\mathrm{s}\sqrt{\mathrm{3}}}{\mathrm{2}}\:...\left(\mathrm{3}\right) \\ $$$$\mathrm{r}+\mathrm{R}=\mathrm{R}\sqrt{\mathrm{2}}\:\Rightarrow\mathrm{r}\:=\:\mathrm{R}\left(\sqrt{\mathrm{2}}−\mathrm{1}\right)...\left(\mathrm{4}\right) \\ $$$$\left(\mathrm{3}\right)\&\left(\mathrm{4}\right)\:\Rightarrow\mathrm{3R}\left(\sqrt{\mathrm{2}}−\mathrm{1}\right)+\mathrm{R}=\frac{\mathrm{s}\sqrt{\mathrm{3}}}{\mathrm{2}} \\ $$$$\mathrm{R}\left(\mathrm{3}\sqrt{\mathrm{2}}−\mathrm{2}\right)=\frac{\mathrm{s}\sqrt{\mathrm{3}}}{\mathrm{2}}\:\Rightarrow\mathrm{R}=\frac{\mathrm{s}\sqrt{\mathrm{3}}}{\mathrm{2}\left(\mathrm{3}\sqrt{\mathrm{2}}−\mathrm{2}\right)} \\ $$$$\mathrm{then}\:\mathrm{r}\:=\:\frac{\mathrm{s}\left(\sqrt{\mathrm{6}}−\sqrt{\mathrm{3}}\right)}{\mathrm{2}\left(\mathrm{3}\sqrt{\mathrm{2}}−\mathrm{2}\right)}\:.\overset{\bullet} {\smile}\mid\overset{\bullet} {\smile}\: \\ $$

Commented by ajfour last updated on 11/Jul/20

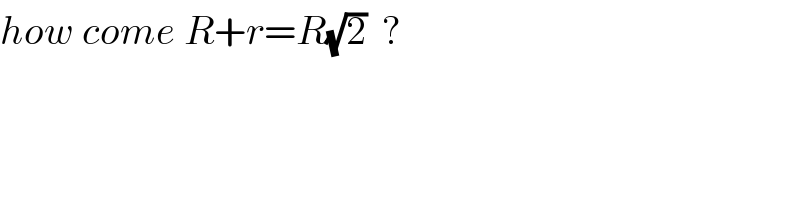
how come R+r=R(√2) ?

$${how}\:{come}\:{R}+{r}={R}\sqrt{\mathrm{2}}\:\:? \\ $$

Commented by bramlex last updated on 11/Jul/20

Commented by ajfour last updated on 11/Jul/20

the quadrilateral isn′t a parallelogram, wrong..

$${the}\:{quadrilateral}\:{isn}'{t}\:{a}\: \\ $$$${parallelogram},\:\:{wrong}.. \\ $$

Commented by bramlex last updated on 11/Jul/20

no.

$$\mathrm{no}. \\ $$

Answered by mr W last updated on 11/Jul/20

Commented by mr W last updated on 11/Jul/20

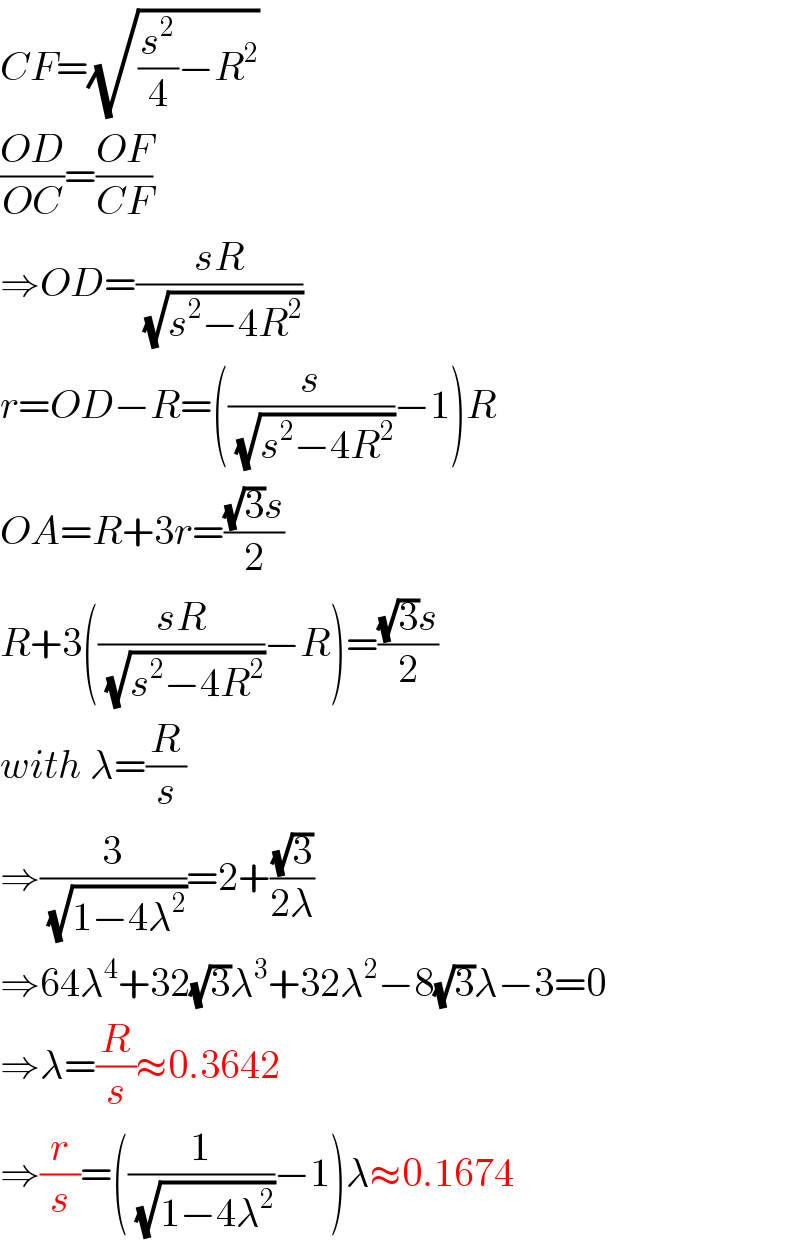
CF=(√((s^2 /4)−R^2 )) ((OD)/(OC))=((OF)/(CF)) ⇒OD=((sR)/(√(s^2 −4R^2 ))) r=OD−R=((s/(√(s^2 −4R^2 )))−1)R OA=R+3r=(((√3)s)/2) R+3(((sR)/(√(s^2 −4R^2 )))−R)=(((√3)s)/2) with λ=(R/s) ⇒(3/(√(1−4λ^2 )))=2+((√3)/(2λ)) ⇒64λ^4 +32(√3)λ^3 +32λ^2 −8(√3)λ−3=0 ⇒λ=(R/s)≈0.3642 ⇒(r/s)=((1/(√(1−4λ^2 )))−1)λ≈0.1674

$${CF}=\sqrt{\frac{{s}^{\mathrm{2}} }{\mathrm{4}}−{R}^{\mathrm{2}} } \\ $$$$\frac{{OD}}{{OC}}=\frac{{OF}}{{CF}} \\ $$$$\Rightarrow{OD}=\frac{{sR}}{\sqrt{{s}^{\mathrm{2}} −\mathrm{4}{R}^{\mathrm{2}} }} \\ $$$${r}={OD}−{R}=\left(\frac{{s}}{\sqrt{{s}^{\mathrm{2}} −\mathrm{4}{R}^{\mathrm{2}} }}−\mathrm{1}\right){R} \\ $$$${OA}={R}+\mathrm{3}{r}=\frac{\sqrt{\mathrm{3}}{s}}{\mathrm{2}} \\ $$$${R}+\mathrm{3}\left(\frac{{sR}}{\sqrt{{s}^{\mathrm{2}} −\mathrm{4}{R}^{\mathrm{2}} }}−{R}\right)=\frac{\sqrt{\mathrm{3}}{s}}{\mathrm{2}} \\ $$$${with}\:\lambda=\frac{{R}}{{s}} \\ $$$$\Rightarrow\frac{\mathrm{3}}{\sqrt{\mathrm{1}−\mathrm{4}\lambda^{\mathrm{2}} }}=\mathrm{2}+\frac{\sqrt{\mathrm{3}}}{\mathrm{2}\lambda} \\ $$$$\Rightarrow\mathrm{64}\lambda^{\mathrm{4}} +\mathrm{32}\sqrt{\mathrm{3}}\lambda^{\mathrm{3}} +\mathrm{32}\lambda^{\mathrm{2}} −\mathrm{8}\sqrt{\mathrm{3}}\lambda−\mathrm{3}=\mathrm{0} \\ $$$$\Rightarrow\lambda=\frac{{R}}{{s}}\approx\mathrm{0}.\mathrm{3642} \\ $$$$\Rightarrow\frac{{r}}{{s}}=\left(\frac{\mathrm{1}}{\sqrt{\mathrm{1}−\mathrm{4}\lambda^{\mathrm{2}} }}−\mathrm{1}\right)\lambda\approx\mathrm{0}.\mathrm{1674} \\ $$

Commented by mr W last updated on 11/Jul/20

Commented by ajfour last updated on 11/Jul/20

Very Nice solution Sir; Thanks!

$${Very}\:{Nice}\:{solution}\:{Sir};\:{Thanks}! \\ $$

Answered by 1549442205 last updated on 11/Jul/20

Commented by 1549442205 last updated on 11/Jul/20

Since DKE^(�) =30°,KD=((DE)/(sinDKE^(�) ))=(r/(sin30°)) ⇒KD=2r.We have KC=KAcos30°=((s(√3))/2) ,so KD=KC−CD.Hence, 2r=((s(√3))/2)−(R+r)⇔r=((s(√3)−2R)/6)(1) On ther other hands, AD^2 =(R+r)^2 +(s^2 /4) KE=r(√3) and AE=s−KE=s−r(√3) , AE^2 +DE^2 =AD^2 =DC^2 +AC^2 ,so (s−r(√3))^2 +r^2 =(R+r)^2 +(s^2 /4) ⇔R^2 +2Rr−3r^2 +2sr(√3)−((3s^2 )/4)=0(due to (1)) ⇔R^2 +((s(√3)−2R)/3)(R+s(√3))−((3s^2 −4Rs(√3)+4R^2 )/3)−((3s^2 )/4)=0 4R^2 −12Rs(√3) +9s^2 =0 .Δ′=108s^2 −36s^2 =72s^2 R=((6s(√3)−6s(√2))/4)=((3s((√3)−(√2)))/2).Replace into (1) we get r=((s(√3)−3s((√3)−(√2)))/6)=(((3(√2)−2(√3))s)/6) Thus, { (((R/s)=((3((√3)−(√2)))/2)≈0.47675586)),(((r/s)=((3(√2)−2(√3))/6)≈0.12975512)) :}

$$\mathrm{Since}\:\widehat {\mathrm{DKE}}=\mathrm{30}°,\mathrm{KD}=\frac{\mathrm{DE}}{\mathrm{sin}\widehat {\mathrm{DKE}}}=\frac{\mathrm{r}}{\mathrm{sin30}°} \\ $$$$\Rightarrow\mathrm{KD}=\mathrm{2r}.\mathrm{We}\:\mathrm{have}\:\mathrm{KC}=\mathrm{KAcos30}°=\frac{\mathrm{s}\sqrt{\mathrm{3}}}{\mathrm{2}} \\ $$$$,\mathrm{so}\:\mathrm{KD}=\mathrm{KC}−\mathrm{CD}.\mathrm{Hence}, \\ $$$$\mathrm{2r}=\frac{\mathrm{s}\sqrt{\mathrm{3}}}{\mathrm{2}}−\left(\mathrm{R}+\mathrm{r}\right)\Leftrightarrow\mathrm{r}=\frac{\mathrm{s}\sqrt{\mathrm{3}}−\mathrm{2R}}{\mathrm{6}}\left(\mathrm{1}\right) \\ $$$$\mathrm{On}\:\mathrm{ther}\:\mathrm{other}\:\mathrm{hands}, \\ $$$$\mathrm{AD}^{\mathrm{2}} =\left(\mathrm{R}+\mathrm{r}\right)^{\mathrm{2}} +\frac{\mathrm{s}^{\mathrm{2}} }{\mathrm{4}} \\ $$$$\mathrm{KE}=\mathrm{r}\sqrt{\mathrm{3}}\:\mathrm{and}\:\mathrm{AE}=\mathrm{s}−\mathrm{KE}=\mathrm{s}−\mathrm{r}\sqrt{\mathrm{3}}\:, \\ $$$$\mathrm{AE}^{\mathrm{2}} +\mathrm{DE}^{\mathrm{2}} =\mathrm{AD}^{\mathrm{2}} =\mathrm{DC}^{\mathrm{2}} +\mathrm{AC}^{\mathrm{2}} ,\mathrm{so} \\ $$$$\left(\mathrm{s}−\mathrm{r}\sqrt{\mathrm{3}}\right)^{\mathrm{2}} +\mathrm{r}^{\mathrm{2}} =\left(\mathrm{R}+\mathrm{r}\right)^{\mathrm{2}} +\frac{\mathrm{s}^{\mathrm{2}} }{\mathrm{4}} \\ $$$$\Leftrightarrow\mathrm{R}^{\mathrm{2}} +\mathrm{2Rr}−\mathrm{3r}^{\mathrm{2}} +\mathrm{2sr}\sqrt{\mathrm{3}}−\frac{\mathrm{3s}^{\mathrm{2}} }{\mathrm{4}}=\mathrm{0}\left(\mathrm{due}\:\mathrm{to}\:\left(\mathrm{1}\right)\right) \\ $$$$\Leftrightarrow\mathrm{R}^{\mathrm{2}} +\frac{\mathrm{s}\sqrt{\mathrm{3}}−\mathrm{2R}}{\mathrm{3}}\left(\mathrm{R}+\mathrm{s}\sqrt{\mathrm{3}}\right)−\frac{\mathrm{3s}^{\mathrm{2}} −\mathrm{4Rs}\sqrt{\mathrm{3}}+\mathrm{4R}^{\mathrm{2}} }{\mathrm{3}}−\frac{\mathrm{3s}^{\mathrm{2}} }{\mathrm{4}}=\mathrm{0} \\ $$$$\mathrm{4R}^{\mathrm{2}} −\mathrm{12Rs}\sqrt{\mathrm{3}}\:+\mathrm{9s}^{\mathrm{2}} =\mathrm{0}\:\:.\Delta'=\mathrm{108s}^{\mathrm{2}} −\mathrm{36s}^{\mathrm{2}} =\mathrm{72s}^{\mathrm{2}} \\ $$$$\mathrm{R}=\frac{\mathrm{6}\boldsymbol{\mathrm{s}}\sqrt{\mathrm{3}}−\mathrm{6}\boldsymbol{\mathrm{s}}\sqrt{\mathrm{2}}}{\mathrm{4}}=\frac{\mathrm{3}\boldsymbol{\mathrm{s}}\left(\sqrt{\mathrm{3}}−\sqrt{\mathrm{2}}\right)}{\mathrm{2}}.\mathrm{Replace} \\ $$$$\mathrm{into}\:\left(\mathrm{1}\right)\:\mathrm{we}\:\mathrm{get} \\ $$$$\:\boldsymbol{\mathrm{r}}=\frac{\boldsymbol{\mathrm{s}}\sqrt{\mathrm{3}}−\mathrm{3}\boldsymbol{\mathrm{s}}\left(\sqrt{\mathrm{3}}−\sqrt{\mathrm{2}}\right)}{\mathrm{6}}=\frac{\left(\mathrm{3}\sqrt{\mathrm{2}}−\mathrm{2}\sqrt{\mathrm{3}}\right)\boldsymbol{\mathrm{s}}}{\mathrm{6}} \\ $$$$\mathrm{Thus},\begin{cases}{\frac{\mathrm{R}}{\mathrm{s}}=\frac{\mathrm{3}\left(\sqrt{\mathrm{3}}−\sqrt{\mathrm{2}}\right)}{\mathrm{2}}\approx\mathrm{0}.\mathrm{47675586}}\\{\frac{\mathrm{r}}{\mathrm{s}}=\frac{\mathrm{3}\sqrt{\mathrm{2}}−\mathrm{2}\sqrt{\mathrm{3}}}{\mathrm{6}}\approx\mathrm{0}.\mathrm{12975512}}\end{cases} \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com