Question and Answers Forum

All Questions      Topic List

Mechanics Questions

Previous in All Question      Next in All Question      

Previous in Mechanics      Next in Mechanics      

Question Number 102978 by ajfour last updated on 12/Jul/20

Commented by ajfour last updated on 12/Jul/20

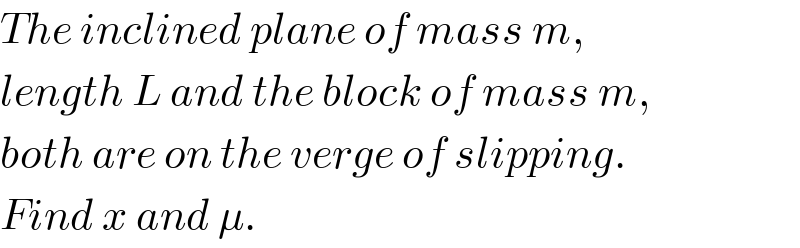
The inclined plane of mass m, length L and the block of mass m, both are on the verge of slipping. Find x and μ.

$${The}\:{inclined}\:{plane}\:{of}\:{mass}\:{m}, \\ $$$${length}\:{L}\:{and}\:{the}\:{block}\:{of}\:{mass}\:{m}, \\ $$$${both}\:{are}\:{on}\:{the}\:{verge}\:{of}\:{slipping}. \\ $$$${Find}\:{x}\:{and}\:\mu. \\ $$

Answered by mr W last updated on 12/Jul/20

Commented by mr W last updated on 12/Jul/20

x=L sin θ C=center of total mass of both objects M=2m BC=p=((mx+m×(L/2))/(2m))=((L(2 sin θ+1))/4) CA=q=L−p=((L(3−2 sin θ))/4) tan φ=μ such that the block m doesn′t slip, tan θ=μ ⇒φ=θ α=(π/2)−θ−φ=(π/2)−2θ β=φ+θ=2θ ((SC)/(sin β))=((BC)/(sin ((π/2)−φ)))=(p/(cos θ)) ⇒SC=((p sin β)/(cos θ))=((L(2 sin θ+1) sin 2θ)/(4 cos θ)) =((L(2 sin θ+1) sin θ)/2) ((SC)/(sin α))=((CA)/(sin φ))=(q/(sin θ)) ⇒SC=((q sin α)/(sin θ))=((L(3−2 sin θ) cos 2θ)/(4 sin θ)) ((L(3−2 sin θ) cos 2θ)/(4 sin θ))=((L(2 sin θ+1) sin θ)/2) ⇒8 sin^2 θ+2 sin θ−3=0 ⇒(2 sin θ−1)(4 sin θ+3)=0 ⇒sin θ=(x/L)=(1/2) or −(3/4) (rejected) ⇒θ=30° ⇒μ=tan θ=(1/(√3))

$${x}={L}\:\mathrm{sin}\:\theta \\ $$$${C}={center}\:{of}\:{total}\:{mass}\:{of}\:{both}\:{objects} \\ $$$${M}=\mathrm{2}{m} \\ $$$${BC}={p}=\frac{{mx}+{m}×\frac{{L}}{\mathrm{2}}}{\mathrm{2}{m}}=\frac{{L}\left(\mathrm{2}\:\mathrm{sin}\:\theta+\mathrm{1}\right)}{\mathrm{4}} \\ $$$${CA}={q}={L}−{p}=\frac{{L}\left(\mathrm{3}−\mathrm{2}\:\mathrm{sin}\:\theta\right)}{\mathrm{4}} \\ $$$$\mathrm{tan}\:\phi=\mu \\ $$$${such}\:{that}\:{the}\:{block}\:{m}\:{doesn}'{t}\:{slip}, \\ $$$$\mathrm{tan}\:\theta=\mu \\ $$$$\Rightarrow\phi=\theta \\ $$$$\alpha=\frac{\pi}{\mathrm{2}}−\theta−\phi=\frac{\pi}{\mathrm{2}}−\mathrm{2}\theta \\ $$$$\beta=\phi+\theta=\mathrm{2}\theta \\ $$$$\frac{{SC}}{\mathrm{sin}\:\beta}=\frac{{BC}}{\mathrm{sin}\:\left(\frac{\pi}{\mathrm{2}}−\phi\right)}=\frac{{p}}{\mathrm{cos}\:\theta} \\ $$$$\Rightarrow{SC}=\frac{{p}\:\mathrm{sin}\:\beta}{\mathrm{cos}\:\theta}=\frac{{L}\left(\mathrm{2}\:\mathrm{sin}\:\theta+\mathrm{1}\right)\:\mathrm{sin}\:\mathrm{2}\theta}{\mathrm{4}\:\mathrm{cos}\:\theta} \\ $$$$=\frac{{L}\left(\mathrm{2}\:\mathrm{sin}\:\theta+\mathrm{1}\right)\:\mathrm{sin}\:\theta}{\mathrm{2}} \\ $$$$\frac{{SC}}{\mathrm{sin}\:\alpha}=\frac{{CA}}{\mathrm{sin}\:\phi}=\frac{{q}}{\mathrm{sin}\:\theta} \\ $$$$\Rightarrow{SC}=\frac{{q}\:\mathrm{sin}\:\alpha}{\mathrm{sin}\:\theta}=\frac{{L}\left(\mathrm{3}−\mathrm{2}\:\mathrm{sin}\:\theta\right)\:\mathrm{cos}\:\mathrm{2}\theta}{\mathrm{4}\:\mathrm{sin}\:\theta} \\ $$$$ \\ $$$$\frac{{L}\left(\mathrm{3}−\mathrm{2}\:\mathrm{sin}\:\theta\right)\:\mathrm{cos}\:\mathrm{2}\theta}{\mathrm{4}\:\mathrm{sin}\:\theta}=\frac{{L}\left(\mathrm{2}\:\mathrm{sin}\:\theta+\mathrm{1}\right)\:\mathrm{sin}\:\theta}{\mathrm{2}} \\ $$$$\Rightarrow\mathrm{8}\:\mathrm{sin}^{\mathrm{2}} \:\theta+\mathrm{2}\:\mathrm{sin}\:\theta−\mathrm{3}=\mathrm{0} \\ $$$$\Rightarrow\left(\mathrm{2}\:\mathrm{sin}\:\theta−\mathrm{1}\right)\left(\mathrm{4}\:\mathrm{sin}\:\theta+\mathrm{3}\right)=\mathrm{0} \\ $$$$\Rightarrow\mathrm{sin}\:\theta=\frac{{x}}{{L}}=\frac{\mathrm{1}}{\mathrm{2}}\:{or}\:−\frac{\mathrm{3}}{\mathrm{4}}\:\left({rejected}\right) \\ $$$$\Rightarrow\theta=\mathrm{30}° \\ $$$$\Rightarrow\mu=\mathrm{tan}\:\theta=\frac{\mathrm{1}}{\sqrt{\mathrm{3}}} \\ $$

Commented by mr W last updated on 12/Jul/20

if block and plane have different masses, say mass of block is λm, and mass of plane is m, then M=(1+λ)m BC=p=((λmx+m×(L/2))/((λ+1)m))=((L(2λ sin θ+1))/(2(λ+1))) CA=q=L−p=((L[1+2λ(1−sin θ)])/(2(λ+1))) ⇒SC=((p sin β)/(cos θ))=((L(2λ sin θ+1) sin θ)/((λ+1))) ⇒SC=((q sin α)/(sin θ))=((L[1+2λ(1−sin θ)]cos 2θ)/(2(λ+1) sin θ)) ((L[1+2λ(1−sin θ)]cos 2θ)/(2(λ+1) sin θ))=((L(2λ sin θ+1) sin θ)/((λ+1))) [1+2λ(1−sin θ)](1−2 sin^2 θ)=2(2λ sin θ+1) sin^2 with t=sin θ 4(λ+1)t^2 +2λt−(2λ+1)=0 t=((−2λ+(√(4λ^2 +16(λ+1)(2λ+1))))/(8(λ+1))) t=((−λ+3λ+2)/(4(λ+1)))=(1/2)=sin θ that means the solution is the same even when both objects have different masses.

$${if}\:{block}\:{and}\:{plane}\:{have}\:{different} \\ $$$${masses},\:{say}\:{mass}\:{of}\:{block}\:{is}\:\lambda{m},\:{and} \\ $$$${mass}\:{of}\:{plane}\:{is}\:{m},\:{then} \\ $$$${M}=\left(\mathrm{1}+\lambda\right){m} \\ $$$${BC}={p}=\frac{\lambda{mx}+{m}×\frac{{L}}{\mathrm{2}}}{\left(\lambda+\mathrm{1}\right){m}}=\frac{{L}\left(\mathrm{2}\lambda\:\mathrm{sin}\:\theta+\mathrm{1}\right)}{\mathrm{2}\left(\lambda+\mathrm{1}\right)} \\ $$$${CA}={q}={L}−{p}=\frac{{L}\left[\mathrm{1}+\mathrm{2}\lambda\left(\mathrm{1}−\mathrm{sin}\:\theta\right)\right]}{\mathrm{2}\left(\lambda+\mathrm{1}\right)} \\ $$$$\Rightarrow{SC}=\frac{{p}\:\mathrm{sin}\:\beta}{\mathrm{cos}\:\theta}=\frac{{L}\left(\mathrm{2}\lambda\:\mathrm{sin}\:\theta+\mathrm{1}\right)\:\mathrm{sin}\:\theta}{\left(\lambda+\mathrm{1}\right)} \\ $$$$\Rightarrow{SC}=\frac{{q}\:\mathrm{sin}\:\alpha}{\mathrm{sin}\:\theta}=\frac{{L}\left[\mathrm{1}+\mathrm{2}\lambda\left(\mathrm{1}−\mathrm{sin}\:\theta\right)\right]\mathrm{cos}\:\mathrm{2}\theta}{\mathrm{2}\left(\lambda+\mathrm{1}\right)\:\mathrm{sin}\:\theta} \\ $$$$\frac{{L}\left[\mathrm{1}+\mathrm{2}\lambda\left(\mathrm{1}−\mathrm{sin}\:\theta\right)\right]\mathrm{cos}\:\mathrm{2}\theta}{\mathrm{2}\left(\lambda+\mathrm{1}\right)\:\mathrm{sin}\:\theta}=\frac{{L}\left(\mathrm{2}\lambda\:\mathrm{sin}\:\theta+\mathrm{1}\right)\:\mathrm{sin}\:\theta}{\left(\lambda+\mathrm{1}\right)} \\ $$$$\left[\mathrm{1}+\mathrm{2}\lambda\left(\mathrm{1}−\mathrm{sin}\:\theta\right)\right]\left(\mathrm{1}−\mathrm{2}\:\mathrm{sin}^{\mathrm{2}} \:\theta\right)=\mathrm{2}\left(\mathrm{2}\lambda\:\mathrm{sin}\:\theta+\mathrm{1}\right)\:\mathrm{sin}^{\mathrm{2}} \\ $$$${with}\:{t}=\mathrm{sin}\:\theta \\ $$$$\mathrm{4}\left(\lambda+\mathrm{1}\right){t}^{\mathrm{2}} +\mathrm{2}\lambda{t}−\left(\mathrm{2}\lambda+\mathrm{1}\right)=\mathrm{0} \\ $$$${t}=\frac{−\mathrm{2}\lambda+\sqrt{\mathrm{4}\lambda^{\mathrm{2}} +\mathrm{16}\left(\lambda+\mathrm{1}\right)\left(\mathrm{2}\lambda+\mathrm{1}\right)}}{\mathrm{8}\left(\lambda+\mathrm{1}\right)} \\ $$$${t}=\frac{−\lambda+\mathrm{3}\lambda+\mathrm{2}}{\mathrm{4}\left(\lambda+\mathrm{1}\right)}=\frac{\mathrm{1}}{\mathrm{2}}=\mathrm{sin}\:\theta \\ $$$${that}\:{means}\:{the}\:{solution}\:{is}\:{the}\:{same} \\ $$$${even}\:{when}\:{both}\:{objects}\:{have}\:{different} \\ $$$${masses}. \\ $$

Commented by ajfour last updated on 12/Jul/20

Great! I got chance to understand the principle once again Sir. Thanksfor this viewpoint.

$${Great}!\:\:{I}\:{got}\:{chance}\:{to}\:{understand} \\ $$$${the}\:{principle}\:{once}\:{again}\:{Sir}. \\ $$$${Thanksfor}\:{this}\:{viewpoint}. \\ $$

Commented by ajfour last updated on 12/Jul/20

Nice, what made you suspect so Sir ?

$${Nice},\:{what}\:{made}\:{you}\:{suspect}\:{so} \\ $$$${Sir}\:? \\ $$

Commented by mr W last updated on 12/Jul/20

for θ=30° we can see that both masses are at the same point, that means it doesn′t matter how large the masses are, i.e. θ=30° is always a solution for any masses. i wanted know if other solution is possible. now i know it is not possible.

$${for}\:\theta=\mathrm{30}°\:{we}\:{can}\:{see}\:{that}\:{both} \\ $$$${masses}\:{are}\:{at}\:{the}\:{same}\:{point},\:{that} \\ $$$${means}\:{it}\:{doesn}'{t}\:{matter}\:{how}\:{large} \\ $$$${the}\:{masses}\:{are},\:{i}.{e}.\:\theta=\mathrm{30}°\:{is}\:{always} \\ $$$${a}\:{solution}\:{for}\:{any}\:{masses}.\:{i}\:{wanted}\: \\ $$$${know}\:{if}\:{other}\:{solution}\:{is}\:{possible}. \\ $$$${now}\:{i}\:{know}\:{it}\:{is}\:{not}\:{possible}. \\ $$

Answered by ajfour last updated on 12/Jul/20

Commented by ajfour last updated on 12/Jul/20

F=mgcos θ And as block is on verge of slipping f=μF = μmgcos θ=mgsin θ ⇒ μ=tan θ .....(i) ⇒ sin θ=(μ/(√(1+μ^2 ))) ....(ii) system: block+inclined plane_(−) N=μR (ΣF_x =0) .....(iii) μN+R=2mg (ΣF_y =0) ⇒ μ^2 R+R=2mg ⇒ ((mg)/R)=((μ^2 +1)/2) .....(iv) Torque about position of block_(−) (μNcos θ+Nsin θ)x −(mgcos θ)(x−(L/2)) = (Rcos θ−μRsin θ)(L−x) Now dividing by RLcos θ and using eqs. (i)→(iv) namely (N/R)=μ , ((mg)/R)=((μ^2 +1)/2) , tan θ=μ ; (x/L)=sin θ=(μ/(√(μ^2 +1))) we arrive at μ(μ+μ)((μ/(√(μ^2 +1)))) −(((μ^2 +1)/2))((μ/(√(μ^2 +1)))−(1/2)) = (1−μ^2 )(1−(μ/(√(μ^2 +1)))) or in terms of θ 2tan^2 θsin θ−(((sin θ−(1/2)))/(2cos^2 θ)) =(1−tan^2 θ)(1−sin θ) let sin θ=t ⇒ 2t^3 −(((2t−1))/4)=(1−2t^2 )(1−t) ⇒ 2t^3 −(t/2)+(1/4)=1+2t^3 −t−2t^2 ⇒ 8t^2 +2t−3=0 ⇒ 8(t+(1/8))^2 =((25)/8) ⇒ t=−(1/8)±(5/8) ⇒t= sin θ=(1/2) & μ=tan θ=(1/(√3)) ■

$${F}={mg}\mathrm{cos}\:\theta \\ $$$${And}\:{as}\:{block}\:{is}\:{on}\:{verge}\:{of}\:{slipping} \\ $$$$\:\:\:{f}=\mu{F}\:=\:\mu{mg}\mathrm{cos}\:\theta={mg}\mathrm{sin}\:\theta \\ $$$$\Rightarrow\:\:\:\mu=\mathrm{tan}\:\theta\:\:\:\:\:\:\:.....\left({i}\right) \\ $$$$\Rightarrow\:\:\mathrm{sin}\:\theta=\frac{\mu}{\sqrt{\mathrm{1}+\mu^{\mathrm{2}} }}\:\:\:\:\:\:....\left({ii}\right) \\ $$$$\underset{−} {{system}:\:{block}+{inclined}\:{plane}} \\ $$$$\:\:\:{N}=\mu{R}\:\:\:\:\:\:\:\:\:\left(\Sigma{F}_{{x}} =\mathrm{0}\right)\:\:\:\:\:.....\left({iii}\right) \\ $$$$\:\:\:\:\mu{N}+{R}=\mathrm{2}{mg}\:\:\:\:\:\left(\Sigma{F}_{{y}} =\mathrm{0}\right) \\ $$$$\Rightarrow\:\:\:\:\mu^{\mathrm{2}} {R}+{R}=\mathrm{2}{mg} \\ $$$$\Rightarrow\:\:\:\:\frac{{mg}}{{R}}=\frac{\mu^{\mathrm{2}} +\mathrm{1}}{\mathrm{2}}\:\:\:\:\:\:\:.....\left({iv}\right) \\ $$$$\:\underset{−} {{Torque}\:{about}\:{position}\:{of}\:{block}} \\ $$$$\:\:\left(\mu{N}\mathrm{cos}\:\theta+{N}\mathrm{sin}\:\theta\right){x} \\ $$$$−\left({mg}\mathrm{cos}\:\theta\right)\left({x}−\frac{{L}}{\mathrm{2}}\right) \\ $$$$\:\:\:\:\:=\:\left({R}\mathrm{cos}\:\theta−\mu{R}\mathrm{sin}\:\theta\right)\left({L}−{x}\right) \\ $$$${Now}\:{dividing}\:{by}\:{RL}\mathrm{cos}\:\theta \\ $$$${and}\:{using}\:{eqs}.\:\left({i}\right)\rightarrow\left({iv}\right)\:{namely} \\ $$$$\:\:\frac{{N}}{{R}}=\mu\:\:,\:\:\frac{{mg}}{{R}}=\frac{\mu^{\mathrm{2}} +\mathrm{1}}{\mathrm{2}}\:,\:\mathrm{tan}\:\theta=\mu\:; \\ $$$$\:\:\:\frac{{x}}{{L}}=\mathrm{sin}\:\theta=\frac{\mu}{\sqrt{\mu^{\mathrm{2}} +\mathrm{1}}}\:\:\:{we}\:{arrive}\:{at} \\ $$$$\:\:\mu\left(\mu+\mu\right)\left(\frac{\mu}{\sqrt{\mu^{\mathrm{2}} +\mathrm{1}}}\right) \\ $$$$\:\:−\left(\frac{\mu^{\mathrm{2}} +\mathrm{1}}{\mathrm{2}}\right)\left(\frac{\mu}{\sqrt{\mu^{\mathrm{2}} +\mathrm{1}}}−\frac{\mathrm{1}}{\mathrm{2}}\right) \\ $$$$\:=\:\left(\mathrm{1}−\mu^{\mathrm{2}} \right)\left(\mathrm{1}−\frac{\mu}{\sqrt{\mu^{\mathrm{2}} +\mathrm{1}}}\right) \\ $$$${or}\:{in}\:{terms}\:{of}\:\theta \\ $$$$\mathrm{2tan}\:^{\mathrm{2}} \theta\mathrm{sin}\:\theta−\frac{\left(\mathrm{sin}\:\theta−\frac{\mathrm{1}}{\mathrm{2}}\right)}{\mathrm{2cos}\:^{\mathrm{2}} \theta} \\ $$$$\:\:\:\:\:\:=\left(\mathrm{1}−\mathrm{tan}\:^{\mathrm{2}} \theta\right)\left(\mathrm{1}−\mathrm{sin}\:\theta\right) \\ $$$${let}\:\:\mathrm{sin}\:\theta={t} \\ $$$$\:\Rightarrow\:\:\mathrm{2}{t}^{\mathrm{3}} −\frac{\left(\mathrm{2}{t}−\mathrm{1}\right)}{\mathrm{4}}=\left(\mathrm{1}−\mathrm{2}{t}^{\mathrm{2}} \right)\left(\mathrm{1}−{t}\right) \\ $$$$\Rightarrow\:\:\mathrm{2}{t}^{\mathrm{3}} −\frac{{t}}{\mathrm{2}}+\frac{\mathrm{1}}{\mathrm{4}}=\mathrm{1}+\mathrm{2}{t}^{\mathrm{3}} −{t}−\mathrm{2}{t}^{\mathrm{2}} \\ $$$$\Rightarrow\:\:\:\mathrm{8}{t}^{\mathrm{2}} +\mathrm{2}{t}−\mathrm{3}=\mathrm{0} \\ $$$$\Rightarrow\:\:\:\mathrm{8}\left({t}+\frac{\mathrm{1}}{\mathrm{8}}\right)^{\mathrm{2}} =\frac{\mathrm{25}}{\mathrm{8}} \\ $$$$\Rightarrow\:\:{t}=−\frac{\mathrm{1}}{\mathrm{8}}\pm\frac{\mathrm{5}}{\mathrm{8}} \\ $$$$\Rightarrow{t}=\:\mathrm{sin}\:\theta=\frac{\mathrm{1}}{\mathrm{2}}\:\:\:\&\:\:\mu=\mathrm{tan}\:\theta=\frac{\mathrm{1}}{\sqrt{\mathrm{3}}}\:\blacksquare \\ $$

Commented by mr W last updated on 12/Jul/20

wonderful solution sir!

$${wonderful}\:{solution}\:{sir}! \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com