given 5x+12y = 60 min value of (√(x^2 +y^2 ))


Question and Answers Forum

All Questions Topic List
Integration Questions
Previous in All Question Next in All Question
Previous in Integration Next in Integration
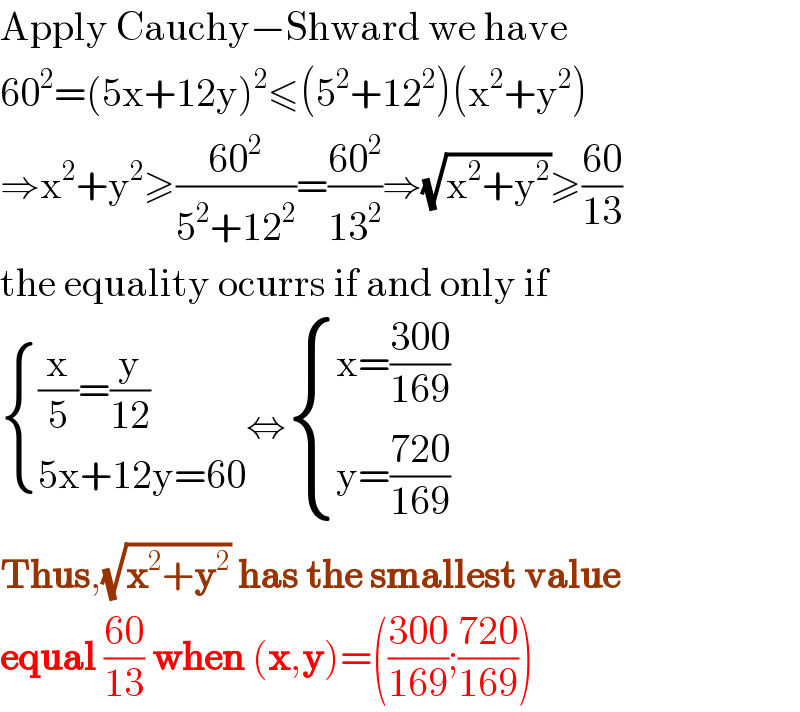
Question Number 103040 by bobhans last updated on 12/Jul/20

$g i v e n 5 x + 12 y = 60$ $m i n v a l u e o f \sqrt{x^{2} + y^{2}}$
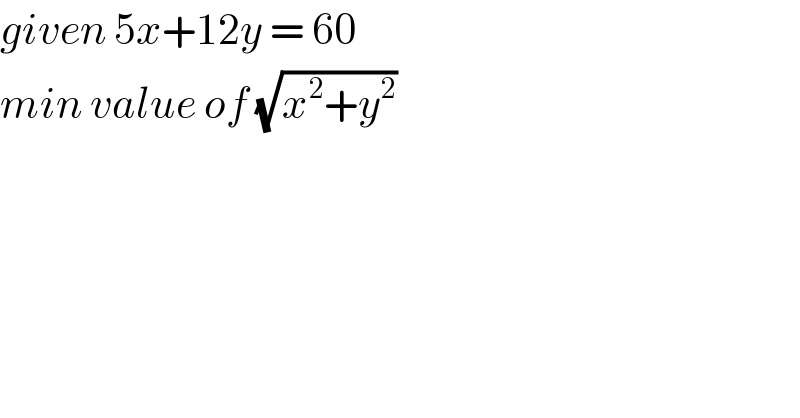
	Answered by bobhans last updated on 12/Jul/20

	Commented by mathmax by abdo last updated on 12/Jul/20

	$error sir \frac{\partial f}{\partial λ} = 5 x + 2 y - 60!$
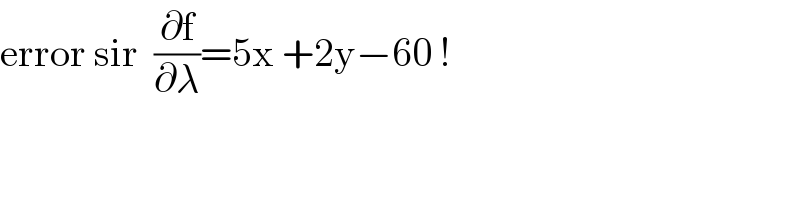
	Commented by bemath last updated on 12/Jul/20

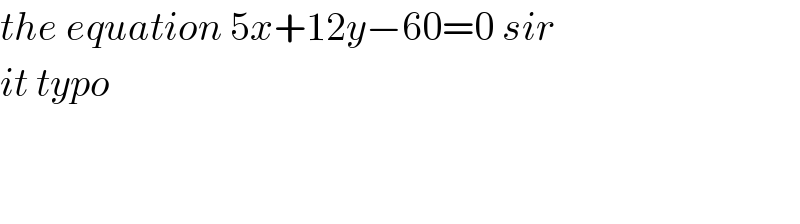
	$t h e e q u a t i o n 5 x + 12 y - 60 = 0 s i r$ $i t t y p o$
	Answered by ajfour last updated on 12/Jul/20
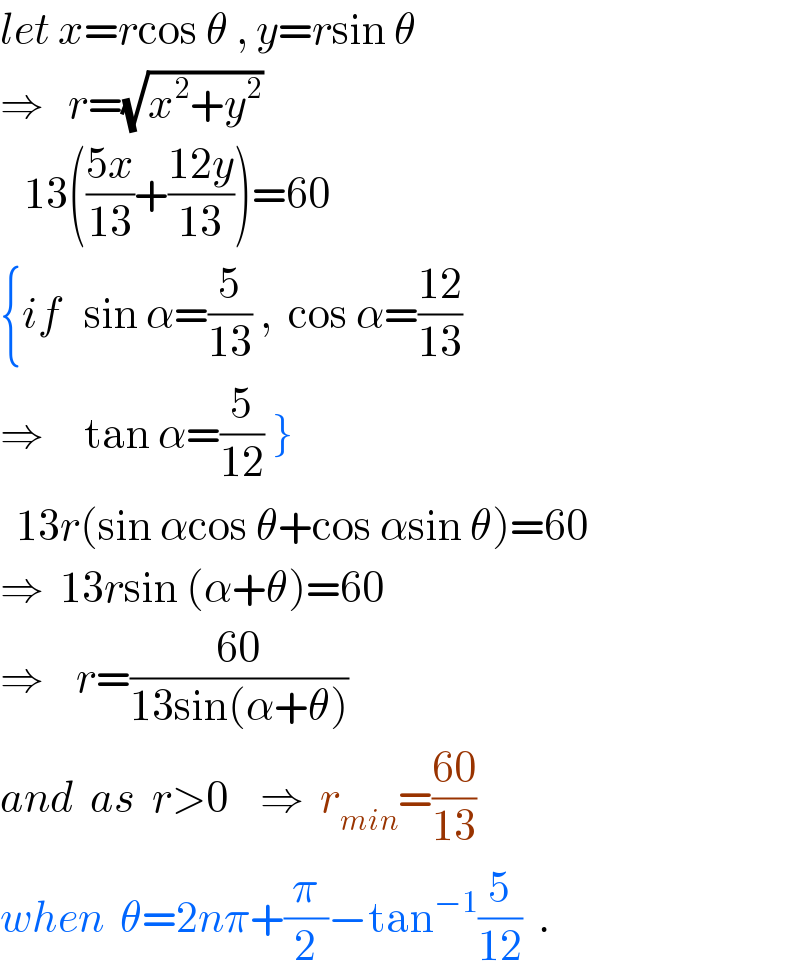
	$let x=rcos θ , y=rsin θ ⇒ r=(√(x^2 +y^2 )) 13(((5x)/(13))+((12y)/(13)))=60 {if sin α=(5/(13)) , cos α=((12)/(13)) ⇒ tan α=(5/(12)) } 13r(sin αcos θ+cos αsin θ)=60 ⇒ 13rsin (α+θ)=60 ⇒ r=((60)/(13sin(α+θ))) and as r>0 ⇒ r_(min) =((60)/(13)) when θ=2nπ+(π/2)−tan^(−1) (5/(12)) .$
	$l e t x = r \cos θ, y = r \sin θ$ $\Rightarrow r = \sqrt{x^{2} + y^{2}}$ $13 (\frac{5 x}{13} + \frac{12 y}{13}) = 60$ ${i f \sin α = \frac{5}{13}, \cos α = \frac{12}{13}$ $\Rightarrow \tan α = \frac{5}{12}}$ $13 r (\sin α \cos θ + \cos α \sin θ) = 60$ $\Rightarrow 13 r \sin (α + θ) = 60$ $\Rightarrow r = \frac{60}{13 \sin (α + θ)}$ $a n d a s r > 0 \Rightarrow r_{m i n} = \frac{60}{13}$ $w h e n θ = 2 n π + \frac{π}{2} - \tan^{- 1} \frac{5}{12} .$
	Answered by maths mind last updated on 12/Jul/20

	$M (x, y) \in (D) : 5 x + 12 y - 60 = 0$ $\sqrt{x^{2} + y^{2}} = d (O, M)$ $m i n d (O, M) = d (O, M^{'})$ $M^{'} p t o j e c t i o n o f O o v e r (d)$ $d (0, M^{'}) = \frac{∣ 0.5 + 12.0 - 60 ∣}{\sqrt{5^{2} + 12^{2}}} = \frac{60}{13}$
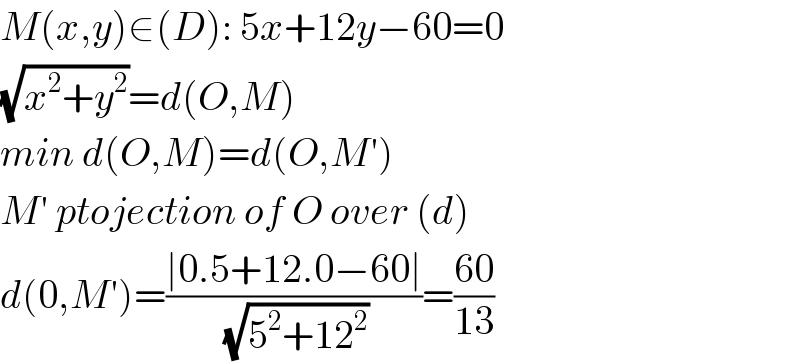
	Answered by mr W last updated on 12/Jul/20

	$s a y \sqrt{x^{2} + y^{2}} = D$ $x^{2} + y^{2} = D^{2}$ $x^{2} + {(5 - \frac{5 x}{12})}^{2} = D^{2}$ $\frac{169}{144} x^{2} - \frac{50}{12} x + 25 - D^{2} = 0$ $Δ = {(\frac{50}{12})}^{2} - 4 \times \frac{169}{144} (25 - D^{2}) = 0$ $D^{2} = \frac{3600}{169}$ $\Rightarrow D = \frac{60}{13}$
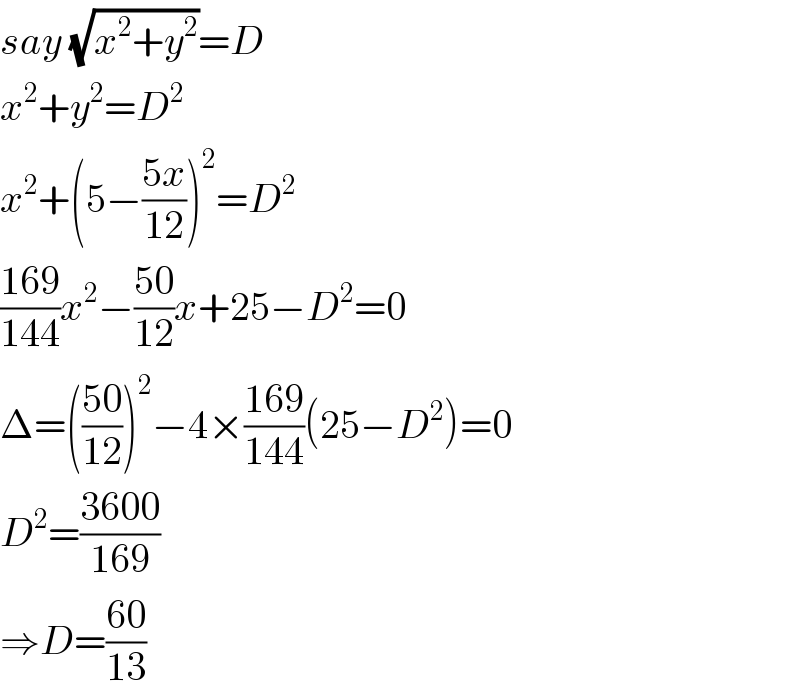
	Answered by 1549442205 last updated on 13/Jul/20
	$Apply Cauchy−Shward we have 60^2 =(5x+12y)^2 ≤(5^2 +12^2 )(x^2 +y^2 ) ⇒x^2 +y^2 ≥((60^2 )/(5^2 +12^2 ))=((60^2 )/(13^2 ))⇒(√(x^2 +y^2 ))≥((60)/(13)) the equality ocurrs if and only if { (((x/5)=(y/(12)))),((5x+12y=60)) :}⇔ { ((x=((300)/(169)))),((y=((720)/(169)))) :} Thus,(√(x^2 +y^2 )) has the smallest value equal ((60)/(13)) when (x,y)=(((300)/(169));((720)/(169)))$
	$Apply Cauchy - Shward we have$ $60^{2} = {(5 x + 12 y)}^{2} ⩽ (5^{2} + 12^{2}) (x^{2} + y^{2})$ $\Rightarrow x^{2} + y^{2} ⩾ \frac{60^{2}}{5^{2} + 12^{2}} = \frac{60^{2}}{13^{2}} \Rightarrow \sqrt{x^{2} + y^{2}} ⩾ \frac{60}{13}$ $the equality ocurrs if and only if$ ${\begin{cases} \frac{x}{5} = \frac{y}{12} \\ 5 x + 12 y = 60 \end{cases} \Leftrightarrow {\begin{cases} x = \frac{300}{169} \\ y = \frac{720}{169} \end{cases}$ $Thus, \sqrt{x^{2} + y^{2}} has the smallest value$ $equal \frac{60}{13} when (x, y) = (\frac{300}{169}; \frac{720}{169})$
Terms of Service
Privacy Policy
Contact: info@tinkutara.com

Question and Answers Forum