
Question Number 103196 by bobhans last updated on 13/Jul/20
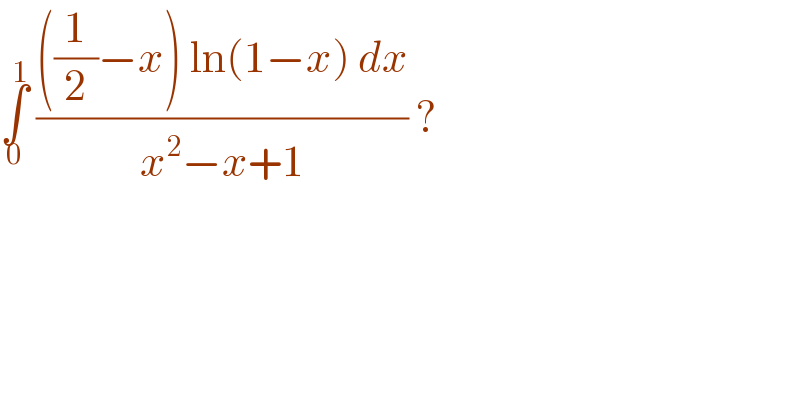
$$\underset{\mathrm{0}} {\overset{\mathrm{1}} {\int}}\:\frac{\left(\frac{\mathrm{1}}{\mathrm{2}}−{x}\right)\:\mathrm{ln}\left(\mathrm{1}−{x}\right)\:{dx}}{{x}^{\mathrm{2}} −{x}+\mathrm{1}}\:? \\ $$
Answered by bramlex last updated on 13/Jul/20
![replace x with 1−x I = ∫_0 ^1 (((x−(1/2)) ln x)/(x^2 −x+1)) dx [ by parts ] { ((u = ln x)),((dv = (((x−(1/2)))/(x^2 −x+1)) dx)) :} I = (1/2)ln (x) ln(x^2 −x+1)∣_0 ^1 − (1/2)∫_0 ^1 ((ln(x^2 −x+1))/x) dx I= −(1/2)∫_0 ^1 (( ln(((x^3 +1)/(x+1))))/x) dx I= (1/2)∫_0 ^1 ((ln(x+1))/x) dx −(1/2)∫_0 ^1 ((ln(x^3 +1))/x) dx I= (1/2)∫_0 ^1 (1/x)Σ_(n=1) ^∞ (((−1)^(n−1) x^n )/n) dx − (1/2)∫_0 ^1 (1/x)Σ_(n=1) ^∞ (((−1)^(n−1) x^(3n) )/n)dx I =(1/2)Σ_(n=1) ^∞ ∫_0 ^1 (((−1)^(n−1) x^(n−1) )/n) dx − (1/2)Σ_(n=1) ^∞ ∫_0 ^1 (((−1)^(n−1) x^(3n−1) )/n) dx I=(1/2)Σ_(n=1) ^∞ (((−1)^(n−1) )/n^2 )− (1/2)Σ_(n=1) ^∞ (((−1)^(n−1) )/(3n)) I= ((1/2)−(1/6))Σ_(n=1) ^∞ (((−1)^(n−1) )/n^2 ) I=(1/3)Σ_(n=1) ^∞ (((−1)^(n−1) )/n^2 ) . however Σ_(n=1) ^∞ (((−1)^(n−1) )/n^2 ) = Σ_(n=1) ^∞ (1/n^2 ) − Σ_(n=1) ^∞ (2/((2n)^2 )) = (1−(1/2)) Σ_(n=1) ^∞ (1/n^2 ) = (1/2)Σ_(n=1) ^∞ (1/n^2 ) = (1/2)×(π^2 /6) = (π^2 /(12)) therefore we conclude that I=∫_0 ^1 ((((1/2)−x)ln(1−x) dx)/(x^2 −x+1)) = (1/3)×(π^2 /(12)) = (π^2 /(36)) . ⊕](Q103197.png)
$$\mathrm{replace}\:\mathrm{x}\:\mathrm{with}\:\mathrm{1}−\mathrm{x}\: \\ $$$$\mathrm{I}\:=\:\underset{\mathrm{0}} {\overset{\mathrm{1}} {\int}}\:\frac{\left(\mathrm{x}−\frac{\mathrm{1}}{\mathrm{2}}\right)\:\mathrm{ln}\:\mathrm{x}}{\mathrm{x}^{\mathrm{2}} −\mathrm{x}+\mathrm{1}}\:\mathrm{dx}\:\left[\:\mathrm{by}\:\mathrm{parts}\:\right] \\ $$$$\begin{cases}{\mathrm{u}\:=\:\mathrm{ln}\:\mathrm{x}}\\{\mathrm{dv}\:=\:\frac{\left(\mathrm{x}−\frac{\mathrm{1}}{\mathrm{2}}\right)}{\mathrm{x}^{\mathrm{2}} −\mathrm{x}+\mathrm{1}}\:\mathrm{dx}}\end{cases} \\ $$$$\mathrm{I}\:=\:\frac{\mathrm{1}}{\mathrm{2}}\mathrm{ln}\:\left(\mathrm{x}\right)\:\mathrm{ln}\left(\mathrm{x}^{\mathrm{2}} −\mathrm{x}+\mathrm{1}\right)\mid_{\mathrm{0}} ^{\mathrm{1}} \:− \\ $$$$\frac{\mathrm{1}}{\mathrm{2}}\underset{\mathrm{0}} {\overset{\mathrm{1}} {\int}}\:\frac{\mathrm{ln}\left(\mathrm{x}^{\mathrm{2}} −\mathrm{x}+\mathrm{1}\right)}{\mathrm{x}}\:\mathrm{dx}\: \\ $$$$\mathrm{I}=\:−\frac{\mathrm{1}}{\mathrm{2}}\underset{\mathrm{0}} {\overset{\mathrm{1}} {\int}}\frac{\:\:\mathrm{ln}\left(\frac{\mathrm{x}^{\mathrm{3}} +\mathrm{1}}{\mathrm{x}+\mathrm{1}}\right)}{\mathrm{x}}\:\mathrm{dx}\: \\ $$$$\mathrm{I}=\:\frac{\mathrm{1}}{\mathrm{2}}\underset{\mathrm{0}} {\overset{\mathrm{1}} {\int}}\:\frac{\mathrm{ln}\left(\mathrm{x}+\mathrm{1}\right)}{\mathrm{x}}\:\mathrm{dx} \\ $$$$−\frac{\mathrm{1}}{\mathrm{2}}\underset{\mathrm{0}} {\overset{\mathrm{1}} {\int}}\frac{\mathrm{ln}\left(\mathrm{x}^{\mathrm{3}} +\mathrm{1}\right)}{\mathrm{x}}\:\mathrm{dx}\: \\ $$$$\mathrm{I}=\:\frac{\mathrm{1}}{\mathrm{2}}\underset{\mathrm{0}} {\overset{\mathrm{1}} {\int}}\frac{\mathrm{1}}{\mathrm{x}}\underset{\mathrm{n}=\mathrm{1}} {\overset{\infty} {\sum}}\frac{\left(−\mathrm{1}\right)^{\mathrm{n}−\mathrm{1}} \:\mathrm{x}^{\mathrm{n}} }{\mathrm{n}}\:\mathrm{dx}\:− \\ $$$$\frac{\mathrm{1}}{\mathrm{2}}\underset{\mathrm{0}} {\overset{\mathrm{1}} {\int}}\frac{\mathrm{1}}{\mathrm{x}}\underset{\mathrm{n}=\mathrm{1}} {\overset{\infty} {\sum}}\frac{\left(−\mathrm{1}\right)^{\mathrm{n}−\mathrm{1}} \:\mathrm{x}^{\mathrm{3n}} }{\mathrm{n}}\mathrm{dx} \\ $$$$\mathrm{I}\:=\frac{\mathrm{1}}{\mathrm{2}}\underset{\mathrm{n}=\mathrm{1}} {\overset{\infty} {\sum}}\underset{\mathrm{0}} {\overset{\mathrm{1}} {\int}}\frac{\left(−\mathrm{1}\right)^{\mathrm{n}−\mathrm{1}} \mathrm{x}^{\mathrm{n}−\mathrm{1}} }{\mathrm{n}}\:\mathrm{dx}\:− \\ $$$$\frac{\mathrm{1}}{\mathrm{2}}\underset{\mathrm{n}=\mathrm{1}} {\overset{\infty} {\sum}}\underset{\mathrm{0}} {\overset{\mathrm{1}} {\int}}\frac{\left(−\mathrm{1}\right)^{\mathrm{n}−\mathrm{1}} \mathrm{x}^{\mathrm{3n}−\mathrm{1}} }{\mathrm{n}}\:\mathrm{dx}\: \\ $$$$\mathrm{I}=\frac{\mathrm{1}}{\mathrm{2}}\underset{\mathrm{n}=\mathrm{1}} {\overset{\infty} {\sum}}\frac{\left(−\mathrm{1}\right)^{\mathrm{n}−\mathrm{1}} }{\mathrm{n}^{\mathrm{2}} }− \\ $$$$\frac{\mathrm{1}}{\mathrm{2}}\underset{\mathrm{n}=\mathrm{1}} {\overset{\infty} {\sum}}\frac{\left(−\mathrm{1}\right)^{\mathrm{n}−\mathrm{1}} }{\mathrm{3n}} \\ $$$$\mathrm{I}=\:\left(\frac{\mathrm{1}}{\mathrm{2}}−\frac{\mathrm{1}}{\mathrm{6}}\right)\underset{\mathrm{n}=\mathrm{1}} {\overset{\infty} {\sum}}\frac{\left(−\mathrm{1}\right)^{\mathrm{n}−\mathrm{1}} }{\mathrm{n}^{\mathrm{2}} } \\ $$$$\mathrm{I}=\frac{\mathrm{1}}{\mathrm{3}}\underset{\mathrm{n}=\mathrm{1}} {\overset{\infty} {\sum}}\frac{\left(−\mathrm{1}\right)^{\mathrm{n}−\mathrm{1}} }{\mathrm{n}^{\mathrm{2}} }\:. \\ $$$$\mathrm{however}\:\underset{\mathrm{n}=\mathrm{1}} {\overset{\infty} {\sum}}\frac{\left(−\mathrm{1}\right)^{\mathrm{n}−\mathrm{1}} }{\mathrm{n}^{\mathrm{2}} }\:=\: \\ $$$$\underset{\mathrm{n}=\mathrm{1}} {\overset{\infty} {\sum}}\frac{\mathrm{1}}{\mathrm{n}^{\mathrm{2}} }\:−\:\underset{\mathrm{n}=\mathrm{1}} {\overset{\infty} {\sum}}\frac{\mathrm{2}}{\left(\mathrm{2n}\right)^{\mathrm{2}} } \\ $$$$=\:\left(\mathrm{1}−\frac{\mathrm{1}}{\mathrm{2}}\right)\:\underset{\mathrm{n}=\mathrm{1}} {\overset{\infty} {\sum}}\frac{\mathrm{1}}{\mathrm{n}^{\mathrm{2}} }\:=\:\frac{\mathrm{1}}{\mathrm{2}}\underset{\mathrm{n}=\mathrm{1}} {\overset{\infty} {\sum}}\frac{\mathrm{1}}{\mathrm{n}^{\mathrm{2}} } \\ $$$$=\:\frac{\mathrm{1}}{\mathrm{2}}×\frac{\pi^{\mathrm{2}} }{\mathrm{6}}\:=\:\frac{\pi^{\mathrm{2}} }{\mathrm{12}} \\ $$$$\mathrm{therefore}\:\mathrm{we}\:\mathrm{conclude}\:\mathrm{that}\: \\ $$$$\mathrm{I}=\underset{\mathrm{0}} {\overset{\mathrm{1}} {\int}}\:\frac{\left(\frac{\mathrm{1}}{\mathrm{2}}−\mathrm{x}\right)\mathrm{ln}\left(\mathrm{1}−\mathrm{x}\right)\:\mathrm{dx}}{\mathrm{x}^{\mathrm{2}} −\mathrm{x}+\mathrm{1}} \\ $$$$=\:\frac{\mathrm{1}}{\mathrm{3}}×\frac{\pi^{\mathrm{2}} }{\mathrm{12}}\:=\:\frac{\pi^{\mathrm{2}} }{\mathrm{36}}\:.\:\oplus\: \\ $$
Commented by Dwaipayan Shikari last updated on 13/Jul/20

$$\boldsymbol{\mathrm{Great}}\:\:\boldsymbol{\mathrm{sir}}! \\ $$
