Question and Answers Forum
Question Number 103366 by Study last updated on 14/Jul/20
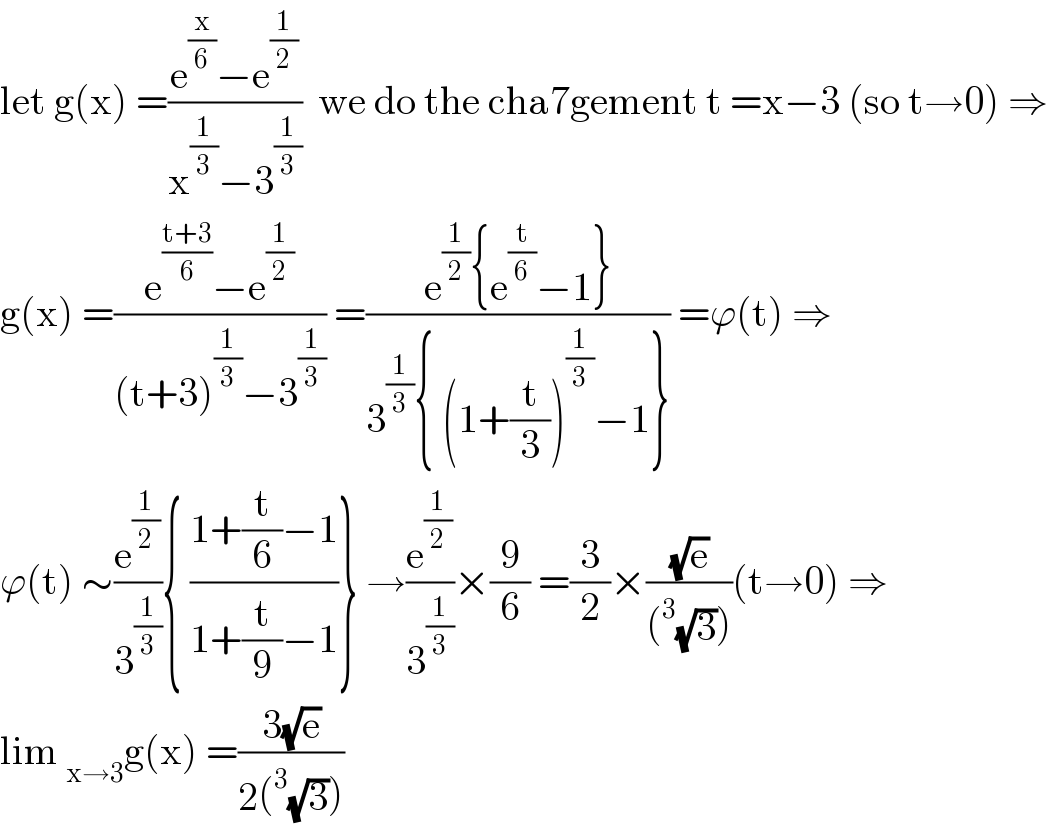
Answered by mathmax by abdo last updated on 14/Jul/20
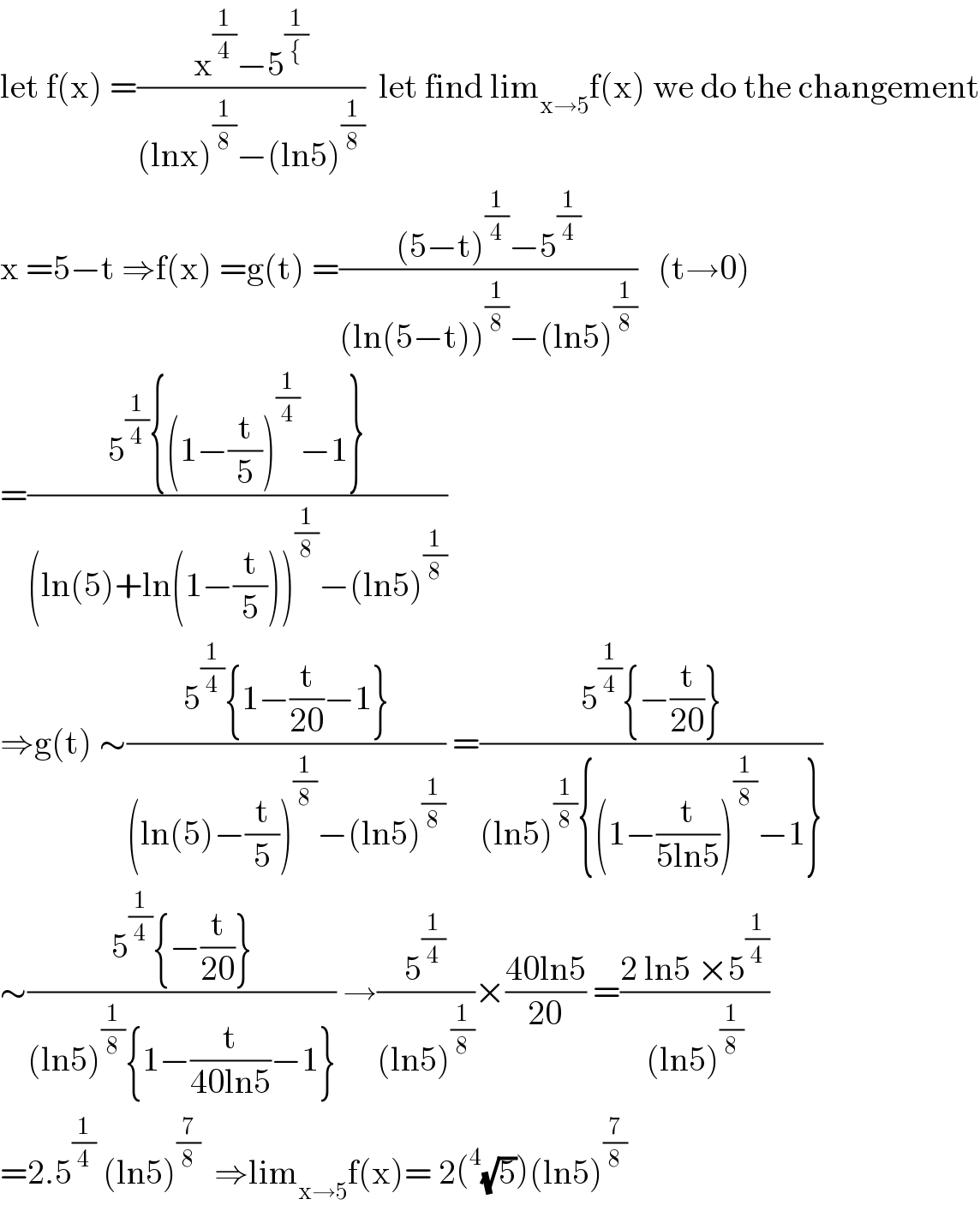
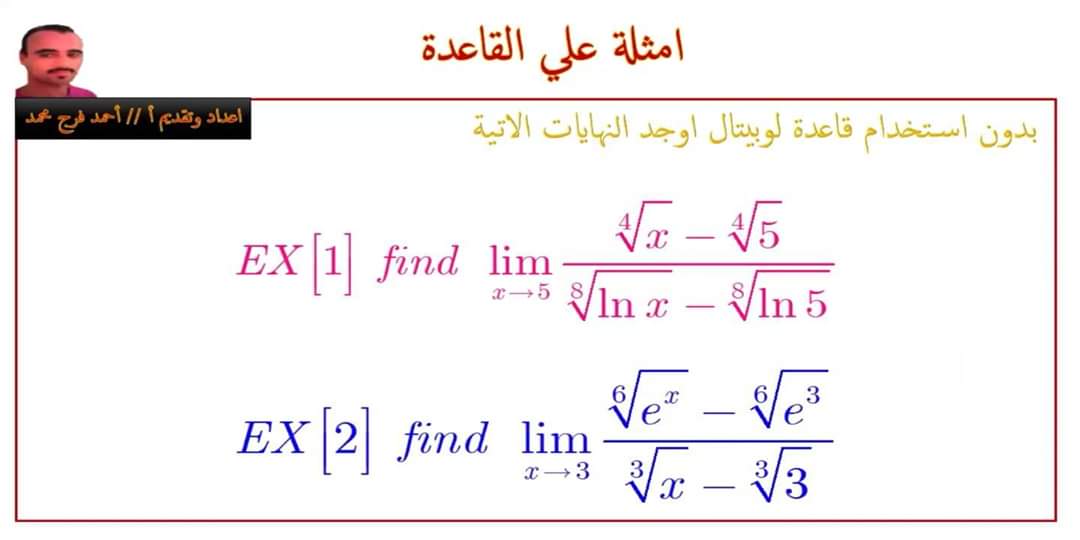
Answered by bemath last updated on 14/Jul/20
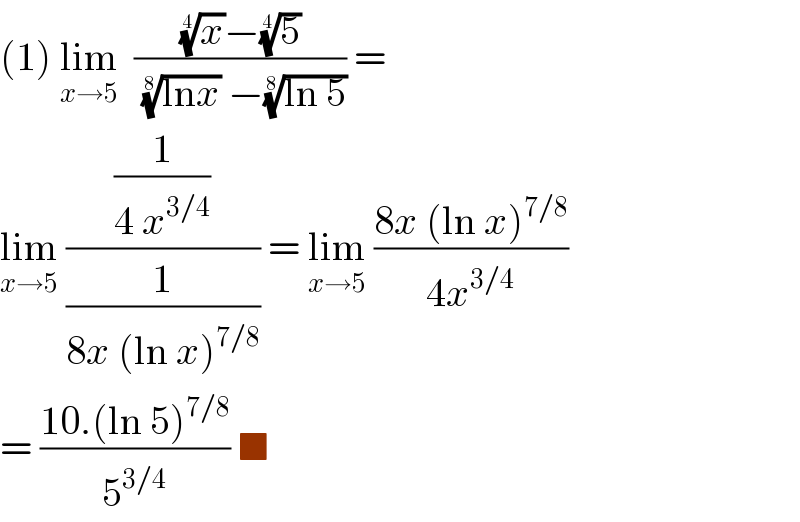
Answered by bemath last updated on 14/Jul/20
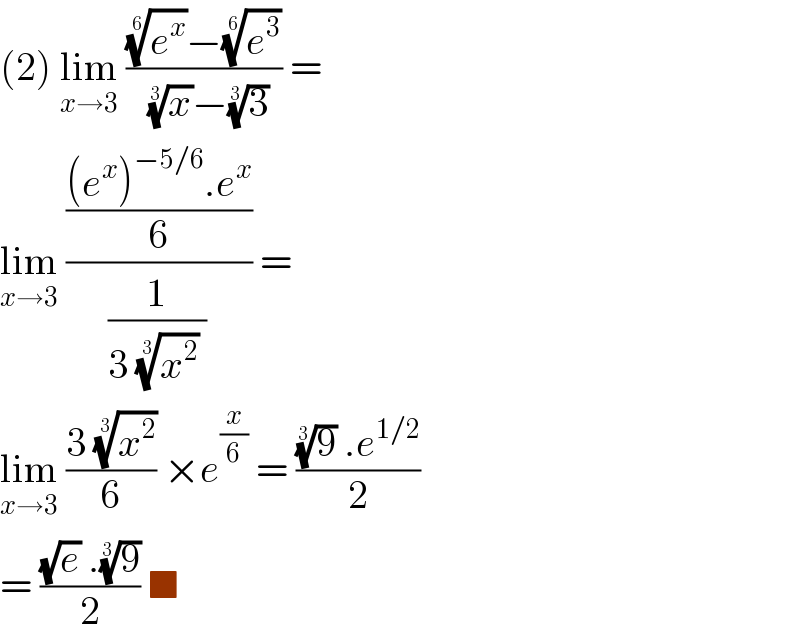
Answered by mathmax by abdo last updated on 15/Jul/20