
Question and Answers Forum
Question Number 103644 by dw last updated on 16/Jul/20

Commented by dw last updated on 16/Jul/20
![[Trigonometric Substit.]](Q103646.png)
Answered by 1549442205 last updated on 16/Jul/20
![putting x=cosϕ(ϕ∈[0,π])we have ((5cosϕ)/(sinϕ))−6cosϕsinϕ=2⇔5cosϕ−6cosϕsin^2 ϕ=2sinϕ Putting tan(ϕ/2)=t we get ((5(1−t^2 ))/(1+t^2 ))−((6(1−t^2 )4t^2 )/((1+t^2 )^3 ))=((4t)/(1+t^2 )) 5(1−t^4 )(1+t^2 )−24(t^2 −t^4 )=4t(t^4 +2t^2 +1) −5t^6 −5t^4 +5t^2 +5−24t^2 +24t^4 =4t^5 +8t^3 +4t ⇔5t^6 +4t^5 −19t^4 +8t^3 +19t^2 +4t−5=0 ⇔5(t^3 −(1/t^3 ))+4(t^2 +(1/t^2 ))−19(t−(1/t))+8=0(1) Putting (t−(1/t))=y⇒t^3 −(1/t^3 )−3(t−(1/t))=y^3 t^2 +(1/t^2 )=y^2 +2.Hence, (1)⇔5y^3 +4y^2 +8−4y+8=0 ⇔5y^3 +4y^2 −4y+16=0 ⇔(y+2)(5y^2 −6y+8)=0 ⇔y+2=0 (because 5y^2 −6y+8=y^2 +(2y−(3/2))^2 +((23)/4)>0) ⇔y=−2⇔t−(1/t)=−2⇔t^2 +2t−1=0 ⇔t=−1±(√2) i)For t=−1+(√2) ⇒t^2 =3−2(√2) we get x=cosϕ=((1−t^2 )/(1+t^2 ))=((2(√2)−2)/(4−2(√2)))=(((√2)−1)/(2−(√2)))=(1/(√2)) ii)For t=−1−(√2) ⇒t^2 =3+2(√2) we get x=cosϕ=((1−t^2 )/(1+t^2 ))=((−2(1+(√2)))/(2(2+(√(2)))))=((−1)/(√2)) Thus ,x∈{−(1/2);((√2)/(√2))} second way: ((5x)/(√(1−x^2 )))+6x(√(1−x^2 ))=2⇔5x−6x(1−x^2 )=2(√(1−x^2 )) ⇔(6x^3 −x)^2 =4(1−x^2 ) ⇔36x^6 −12x^4 +x^2 =4−4x^2 Putting x^2 =y we get 36y^3 −12y^2 +5y−4=0 ⇔(2y−1)(18y^2 −3y+4)=0 ⇔2y−1=0 because 18y^2 −3y+4=17y^2 +(y−(3/2))^2 +(7/4)>0 ⇔y=(1/2)⇔x^2 =(1/2)⇔x=±((√2)/2)](Q103657.png)
Commented by maths mind last updated on 16/Jul/20
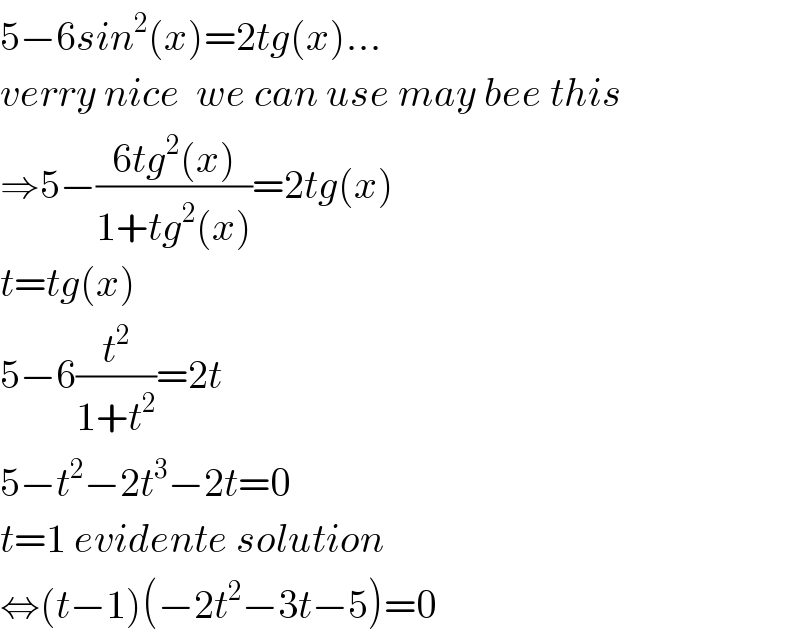
| ||
Question and Answers Forum | ||
Question Number 103644 by dw last updated on 16/Jul/20 | ||
 | ||
Commented by dw last updated on 16/Jul/20 | ||
![[Trigonometric Substit.]](Q103646.png) | ||
Answered by 1549442205 last updated on 16/Jul/20 | ||
![putting x=cosϕ(ϕ∈[0,π])we have ((5cosϕ)/(sinϕ))−6cosϕsinϕ=2⇔5cosϕ−6cosϕsin^2 ϕ=2sinϕ Putting tan(ϕ/2)=t we get ((5(1−t^2 ))/(1+t^2 ))−((6(1−t^2 )4t^2 )/((1+t^2 )^3 ))=((4t)/(1+t^2 )) 5(1−t^4 )(1+t^2 )−24(t^2 −t^4 )=4t(t^4 +2t^2 +1) −5t^6 −5t^4 +5t^2 +5−24t^2 +24t^4 =4t^5 +8t^3 +4t ⇔5t^6 +4t^5 −19t^4 +8t^3 +19t^2 +4t−5=0 ⇔5(t^3 −(1/t^3 ))+4(t^2 +(1/t^2 ))−19(t−(1/t))+8=0(1) Putting (t−(1/t))=y⇒t^3 −(1/t^3 )−3(t−(1/t))=y^3 t^2 +(1/t^2 )=y^2 +2.Hence, (1)⇔5y^3 +4y^2 +8−4y+8=0 ⇔5y^3 +4y^2 −4y+16=0 ⇔(y+2)(5y^2 −6y+8)=0 ⇔y+2=0 (because 5y^2 −6y+8=y^2 +(2y−(3/2))^2 +((23)/4)>0) ⇔y=−2⇔t−(1/t)=−2⇔t^2 +2t−1=0 ⇔t=−1±(√2) i)For t=−1+(√2) ⇒t^2 =3−2(√2) we get x=cosϕ=((1−t^2 )/(1+t^2 ))=((2(√2)−2)/(4−2(√2)))=(((√2)−1)/(2−(√2)))=(1/(√2)) ii)For t=−1−(√2) ⇒t^2 =3+2(√2) we get x=cosϕ=((1−t^2 )/(1+t^2 ))=((−2(1+(√2)))/(2(2+(√(2)))))=((−1)/(√2)) Thus ,x∈{−(1/2);((√2)/(√2))} second way: ((5x)/(√(1−x^2 )))+6x(√(1−x^2 ))=2⇔5x−6x(1−x^2 )=2(√(1−x^2 )) ⇔(6x^3 −x)^2 =4(1−x^2 ) ⇔36x^6 −12x^4 +x^2 =4−4x^2 Putting x^2 =y we get 36y^3 −12y^2 +5y−4=0 ⇔(2y−1)(18y^2 −3y+4)=0 ⇔2y−1=0 because 18y^2 −3y+4=17y^2 +(y−(3/2))^2 +(7/4)>0 ⇔y=(1/2)⇔x^2 =(1/2)⇔x=±((√2)/2)](Q103657.png) | ||
| ||
Commented by maths mind last updated on 16/Jul/20 | ||
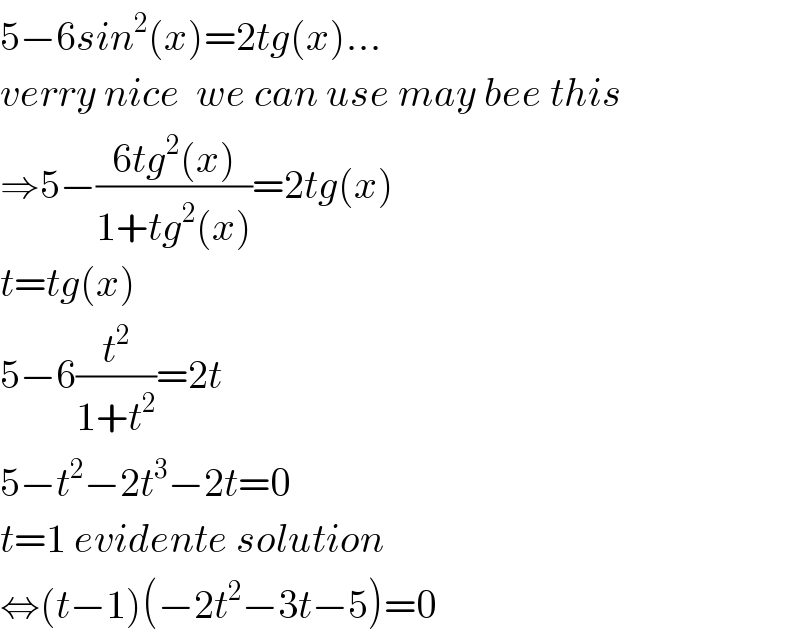 | ||