y′ − (y/(x^2 −1)) = y^2


Question and Answers Forum

All Questions Topic List
Differential Equation Questions
Previous in All Question Next in All Question
Previous in Differential Equation Next in Differential Equation
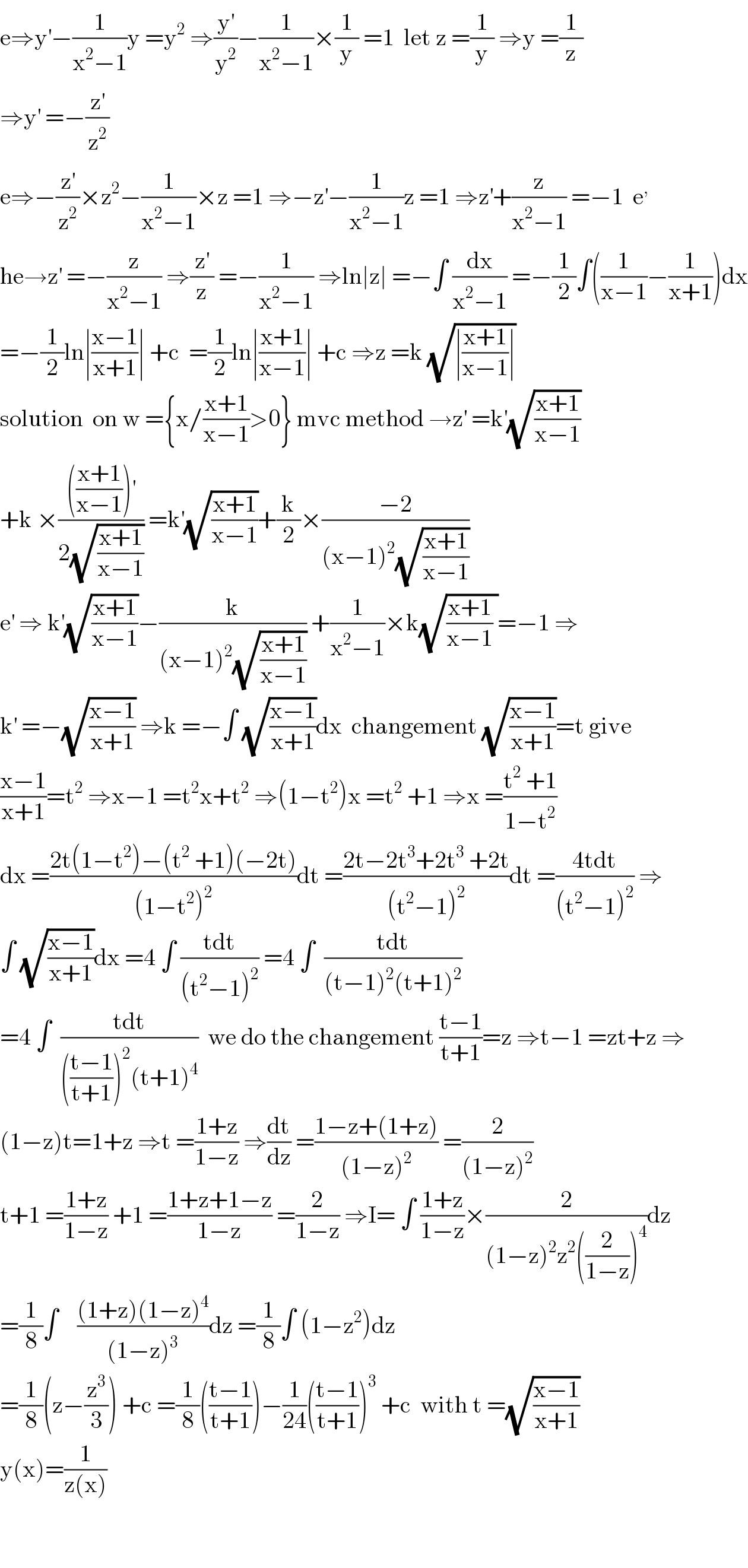
Question Number 103776 by bemath last updated on 17/Jul/20

$y^{'} - \frac{y}{x^{2} - 1} = y^{2}$
	Answered by bemath last updated on 17/Jul/20
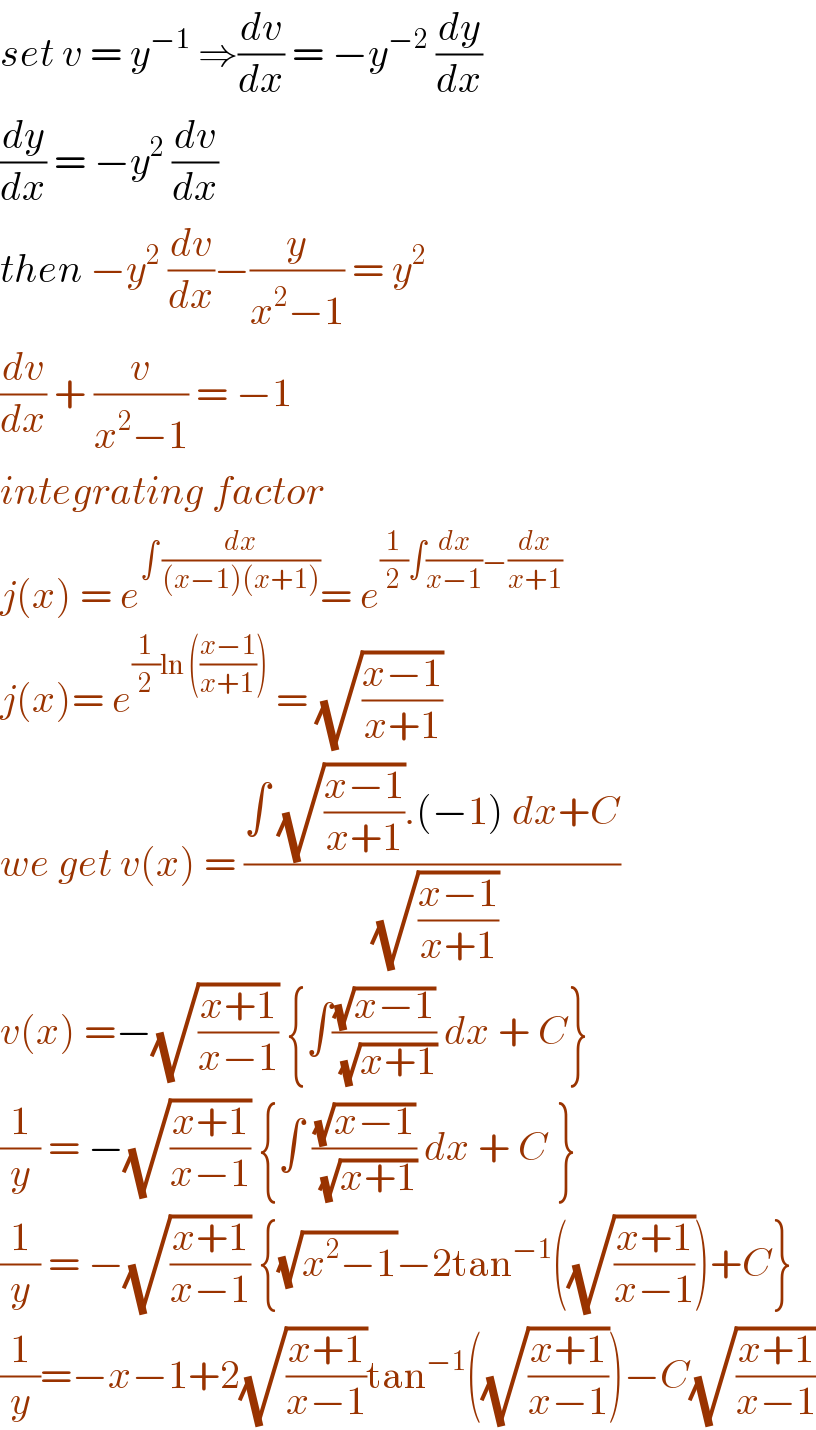
	$set v = y^(−1) ⇒(dv/dx) = −y^(−2) (dy/dx) (dy/dx) = −y^2 (dv/dx) then −y^2 (dv/dx)−(y/(x^2 −1)) = y^2 (dv/dx) + (v/(x^2 −1)) = −1 integrating factor j(x) = e^(∫ (dx/((x−1)(x+1)))) = e^((1/2)∫(dx/(x−1))−(dx/(x+1))) j(x)= e^((1/2)ln (((x−1)/(x+1)))) = (√((x−1)/(x+1))) we get v(x) = ((∫ (√((x−1)/(x+1))).(−1) dx+C)/(√((x−1)/(x+1)))) v(x) =−(√((x+1)/(x−1))) {∫((√(x−1))/(√(x+1))) dx + C} (1/y) = −(√((x+1)/(x−1))) {∫ ((√(x−1))/(√(x+1))) dx + C } (1/y) = −(√((x+1)/(x−1))) {(√(x^2 −1))−2tan^(−1) ((√((x+1)/(x−1))))+C} (1/y)=−x−1+2(√((x+1)/(x−1)))tan^(−1) ((√((x+1)/(x−1))))−C(√((x+1)/(x−1)))$
	$s e t v = y^{- 1} \Rightarrow \frac{d v}{d x} = - y^{- 2} \frac{d y}{d x}$ $\frac{d y}{d x} = - y^{2} \frac{d v}{d x}$ $t h e n - y^{2} \frac{d v}{d x} - \frac{y}{x^{2} - 1} = y^{2}$ $\frac{d v}{d x} + \frac{v}{x^{2} - 1} = - 1$ $i n t e g r a t i n g f a c t o r$ $j (x) = e^{\int \frac{d x}{(x - 1) (x + 1)}} = e^{\frac{1}{2} \int \frac{d x}{x - 1} - \frac{d x}{x + 1}}$ $j (x) = e^{\frac{1}{2} \ln (\frac{x - 1}{x + 1})} = \sqrt{\frac{x - 1}{x + 1}}$ $w e g e t v (x) = \frac{\int \sqrt{\frac{x - 1}{x + 1}} . (- 1) d x + C}{\sqrt{\frac{x - 1}{x + 1}}}$ $v (x) = - \sqrt{\frac{x + 1}{x - 1}} {\int \frac{\sqrt{x - 1}}{\sqrt{x + 1}} d x + C}$ $\frac{1}{y} = - \sqrt{\frac{x + 1}{x - 1}} {\int \frac{\sqrt{x - 1}}{\sqrt{x + 1}} d x + C}$ $\frac{1}{y} = - \sqrt{\frac{x + 1}{x - 1}} {\sqrt{x^{2} - 1} - {2 \tan}^{- 1} (\sqrt{\frac{x + 1}{x - 1}}) + C}$ $\frac{1}{y} = - x - 1 + 2 \sqrt{\frac{x + 1}{x - 1}} \tan^{- 1} (\sqrt{\frac{x + 1}{x - 1}}) - C \sqrt{\frac{x + 1}{x - 1}}$
	Commented by Ar Brandon last updated on 17/Jul/20
	What's the secret to knowing the right change of variable to use ? ��
	Commented by bobhans last updated on 17/Jul/20

	$(⌢∵⌢) \int \frac{\sqrt{x - 1}}{\sqrt{x + 1}} d x = I$ $I = \sqrt{(x - 1) (x + 1)} - {2 \tan}^{- 1} \sqrt{\frac{x + 1}{x - 1}} + k$
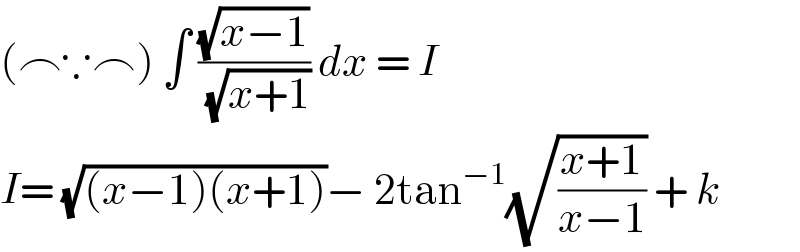
	Commented by bemath last updated on 17/Jul/20

	$B e r n o u l l i e q s i r$
	Commented by Coronavirus last updated on 17/Jul/20

	${i t}^{'} s a B e r n o u l l i e q u a t i o n$
	Answered by mathmax by abdo last updated on 17/Jul/20
	$e⇒y^′ −(1/(x^2 −1))y =y^2 ⇒(y^′ /y^2 )−(1/(x^2 −1))×(1/y) =1 let z =(1/y) ⇒y =(1/z) ⇒y^′ =−(z^′ /z^2 ) e⇒−(z^′ /z^2 )×z^2 −(1/(x^2 −1))×z =1 ⇒−z^′ −(1/(x^2 −1))z =1 ⇒z^′ +(z/(x^2 −1)) =−1 e^, he→z^′ =−(z/(x^2 −1)) ⇒(z^′ /z) =−(1/(x^2 −1)) ⇒ln∣z∣ =−∫ (dx/(x^2 −1)) =−(1/2)∫((1/(x−1))−(1/(x+1)))dx =−(1/2)ln∣((x−1)/(x+1))∣ +c =(1/2)ln∣((x+1)/(x−1))∣ +c ⇒z =k (√(∣((x+1)/(x−1))∣)) solution on w ={x/((x+1)/(x−1))>0} mvc method →z^′ =k^′ (√((x+1)/(x−1))) +k ×(((((x+1)/(x−1)))^′ )/(2(√((x+1)/(x−1))))) =k^′ (√((x+1)/(x−1)))+(k/2)×((−2)/((x−1)^2 (√((x+1)/(x−1))))) e^′ ⇒ k^′ (√((x+1)/(x−1)))−(k/((x−1)^2 (√((x+1)/(x−1))))) +(1/(x^2 −1))×k(√(((x+1)/(x−1)) ))=−1 ⇒ k^′ =−(√((x−1)/(x+1))) ⇒k =−∫ (√((x−1)/(x+1)))dx changement (√((x−1)/(x+1)))=t give ((x−1)/(x+1))=t^2 ⇒x−1 =t^2 x+t^2 ⇒(1−t^2 )x =t^2 +1 ⇒x =((t^2 +1)/(1−t^2 )) dx =((2t(1−t^2 )−(t^2 +1)(−2t))/((1−t^2 )^2 ))dt =((2t−2t^3 +2t^3 +2t)/((t^2 −1)^2 ))dt =((4tdt)/((t^2 −1)^2 )) ⇒ ∫ (√((x−1)/(x+1)))dx =4 ∫ ((tdt)/((t^2 −1)^2 )) =4 ∫ ((tdt)/((t−1)^2 (t+1)^2 )) =4 ∫ ((tdt)/((((t−1)/(t+1)))^2 (t+1)^4 )) we do the changement ((t−1)/(t+1))=z ⇒t−1 =zt+z ⇒ (1−z)t=1+z ⇒t =((1+z)/(1−z)) ⇒(dt/dz) =((1−z+(1+z))/((1−z)^2 )) =(2/((1−z)^2 )) t+1 =((1+z)/(1−z)) +1 =((1+z+1−z)/(1−z)) =(2/(1−z)) ⇒I= ∫ ((1+z)/(1−z))×(2/((1−z)^2 z^2 ((2/(1−z)))^4 ))dz =(1/8)∫ (((1+z)(1−z)^4 )/((1−z)^3 ))dz =(1/8)∫ (1−z^2 )dz =(1/8)(z−(z^3 /3)) +c =(1/8)(((t−1)/(t+1)))−(1/(24))(((t−1)/(t+1)))^3 +c with t =(√((x−1)/(x+1))) y(x)=(1/(z(x)))$
	$e \Rightarrow y^{^{'}} - \frac{1}{x^{2} - 1} y = y^{2} \Rightarrow \frac{y^{^{'}}}{y^{2}} - \frac{1}{x^{2} - 1} \times \frac{1}{y} = 1 let z = \frac{1}{y} \Rightarrow y = \frac{1}{z}$ $\Rightarrow y^{^{'}} = - \frac{z^{^{'}}}{z^{2}}$ $e \Rightarrow - \frac{z^{^{'}}}{z^{2}} \times z^{2} - \frac{1}{x^{2} - 1} \times z = 1 \Rightarrow - z^{^{'}} - \frac{1}{x^{2} - 1} z = 1 \Rightarrow z^{^{'}} + \frac{z}{x^{2} - 1} = - 1 e^{,}$ $he \to z^{^{'}} = - \frac{z}{x^{2} - 1} \Rightarrow \frac{z^{^{'}}}{z} = - \frac{1}{x^{2} - 1} \Rightarrow \ln ∣ z ∣ = - \int \frac{dx}{x^{2} - 1} = - \frac{1}{2} \int (\frac{1}{x - 1} - \frac{1}{x + 1}) dx$ $= - \frac{1}{2} \ln ∣ \frac{x - 1}{x + 1} ∣ + c = \frac{1}{2} \ln ∣ \frac{x + 1}{x - 1} ∣ + c \Rightarrow z = k \sqrt{∣ \frac{x + 1}{x - 1} ∣}$ $solution on w = {x / \frac{x + 1}{x - 1} > 0} mvc method \to z^{^{'}} = k^{^{'}} \sqrt{\frac{x + 1}{x - 1}}$ $+ k \times \frac{{(\frac{x + 1}{x - 1})}^{^{'}}}{2 \sqrt{\frac{x + 1}{x - 1}}} = k^{^{'}} \sqrt{\frac{x + 1}{x - 1}} + \frac{k}{2} \times \frac{- 2}{{(x - 1)}^{2} \sqrt{\frac{x + 1}{x - 1}}}$ $e^{^{'}} \Rightarrow k^{^{'}} \sqrt{\frac{x + 1}{x - 1}} - \frac{k}{{(x - 1)}^{2} \sqrt{\frac{x + 1}{x - 1}}} + \frac{1}{x^{2} - 1} \times k \sqrt{\frac{x + 1}{x - 1}} = - 1 \Rightarrow$ $k^{^{'}} = - \sqrt{\frac{x - 1}{x + 1}} \Rightarrow k = - \int \sqrt{\frac{x - 1}{x + 1}} dx changement \sqrt{\frac{x - 1}{x + 1}} = t give$ $\frac{x - 1}{x + 1} = t^{2} \Rightarrow x - 1 = t^{2} x + t^{2} \Rightarrow (1 - t^{2}) x = t^{2} + 1 \Rightarrow x = \frac{t^{2} + 1}{1 - t^{2}}$ $dx = \frac{2 t (1 - t^{2}) - (t^{2} + 1) (- 2 t)}{{(1 - t^{2})}^{2}} dt = \frac{2 t - {2 t}^{3} + {2 t}^{3} + 2 t}{{(t^{2} - 1)}^{2}} dt = \frac{4 tdt}{{(t^{2} - 1)}^{2}} \Rightarrow$ $\int \sqrt{\frac{x - 1}{x + 1}} dx = 4 \int \frac{tdt}{{(t^{2} - 1)}^{2}} = 4 \int \frac{tdt}{{(t - 1)}^{2} {(t + 1)}^{2}}$ $= 4 \int \frac{tdt}{{(\frac{t - 1}{t + 1})}^{2} {(t + 1)}^{4}} we do the changement \frac{t - 1}{t + 1} = z \Rightarrow t - 1 = zt + z \Rightarrow$ $(1 - z) t = 1 + z \Rightarrow t = \frac{1 + z}{1 - z} \Rightarrow \frac{dt}{dz} = \frac{1 - z + (1 + z)}{{(1 - z)}^{2}} = \frac{2}{{(1 - z)}^{2}}$ $t + 1 = \frac{1 + z}{1 - z} + 1 = \frac{1 + z + 1 - z}{1 - z} = \frac{2}{1 - z} \Rightarrow I = \int \frac{1 + z}{1 - z} \times \frac{2}{{(1 - z)}^{2} z^{2} {(\frac{2}{1 - z})}^{4}} dz$ $= \frac{1}{8} \int \frac{(1 + z) {(1 - z)}^{4}}{{(1 - z)}^{3}} dz = \frac{1}{8} \int (1 - z^{2}) dz$ $= \frac{1}{8} (z - \frac{z^{3}}{3}) + c = \frac{1}{8} (\frac{t - 1}{t + 1}) - \frac{1}{24} {(\frac{t - 1}{t + 1})}^{3} + c with t = \sqrt{\frac{x - 1}{x + 1}}$ $y (x) = \frac{1}{z (x)}$
Terms of Service
Privacy Policy
Contact: info@tinkutara.com

Question and Answers Forum