Question and Answers Forum

All Questions      Topic List

Integration Questions

Previous in All Question      Next in All Question      

Previous in Integration      Next in Integration      

Question Number 103825 by mohammad17 last updated on 17/Jul/20

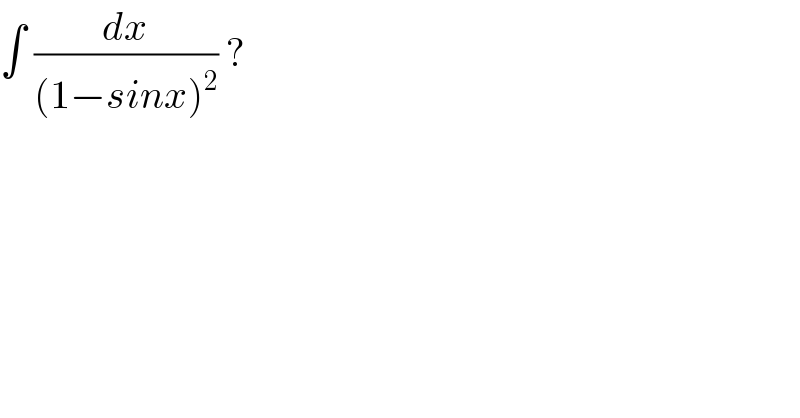
∫ (dx/((1−sinx)^2 )) ?

$$\int\:\frac{{dx}}{\left(\mathrm{1}−{sinx}\right)^{\mathrm{2}} }\:? \\ $$

Answered by ~blr237~ last updated on 17/Jul/20

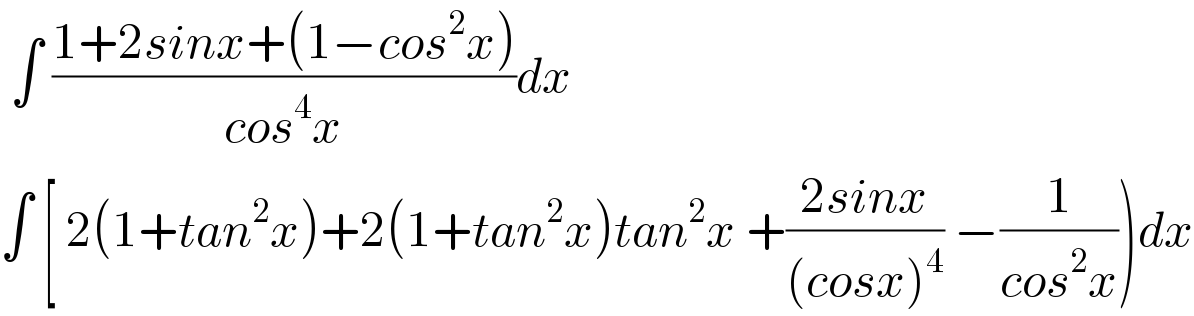
∫ ((1+2sinx+(1−cos^2 x))/(cos^4 x))dx ∫ [ 2(1+tan^2 x)+2(1+tan^2 x)tan^2 x +((2sinx)/((cosx)^4 )) −(1/(cos^2 x)))dx

$$\:\int\:\frac{\mathrm{1}+\mathrm{2}{sinx}+\left(\mathrm{1}−{cos}^{\mathrm{2}} {x}\right)}{{cos}^{\mathrm{4}} {x}}{dx} \\ $$$$\int\:\left[\:\mathrm{2}\left(\mathrm{1}+{tan}^{\mathrm{2}} {x}\right)+\mathrm{2}\left(\mathrm{1}+{tan}^{\mathrm{2}} {x}\right){tan}^{\mathrm{2}} {x}\:+\frac{\mathrm{2}{sinx}}{\left({cosx}\right)^{\mathrm{4}} }\:−\frac{\mathrm{1}}{{cos}^{\mathrm{2}} {x}}\right){dx} \\ $$

Commented by mohammad17 last updated on 17/Jul/20

how this sir

$${how}\:{this}\:{sir} \\ $$

Answered by mathmax by abdo last updated on 17/Jul/20

let f(a) =∫ (dx/(a−sinx)) we have f^′ (a) =−∫ (dx/((a−sinx)^2 )) ⇒ ∫ (dx/((a−sinx)^2 )) =−f^′ (a) and ∫ (dx/((1−sinx)^2 )) =−f^′ (1) let explicite f(a) changement tan((x/2))=t give f(a) =∫ ((2dt)/((1+t^2 )(a−((2t)/(1+t^2 ))))) =∫ ((2dt)/(a+at^2 −2t)) =∫ ((2dt)/(at^2 −2t +a)) Δ^′ =1−a^2 let take a>1 ⇒ Δ^′ <0 ⇒f(a) =(2/a)∫ (dt/(t^2 −((2t)/a) +1)) =(2/a)∫ (dt/(t^2 −2(t/a) +(1/a^2 ) +1−(1/a^2 ))) =(2/a)∫ (dt/((t−(1/a))^2 +((a^2 −1)/a^2 ))) =_(t−(1/a)=((√(a^2 −1))/a)u) (2/a)×(a^2 /(a^2 −1)) ∫ (1/(1+u^2 ))×((√(a^2 −1))/a)du =(2/(√(a^2 −1)))∫ (du/(1+u^2 )) =(2/(√(a^2 −1))) arctan(((at−1)/(√(a^2 −1)))) =(2/(√(a^2 −1))) arctan(((atan((x/2))−1)/(√(a^2 −1)))) +c rest to calculate f^′ (a) and lim_(a→1) f^′ (a) ...be continued...

$$\mathrm{let}\:\mathrm{f}\left(\mathrm{a}\right)\:=\int\:\:\frac{\mathrm{dx}}{\mathrm{a}−\mathrm{sinx}}\:\:\mathrm{we}\:\mathrm{have}\:\mathrm{f}^{'} \left(\mathrm{a}\right)\:=−\int\:\:\frac{\mathrm{dx}}{\left(\mathrm{a}−\mathrm{sinx}\right)^{\mathrm{2}} }\:\Rightarrow \\ $$$$\int\:\frac{\mathrm{dx}}{\left(\mathrm{a}−\mathrm{sinx}\right)^{\mathrm{2}} }\:=−\mathrm{f}^{'} \left(\mathrm{a}\right)\:\mathrm{and}\:\int\:\frac{\mathrm{dx}}{\left(\mathrm{1}−\mathrm{sinx}\right)^{\mathrm{2}} }\:=−\mathrm{f}^{'} \left(\mathrm{1}\right)\:\mathrm{let}\:\mathrm{explicite}\:\mathrm{f}\left(\mathrm{a}\right) \\ $$$$\mathrm{changement}\:\mathrm{tan}\left(\frac{\mathrm{x}}{\mathrm{2}}\right)=\mathrm{t}\:\mathrm{give}\:\mathrm{f}\left(\mathrm{a}\right)\:=\int\:\:\frac{\mathrm{2dt}}{\left(\mathrm{1}+\mathrm{t}^{\mathrm{2}} \right)\left(\mathrm{a}−\frac{\mathrm{2t}}{\mathrm{1}+\mathrm{t}^{\mathrm{2}} }\right)} \\ $$$$=\int\:\frac{\mathrm{2dt}}{\mathrm{a}+\mathrm{at}^{\mathrm{2}} −\mathrm{2t}}\:=\int\:\frac{\mathrm{2dt}}{\mathrm{at}^{\mathrm{2}} −\mathrm{2t}\:+\mathrm{a}} \\ $$$$\Delta^{'} \:=\mathrm{1}−\mathrm{a}^{\mathrm{2}} \:\mathrm{let}\:\mathrm{take}\:\mathrm{a}>\mathrm{1}\:\Rightarrow\:\Delta^{'} <\mathrm{0}\:\Rightarrow\mathrm{f}\left(\mathrm{a}\right)\:=\frac{\mathrm{2}}{\mathrm{a}}\int\:\frac{\mathrm{dt}}{\mathrm{t}^{\mathrm{2}} −\frac{\mathrm{2t}}{\mathrm{a}}\:+\mathrm{1}} \\ $$$$=\frac{\mathrm{2}}{\mathrm{a}}\int\:\:\frac{\mathrm{dt}}{\mathrm{t}^{\mathrm{2}} −\mathrm{2}\frac{\mathrm{t}}{\mathrm{a}}\:+\frac{\mathrm{1}}{\mathrm{a}^{\mathrm{2}} }\:+\mathrm{1}−\frac{\mathrm{1}}{\mathrm{a}^{\mathrm{2}} }}\:=\frac{\mathrm{2}}{\mathrm{a}}\int\:\:\frac{\mathrm{dt}}{\left(\mathrm{t}−\frac{\mathrm{1}}{\mathrm{a}}\right)^{\mathrm{2}} \:+\frac{\mathrm{a}^{\mathrm{2}} −\mathrm{1}}{\mathrm{a}^{\mathrm{2}} }} \\ $$$$=_{\mathrm{t}−\frac{\mathrm{1}}{\mathrm{a}}=\frac{\sqrt{\mathrm{a}^{\mathrm{2}} −\mathrm{1}}}{\mathrm{a}}\mathrm{u}} \:\:\:\:\frac{\mathrm{2}}{\mathrm{a}}×\frac{\mathrm{a}^{\mathrm{2}} }{\mathrm{a}^{\mathrm{2}} −\mathrm{1}}\:\int\:\:\frac{\mathrm{1}}{\mathrm{1}+\mathrm{u}^{\mathrm{2}} }×\frac{\sqrt{\mathrm{a}^{\mathrm{2}} −\mathrm{1}}}{\mathrm{a}}\mathrm{du} \\ $$$$=\frac{\mathrm{2}}{\sqrt{\mathrm{a}^{\mathrm{2}} −\mathrm{1}}}\int\:\frac{\mathrm{du}}{\mathrm{1}+\mathrm{u}^{\mathrm{2}} }\:=\frac{\mathrm{2}}{\sqrt{\mathrm{a}^{\mathrm{2}} −\mathrm{1}}}\:\mathrm{arctan}\left(\frac{\mathrm{at}−\mathrm{1}}{\sqrt{\mathrm{a}^{\mathrm{2}} −\mathrm{1}}}\right)\:=\frac{\mathrm{2}}{\sqrt{\mathrm{a}^{\mathrm{2}} −\mathrm{1}}}\:\mathrm{arctan}\left(\frac{\mathrm{atan}\left(\frac{\mathrm{x}}{\mathrm{2}}\right)−\mathrm{1}}{\sqrt{\mathrm{a}^{\mathrm{2}} −\mathrm{1}}}\right)\:+\mathrm{c} \\ $$$$\mathrm{rest}\:\mathrm{to}\:\mathrm{calculate}\:\mathrm{f}^{'} \left(\mathrm{a}\right)\:\mathrm{and}\:\mathrm{lim}_{\mathrm{a}\rightarrow\mathrm{1}} \mathrm{f}^{'} \left(\mathrm{a}\right)\:...\mathrm{be}\:\mathrm{continued}... \\ $$

Answered by Dwaipayan Shikari last updated on 17/Jul/20

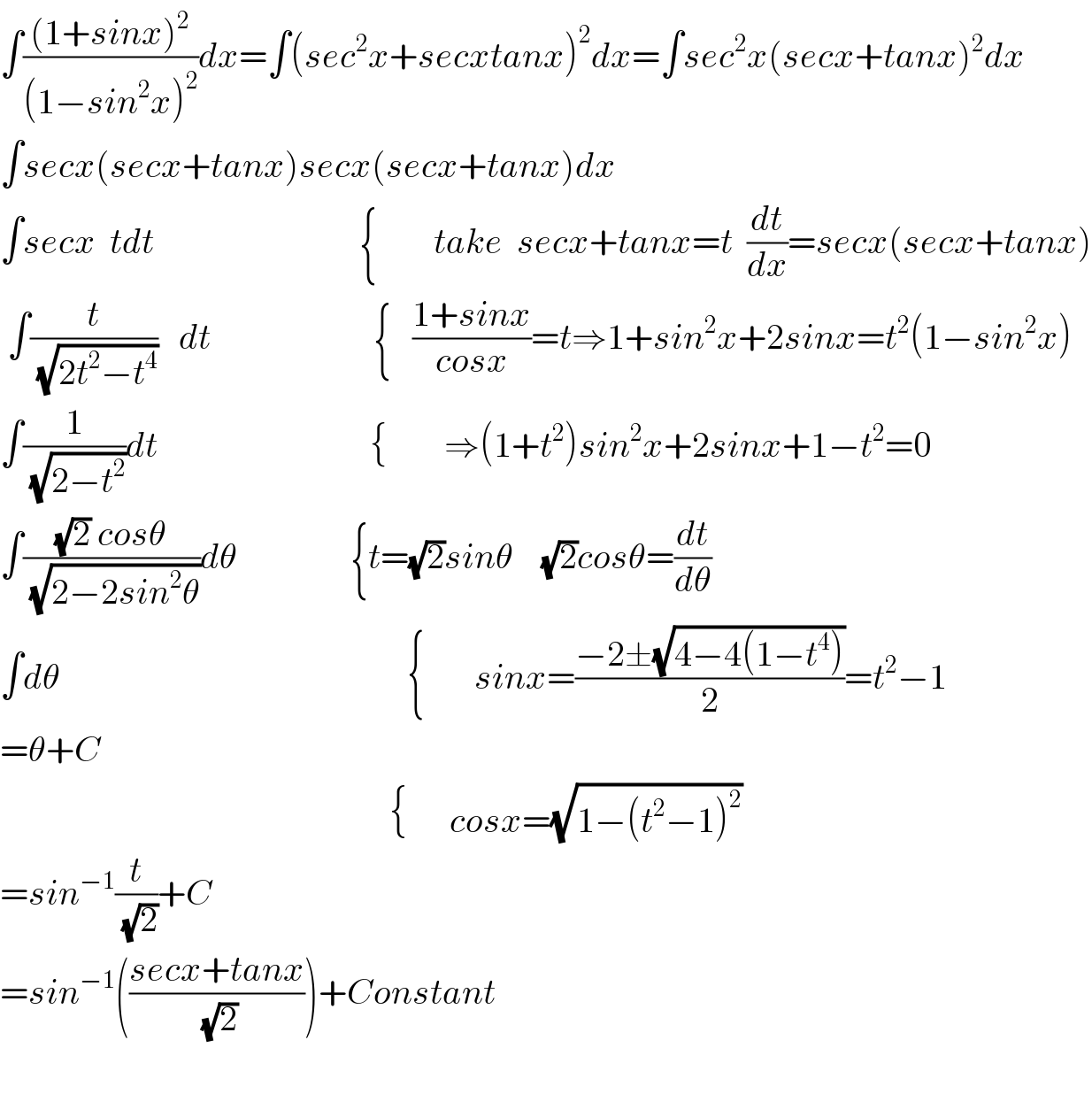
∫(((1+sinx)^2 )/((1−sin^2 x)^2 ))dx=∫(sec^2 x+secxtanx)^2 dx=∫sec^2 x(secx+tanx)^2 dx ∫secx(secx+tanx)secx(secx+tanx)dx ∫secx tdt { take secx+tanx=t (dt/dx)=secx(secx+tanx) ∫(t/(√(2t^2 −t^4 ))) dt { ((1+sinx)/(cosx))=t⇒1+sin^2 x+2sinx=t^2 (1−sin^2 x) ∫(1/(√(2−t^2 )))dt { ⇒(1+t^2 )sin^2 x+2sinx+1−t^2 =0 ∫(((√2) cosθ)/(√(2−2sin^2 θ)))dθ {t=(√2)sinθ (√2)cosθ=(dt/dθ) ∫dθ { sinx=((−2±(√(4−4(1−t^4 ))))/2)=t^2 −1 =θ+C { cosx=(√(1−(t^2 −1)^2 )) =sin^(−1) (t/(√2))+C =sin^(−1) (((secx+tanx)/(√2)))+Constant

$$\int\frac{\left(\mathrm{1}+{sinx}\right)^{\mathrm{2}} }{\left(\mathrm{1}−{sin}^{\mathrm{2}} {x}\right)^{\mathrm{2}} }{dx}=\int\left({sec}^{\mathrm{2}} {x}+{secxtanx}\right)^{\mathrm{2}} {dx}=\int{sec}^{\mathrm{2}} {x}\left({secx}+{tanx}\right)^{\mathrm{2}} {dx} \\ $$$$\int{secx}\left({secx}+{tanx}\right){secx}\left({secx}+{tanx}\right){dx} \\ $$$$\int{secx}\:\:{tdt}\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\left\{\:\:\:\:\:\:\:\:{take}\:\:{secx}+{tanx}={t}\:\:\frac{{dt}}{{dx}}={secx}\left({secx}+{tanx}\right)\right. \\ $$$$\:\int\frac{{t}}{\sqrt{\mathrm{2}{t}^{\mathrm{2}} −{t}^{\mathrm{4}} }}\:\:\:{dt}\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\left\{\:\:\:\frac{\mathrm{1}+{sinx}}{{cosx}}={t}\Rightarrow\mathrm{1}+{sin}^{\mathrm{2}} {x}+\mathrm{2}{sinx}={t}^{\mathrm{2}} \left(\mathrm{1}−{sin}^{\mathrm{2}} {x}\right)\right. \\ $$$$\int\frac{\mathrm{1}}{\sqrt{\mathrm{2}−{t}^{\mathrm{2}} }}{dt}\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\left\{\:\:\:\:\:\:\:\:\Rightarrow\left(\mathrm{1}+{t}^{\mathrm{2}} \right){sin}^{\mathrm{2}} {x}+\mathrm{2}{sinx}+\mathrm{1}−{t}^{\mathrm{2}} =\mathrm{0}\right. \\ $$$$\int\frac{\sqrt{\mathrm{2}}\:{cos}\theta}{\sqrt{\mathrm{2}−\mathrm{2}{sin}^{\mathrm{2}} \theta}}{d}\theta\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\left\{{t}=\sqrt{\mathrm{2}}{sin}\theta\:\:\:\:\sqrt{\mathrm{2}}{cos}\theta=\frac{{dt}}{{d}\theta}\right. \\ $$$$\int{d}\theta\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\left\{\:\:\:\:\:\:\:{sinx}=\frac{−\mathrm{2}\pm\sqrt{\mathrm{4}−\mathrm{4}\left(\mathrm{1}−{t}^{\mathrm{4}} \right)}}{\mathrm{2}}={t}^{\mathrm{2}} −\mathrm{1}\right. \\ $$$$=\theta+{C} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\left\{\:\:\:\:\:\:{cosx}=\sqrt{\mathrm{1}−\left({t}^{\mathrm{2}} −\mathrm{1}\right)^{\mathrm{2}} }\right. \\ $$$$={sin}^{−\mathrm{1}} \frac{{t}}{\sqrt{\mathrm{2}}}+{C} \\ $$$$={sin}^{−\mathrm{1}} \left(\frac{{secx}+{tanx}}{\sqrt{\mathrm{2}}}\right)+{Constant} \\ $$$$ \\ $$

Commented by mohammad17 last updated on 17/Jul/20

thank you sir

$${thank}\:{you}\:{sir} \\ $$

Commented by Dwaipayan Shikari last updated on 17/Jul/20

Kindly check it

$${Kindly}\:{check}\:{it} \\ $$

Commented by Dwaipayan Shikari last updated on 17/Jul/20

Commented by Dwaipayan Shikari last updated on 17/Jul/20

Alternate solution

$${Alternate}\:{solution} \\ $$

Answered by mathmax by abdo last updated on 17/Jul/20

let try another way I =∫ (dx/((1−sinx)^2 )) ⇒ I =∫ (dx/((1−cos((π/2)−x))^2 )) =∫ (dx/((2sin^2 ((π/4)−(x/2)))^4 )) =(1/(16))∫ (dx/(sin^4 ((π/4)−(x/2)))) =_((π/4)−(x/2)=t) (1/(16)) ∫ ((−2dt)/(sin^4 t)) =−(1/8)∫ (dt/(sin^4 t)) changement tan((t/2))=u give ∫ (dt/(sin^4 t)) =∫ ((2du)/((1+u^2 )(((2u)/(1+u^2 )))^4 )) =∫ ((2(1+u)^3 )/(16u^4 )) du =(1/8) ∫ ((u^3 +3u^2 +3u +1)/u^4 )du =(1/8)∫ ((1/u) +(3/u^2 ) +(3/u^3 ) +(1/u^4 ))du =(1/8){ ln∣u∣−(3/u) +3(−(1/(2u^2 ))) −(1/(3u^3 ))} +C ⇒ I =−(1/(64)){ ln∣tan((t/2))∣−(3/(tan((t/2)))) −(3/(2tan^2 ((t/2))))−(1/(3tan^3 ((t/2))))} +C =−(1/(64)){ ln∣tan((π/8)−(x/4))∣−(3/(tan((π/8)−(x/4)))) −(3/(2tan^2 ((π/8)−(x/4))))−(1/(3tan^3 ((π/8)−(x/4))))} +C

$$\mathrm{let}\:\mathrm{try}\:\mathrm{another}\:\mathrm{way}\: \\ $$$$\mathrm{I}\:=\int\:\:\frac{\mathrm{dx}}{\left(\mathrm{1}−\mathrm{sinx}\right)^{\mathrm{2}} }\:\Rightarrow\:\mathrm{I}\:=\int\:\frac{\mathrm{dx}}{\left(\mathrm{1}−\mathrm{cos}\left(\frac{\pi}{\mathrm{2}}−\mathrm{x}\right)\right)^{\mathrm{2}} }\:=\int\:\frac{\mathrm{dx}}{\left(\mathrm{2sin}^{\mathrm{2}} \left(\frac{\pi}{\mathrm{4}}−\frac{\mathrm{x}}{\mathrm{2}}\right)\right)^{\mathrm{4}} } \\ $$$$=\frac{\mathrm{1}}{\mathrm{16}}\int\:\:\frac{\mathrm{dx}}{\mathrm{sin}^{\mathrm{4}} \left(\frac{\pi}{\mathrm{4}}−\frac{\mathrm{x}}{\mathrm{2}}\right)}\:=_{\frac{\pi}{\mathrm{4}}−\frac{\mathrm{x}}{\mathrm{2}}=\mathrm{t}} \:\:\:\frac{\mathrm{1}}{\mathrm{16}}\:\int\:\:\frac{−\mathrm{2dt}}{\mathrm{sin}^{\mathrm{4}} \mathrm{t}}\:=−\frac{\mathrm{1}}{\mathrm{8}}\int\:\frac{\mathrm{dt}}{\mathrm{sin}^{\mathrm{4}} \mathrm{t}} \\ $$$$\mathrm{changement}\:\:\mathrm{tan}\left(\frac{\mathrm{t}}{\mathrm{2}}\right)=\mathrm{u}\:\mathrm{give}\:\int\:\frac{\mathrm{dt}}{\mathrm{sin}^{\mathrm{4}} \mathrm{t}}\:=\int\:\:\frac{\mathrm{2du}}{\left(\mathrm{1}+\mathrm{u}^{\mathrm{2}} \right)\left(\frac{\mathrm{2u}}{\mathrm{1}+\mathrm{u}^{\mathrm{2}} }\right)^{\mathrm{4}} } \\ $$$$=\int\:\frac{\mathrm{2}\left(\mathrm{1}+\mathrm{u}\right)^{\mathrm{3}} }{\mathrm{16u}^{\mathrm{4}} }\:\mathrm{du}\:=\frac{\mathrm{1}}{\mathrm{8}}\:\int\:\frac{\mathrm{u}^{\mathrm{3}} \:+\mathrm{3u}^{\mathrm{2}} \:+\mathrm{3u}\:+\mathrm{1}}{\mathrm{u}^{\mathrm{4}} }\mathrm{du} \\ $$$$=\frac{\mathrm{1}}{\mathrm{8}}\int\:\left(\frac{\mathrm{1}}{\mathrm{u}}\:+\frac{\mathrm{3}}{\mathrm{u}^{\mathrm{2}} }\:+\frac{\mathrm{3}}{\mathrm{u}^{\mathrm{3}} }\:+\frac{\mathrm{1}}{\mathrm{u}^{\mathrm{4}} }\right)\mathrm{du} \\ $$$$=\frac{\mathrm{1}}{\mathrm{8}}\left\{\:\mathrm{ln}\mid\mathrm{u}\mid−\frac{\mathrm{3}}{\mathrm{u}}\:+\mathrm{3}\left(−\frac{\mathrm{1}}{\mathrm{2u}^{\mathrm{2}} }\right)\:−\frac{\mathrm{1}}{\mathrm{3u}^{\mathrm{3}} }\right\}\:+\mathrm{C} \\ $$$$\Rightarrow\:\mathrm{I}\:=−\frac{\mathrm{1}}{\mathrm{64}}\left\{\:\mathrm{ln}\mid\mathrm{tan}\left(\frac{\mathrm{t}}{\mathrm{2}}\right)\mid−\frac{\mathrm{3}}{\mathrm{tan}\left(\frac{\mathrm{t}}{\mathrm{2}}\right)}\:−\frac{\mathrm{3}}{\mathrm{2tan}^{\mathrm{2}} \left(\frac{\mathrm{t}}{\mathrm{2}}\right)}−\frac{\mathrm{1}}{\mathrm{3tan}^{\mathrm{3}} \left(\frac{\mathrm{t}}{\mathrm{2}}\right)}\right\}\:+\mathrm{C} \\ $$$$=−\frac{\mathrm{1}}{\mathrm{64}}\left\{\:\mathrm{ln}\mid\mathrm{tan}\left(\frac{\pi}{\mathrm{8}}−\frac{\mathrm{x}}{\mathrm{4}}\right)\mid−\frac{\mathrm{3}}{\mathrm{tan}\left(\frac{\pi}{\mathrm{8}}−\frac{\mathrm{x}}{\mathrm{4}}\right)}\:−\frac{\mathrm{3}}{\mathrm{2tan}^{\mathrm{2}} \left(\frac{\pi}{\mathrm{8}}−\frac{\mathrm{x}}{\mathrm{4}}\right)}−\frac{\mathrm{1}}{\mathrm{3tan}^{\mathrm{3}} \left(\frac{\pi}{\mathrm{8}}−\frac{\mathrm{x}}{\mathrm{4}}\right)}\right\}\:+\mathrm{C} \\ $$

Commented by mathmax by abdo last updated on 17/Jul/20

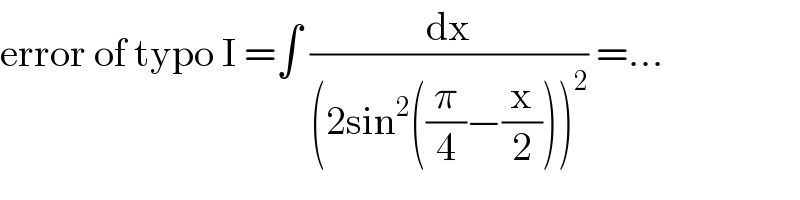
error of typo I =∫ (dx/((2sin^2 ((π/4)−(x/2)))^2 )) =...

$$\mathrm{error}\:\mathrm{of}\:\mathrm{typo}\:\mathrm{I}\:=\int\:\frac{\mathrm{dx}}{\left(\mathrm{2sin}^{\mathrm{2}} \left(\frac{\pi}{\mathrm{4}}−\frac{\mathrm{x}}{\mathrm{2}}\right)\right)^{\mathrm{2}} }\:=... \\ $$

Commented by mathmax by abdo last updated on 17/Jul/20

sorry change 64 by 32...

$$\mathrm{sorry}\:\mathrm{change}\:\mathrm{64}\:\mathrm{by}\:\mathrm{32}... \\ $$

Commented by mohammad17 last updated on 17/Jul/20

ok sir thank you

$${ok}\:{sir}\:{thank}\:{you} \\ $$

Answered by PRITHWISH SEN 2 last updated on 17/Jul/20

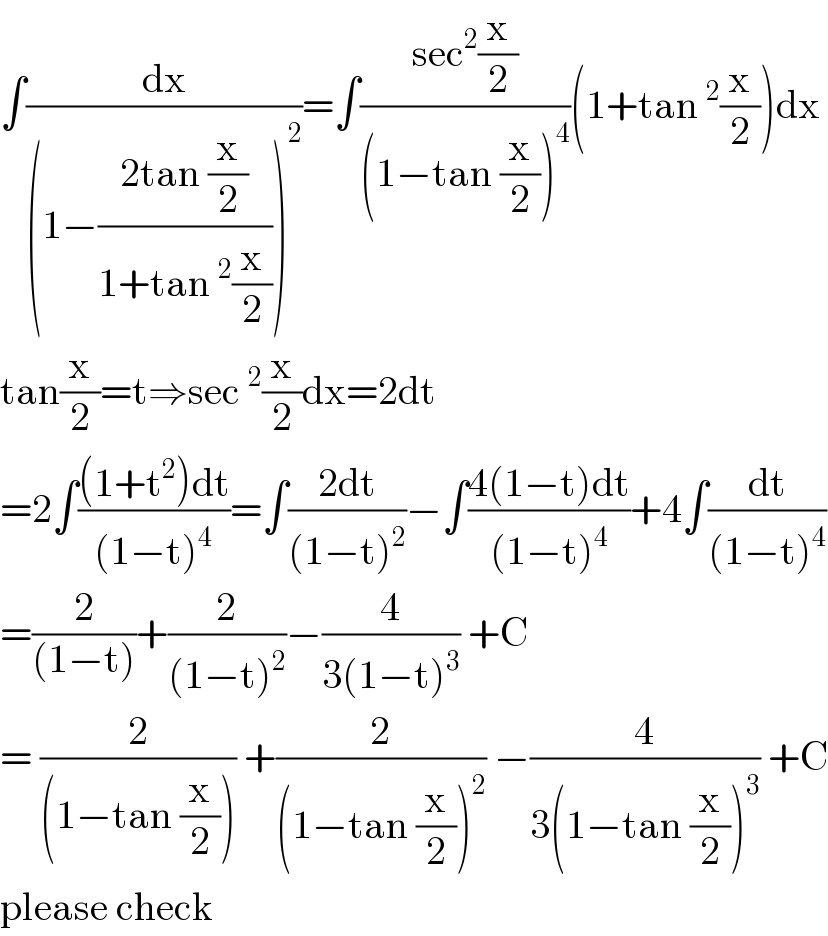
∫(dx/((1−((2tan (x/2))/(1+tan^2 (x/2))))^2 ))=∫((sec^2 (x/2))/((1−tan (x/2))^4 ))(1+tan^2 (x/2))dx tan(x/2)=t⇒sec^2 (x/2)dx=2dt =2∫(((1+t^2 )dt)/((1−t)^4 ))=∫((2dt)/((1−t)^2 ))−∫((4(1−t)dt)/((1−t)^4 ))+4∫(dt/((1−t)^4 )) =(2/((1−t)))+(2/((1−t)^2 ))−(4/(3(1−t)^3 )) +C = (2/((1−tan (x/2)))) +(2/((1−tan (x/2))^2 )) −(4/(3(1−tan (x/2))^3 )) +C please check

$$\int\frac{\mathrm{dx}}{\left(\mathrm{1}−\frac{\mathrm{2tan}\:\frac{\mathrm{x}}{\mathrm{2}}}{\mathrm{1}+\mathrm{tan}\:^{\mathrm{2}} \frac{\mathrm{x}}{\mathrm{2}}}\right)^{\mathrm{2}} }=\int\frac{\mathrm{sec}^{\mathrm{2}} \frac{\mathrm{x}}{\mathrm{2}}}{\left(\mathrm{1}−\mathrm{tan}\:\frac{\mathrm{x}}{\mathrm{2}}\right)^{\mathrm{4}} }\left(\mathrm{1}+\mathrm{tan}\:^{\mathrm{2}} \frac{\mathrm{x}}{\mathrm{2}}\right)\mathrm{dx} \\ $$$$\mathrm{tan}\frac{\mathrm{x}}{\mathrm{2}}=\mathrm{t}\Rightarrow\mathrm{sec}\:^{\mathrm{2}} \frac{\mathrm{x}}{\mathrm{2}}\mathrm{dx}=\mathrm{2dt} \\ $$$$=\mathrm{2}\int\frac{\left(\mathrm{1}+\mathrm{t}^{\mathrm{2}} \right)\mathrm{dt}}{\left(\mathrm{1}−\mathrm{t}\right)^{\mathrm{4}} }=\int\frac{\mathrm{2dt}}{\left(\mathrm{1}−\mathrm{t}\right)^{\mathrm{2}} }−\int\frac{\mathrm{4}\left(\mathrm{1}−\mathrm{t}\right)\mathrm{dt}}{\left(\mathrm{1}−\mathrm{t}\right)^{\mathrm{4}} }+\mathrm{4}\int\frac{\mathrm{dt}}{\left(\mathrm{1}−\mathrm{t}\right)^{\mathrm{4}} } \\ $$$$=\frac{\mathrm{2}}{\left(\mathrm{1}−\mathrm{t}\right)}+\frac{\mathrm{2}}{\left(\mathrm{1}−\mathrm{t}\right)^{\mathrm{2}} }−\frac{\mathrm{4}}{\mathrm{3}\left(\mathrm{1}−\mathrm{t}\right)^{\mathrm{3}} }\:+\mathrm{C} \\ $$$$=\:\frac{\mathrm{2}}{\left(\mathrm{1}−\mathrm{tan}\:\frac{\mathrm{x}}{\mathrm{2}}\right)}\:+\frac{\mathrm{2}}{\left(\mathrm{1}−\mathrm{tan}\:\frac{\mathrm{x}}{\mathrm{2}}\right)^{\mathrm{2}} }\:−\frac{\mathrm{4}}{\mathrm{3}\left(\mathrm{1}−\mathrm{tan}\:\frac{\mathrm{x}}{\mathrm{2}}\right)^{\mathrm{3}} }\:+\mathrm{C} \\ $$$$\mathrm{please}\:\mathrm{check} \\ $$

Commented by mohammad17 last updated on 17/Jul/20

than you sir

$${than}\:{you}\:{sir} \\ $$

Answered by Ar Brandon last updated on 19/Jul/20

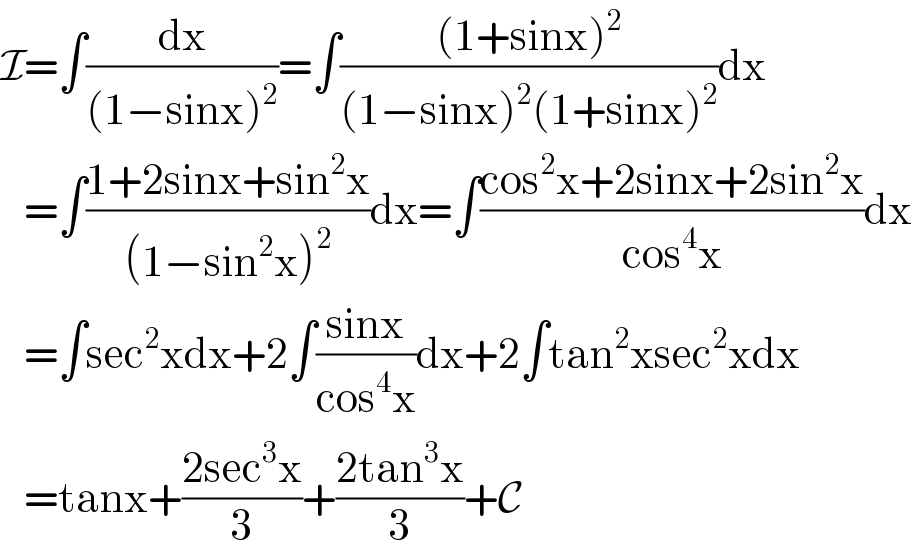
I=∫(dx/((1−sinx)^2 ))=∫(((1+sinx)^2 )/((1−sinx)^2 (1+sinx)^2 ))dx =∫((1+2sinx+sin^2 x)/((1−sin^2 x)^2 ))dx=∫((cos^2 x+2sinx+2sin^2 x)/(cos^4 x))dx =∫sec^2 xdx+2∫((sinx)/(cos^4 x))dx+2∫tan^2 xsec^2 xdx =tanx+((2sec^3 x)/3)+((2tan^3 x)/3)+C

$$\mathcal{I}=\int\frac{\mathrm{dx}}{\left(\mathrm{1}−\mathrm{sinx}\right)^{\mathrm{2}} }=\int\frac{\left(\mathrm{1}+\mathrm{sinx}\right)^{\mathrm{2}} }{\left(\mathrm{1}−\mathrm{sinx}\right)^{\mathrm{2}} \left(\mathrm{1}+\mathrm{sinx}\right)^{\mathrm{2}} }\mathrm{dx} \\ $$$$\:\:\:=\int\frac{\mathrm{1}+\mathrm{2sinx}+\mathrm{sin}^{\mathrm{2}} \mathrm{x}}{\left(\mathrm{1}−\mathrm{sin}^{\mathrm{2}} \mathrm{x}\right)^{\mathrm{2}} }\mathrm{dx}=\int\frac{\mathrm{cos}^{\mathrm{2}} \mathrm{x}+\mathrm{2sinx}+\mathrm{2sin}^{\mathrm{2}} \mathrm{x}}{\mathrm{cos}^{\mathrm{4}} \mathrm{x}}\mathrm{dx} \\ $$$$\:\:\:=\int\mathrm{sec}^{\mathrm{2}} \mathrm{xdx}+\mathrm{2}\int\frac{\mathrm{sinx}}{\mathrm{cos}^{\mathrm{4}} \mathrm{x}}\mathrm{dx}+\mathrm{2}\int\mathrm{tan}^{\mathrm{2}} \mathrm{xsec}^{\mathrm{2}} \mathrm{xdx} \\ $$$$\:\:\:=\mathrm{tanx}+\frac{\mathrm{2sec}^{\mathrm{3}} \mathrm{x}}{\mathrm{3}}+\frac{\mathrm{2tan}^{\mathrm{3}} \mathrm{x}}{\mathrm{3}}+\mathcal{C} \\ $$

Answered by Her_Majesty last updated on 07/Aug/20

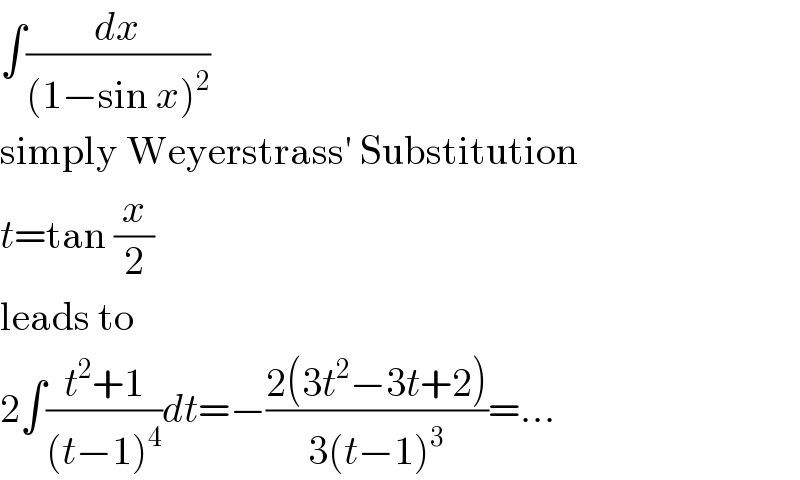
∫(dx/((1−sin x)^2 )) simply Weyerstrass′ Substitution t=tan (x/2) leads to 2∫((t^2 +1)/((t−1)^4 ))dt=−((2(3t^2 −3t+2))/(3(t−1)^3 ))=...

$$\int\frac{{dx}}{\left(\mathrm{1}−\mathrm{sin}\:{x}\right)^{\mathrm{2}} } \\ $$$$\mathrm{simply}\:\mathrm{Weyerstrass}'\:\mathrm{Substitution} \\ $$$${t}=\mathrm{tan}\:\frac{{x}}{\mathrm{2}} \\ $$$$\mathrm{leads}\:\mathrm{to} \\ $$$$\mathrm{2}\int\frac{{t}^{\mathrm{2}} +\mathrm{1}}{\left({t}−\mathrm{1}\right)^{\mathrm{4}} }{dt}=−\frac{\mathrm{2}\left(\mathrm{3}{t}^{\mathrm{2}} −\mathrm{3}{t}+\mathrm{2}\right)}{\mathrm{3}\left({t}−\mathrm{1}\right)^{\mathrm{3}} }=... \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com