Question and Answers Forum

All Questions      Topic List

Integration Questions

Previous in All Question      Next in All Question      

Previous in Integration      Next in Integration      

Question Number 104312 by Ar Brandon last updated on 20/Jul/20

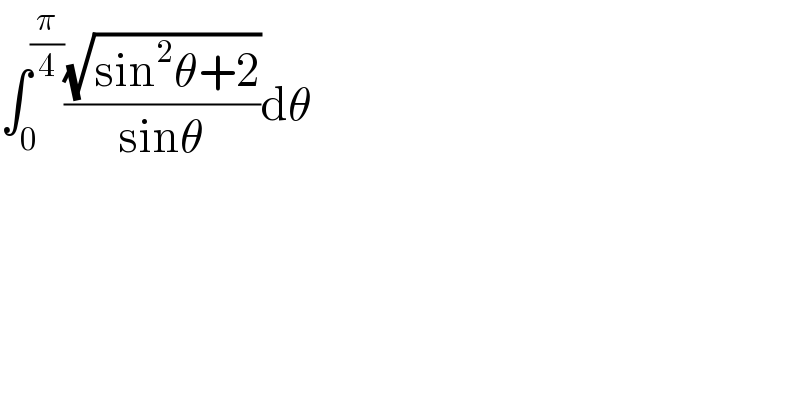
∫_0 ^(π/4) ((√(sin^2 θ+2))/(sinθ))dθ

$$\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{4}}} \frac{\sqrt{\mathrm{sin}^{\mathrm{2}} \theta+\mathrm{2}}}{\mathrm{sin}\theta}\mathrm{d}\theta \\ $$

Answered by mathmax by abdo last updated on 21/Jul/20

I =∫_0 ^(π/4) ((√(sin^2 θ+2))/(sinθ))dθ ⇒I =∫_0 ^(π/4) ((sinθ(√(1+(2/(sin^2 θ)))))/(sinθ))dθ =∫_0 ^(π/4) (√(1+(2/(1−cos^2 θ))))dθ we have 1+tan^2 θ =(1/(cos^2 θ)) ⇒cos^2 θ =(1/(1+tan^2 θ)) ⇒1−cos^2 θ=1−(1/(1+tan^2 θ)) =((tan^2 θ)/(1+tan^2 θ)) ⇒(2/(1−cos^2 θ)) =2×((1+tan^2 θ)/(tan^2 θ)) ⇒ I =∫_0 ^(π/4) (√(1+((2+2tan^2 θ)/(tan^2 θ))))dθ =∫_0 ^(π/4) (√((3tan^2 θ+2)/(tan^2 θ)))dθ =_(tanθ =x) =∫_0 ^1 (√((3x^2 +2)/x^2 )) (dx/(1+x^2 )) =∫_0 ^1 ((√(3x^2 +2))/(x(1+x^2 )))dx =(√3)∫_0 ^1 ((√(x^2 +(2/3)))/(x(1+x^2 )))dx cha7gement x =(√(2/3))shu give u =argsh((√(3/2))x) I =(√3)∫_0 ^(arhsh((√(3/2)))) (2/3)×((chu)/((√(2/3))sh(u)(1+(2/3)sh^2 u)))×(√(2/3))ch(u)du =2(√3)∫_0 ^(ln((√(3/2))+(√(1+(9/4))))) ((ch^2 u)/(shu(3+2sh^2 u)))du and this integral can be solved ...be continued...

$$\mathrm{I}\:=\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{4}}} \:\frac{\sqrt{\mathrm{sin}^{\mathrm{2}} \theta+\mathrm{2}}}{\mathrm{sin}\theta}\mathrm{d}\theta\:\Rightarrow\mathrm{I}\:=\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{4}}} \:\frac{\mathrm{sin}\theta\sqrt{\mathrm{1}+\frac{\mathrm{2}}{\mathrm{sin}^{\mathrm{2}} \theta}}}{\mathrm{sin}\theta}\mathrm{d}\theta\:=\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{4}}} \sqrt{\mathrm{1}+\frac{\mathrm{2}}{\mathrm{1}−\mathrm{cos}^{\mathrm{2}} \theta}}\mathrm{d}\theta \\ $$$$\mathrm{we}\:\mathrm{have}\:\mathrm{1}+\mathrm{tan}^{\mathrm{2}} \theta\:=\frac{\mathrm{1}}{\mathrm{cos}^{\mathrm{2}} \theta}\:\Rightarrow\mathrm{cos}^{\mathrm{2}} \theta\:=\frac{\mathrm{1}}{\mathrm{1}+\mathrm{tan}^{\mathrm{2}} \theta}\:\Rightarrow\mathrm{1}−\mathrm{cos}^{\mathrm{2}} \theta=\mathrm{1}−\frac{\mathrm{1}}{\mathrm{1}+\mathrm{tan}^{\mathrm{2}} \theta} \\ $$$$=\frac{\mathrm{tan}^{\mathrm{2}} \theta}{\mathrm{1}+\mathrm{tan}^{\mathrm{2}} \theta}\:\Rightarrow\frac{\mathrm{2}}{\mathrm{1}−\mathrm{cos}^{\mathrm{2}} \theta}\:=\mathrm{2}×\frac{\mathrm{1}+\mathrm{tan}^{\mathrm{2}} \theta}{\mathrm{tan}^{\mathrm{2}} \theta}\:\Rightarrow \\ $$$$\mathrm{I}\:=\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{4}}} \sqrt{\mathrm{1}+\frac{\mathrm{2}+\mathrm{2tan}^{\mathrm{2}} \theta}{\mathrm{tan}^{\mathrm{2}} \theta}}\mathrm{d}\theta\:\:=\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{4}}} \sqrt{\frac{\mathrm{3tan}^{\mathrm{2}} \theta+\mathrm{2}}{\mathrm{tan}^{\mathrm{2}} \theta}}\mathrm{d}\theta\:\:=_{\mathrm{tan}\theta\:=\mathrm{x}} \\ $$$$=\int_{\mathrm{0}} ^{\mathrm{1}} \sqrt{\frac{\mathrm{3x}^{\mathrm{2}} +\mathrm{2}}{\mathrm{x}^{\mathrm{2}} }}\:\frac{\mathrm{dx}}{\mathrm{1}+\mathrm{x}^{\mathrm{2}} }\:\:=\int_{\mathrm{0}} ^{\mathrm{1}} \:\:\frac{\sqrt{\mathrm{3x}^{\mathrm{2}} +\mathrm{2}}}{\mathrm{x}\left(\mathrm{1}+\mathrm{x}^{\mathrm{2}} \right)}\mathrm{dx}\:\:=\sqrt{\mathrm{3}}\int_{\mathrm{0}} ^{\mathrm{1}} \:\frac{\sqrt{\mathrm{x}^{\mathrm{2}} \:+\frac{\mathrm{2}}{\mathrm{3}}}}{\mathrm{x}\left(\mathrm{1}+\mathrm{x}^{\mathrm{2}} \right)}\mathrm{dx} \\ $$$$\mathrm{cha7gement}\:\mathrm{x}\:=\sqrt{\frac{\mathrm{2}}{\mathrm{3}}}\mathrm{shu}\:\mathrm{give}\:\mathrm{u}\:=\mathrm{argsh}\left(\sqrt{\frac{\mathrm{3}}{\mathrm{2}}}\mathrm{x}\right) \\ $$$$\mathrm{I}\:=\sqrt{\mathrm{3}}\int_{\mathrm{0}} ^{\mathrm{arhsh}\left(\sqrt{\frac{\mathrm{3}}{\mathrm{2}}}\right)} \:\:\frac{\mathrm{2}}{\mathrm{3}}×\frac{\mathrm{chu}}{\sqrt{\frac{\mathrm{2}}{\mathrm{3}}}\mathrm{sh}\left(\mathrm{u}\right)\left(\mathrm{1}+\frac{\mathrm{2}}{\mathrm{3}}\mathrm{sh}^{\mathrm{2}} \mathrm{u}\right)}×\sqrt{\frac{\mathrm{2}}{\mathrm{3}}}\mathrm{ch}\left(\mathrm{u}\right)\mathrm{du} \\ $$$$=\mathrm{2}\sqrt{\mathrm{3}}\int_{\mathrm{0}} ^{\mathrm{ln}\left(\sqrt{\frac{\mathrm{3}}{\mathrm{2}}}+\sqrt{\mathrm{1}+\frac{\mathrm{9}}{\mathrm{4}}}\right)} \:\:\:\frac{\mathrm{ch}^{\mathrm{2}} \mathrm{u}}{\mathrm{shu}\left(\mathrm{3}+\mathrm{2sh}^{\mathrm{2}} \mathrm{u}\right)}\mathrm{du}\:\:\:\:\mathrm{and}\:\mathrm{this}\:\mathrm{integral}\:\:\mathrm{can}\:\mathrm{be}\:\mathrm{solved} \\ $$$$...\mathrm{be}\:\mathrm{continued}... \\ $$

Commented by Ar Brandon last updated on 21/Jul/20

wow wow wow ! superb. Thanks so much ��

Commented by Ar Brandon last updated on 21/Jul/20

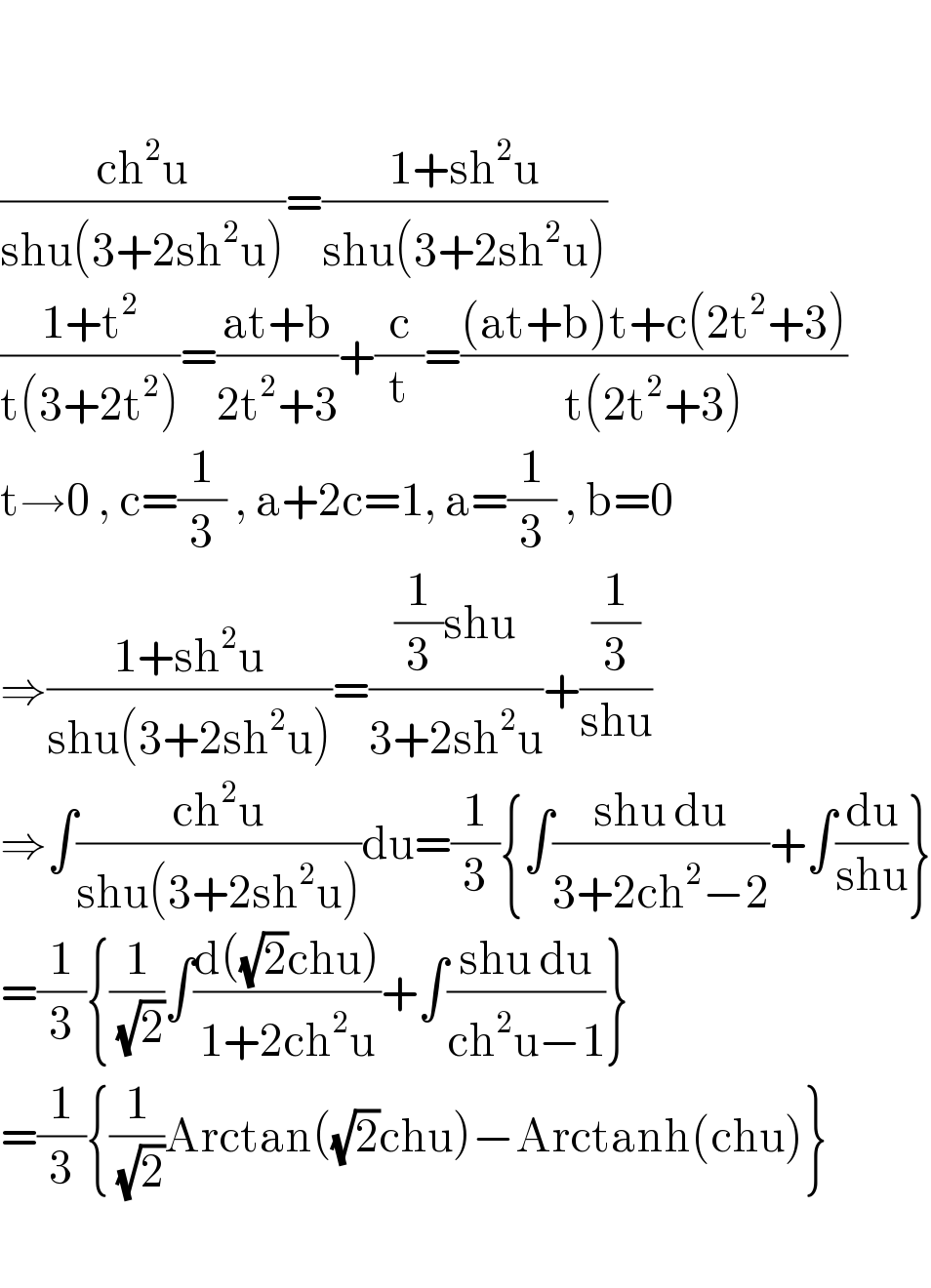
((ch^2 u)/(shu(3+2sh^2 u)))=((1+sh^2 u)/(shu(3+2sh^2 u))) ((1+t^2 )/(t(3+2t^2 )))=((at+b)/(2t^2 +3))+(c/t)=(((at+b)t+c(2t^2 +3))/(t(2t^2 +3))) t→0 , c=(1/3) , a+2c=1, a=(1/3) , b=0 ⇒((1+sh^2 u)/(shu(3+2sh^2 u)))=(((1/3)shu)/(3+2sh^2 u))+((1/3)/(shu)) ⇒∫((ch^2 u)/(shu(3+2sh^2 u)))du=(1/3){∫((shu du)/(3+2ch^2 −2))+∫(du/(shu))} =(1/3){(1/(√2))∫((d((√2)chu))/(1+2ch^2 u))+∫((shu du)/(ch^2 u−1))} =(1/3){(1/(√2))Arctan((√2)chu)−Arctanh(chu)}

$$ \\ $$$$ \\ $$$$\frac{\mathrm{ch}^{\mathrm{2}} \mathrm{u}}{\mathrm{shu}\left(\mathrm{3}+\mathrm{2sh}^{\mathrm{2}} \mathrm{u}\right)}=\frac{\mathrm{1}+\mathrm{sh}^{\mathrm{2}} \mathrm{u}}{\mathrm{shu}\left(\mathrm{3}+\mathrm{2sh}^{\mathrm{2}} \mathrm{u}\right)} \\ $$$$\frac{\mathrm{1}+\mathrm{t}^{\mathrm{2}} }{\mathrm{t}\left(\mathrm{3}+\mathrm{2t}^{\mathrm{2}} \right)}=\frac{\mathrm{at}+\mathrm{b}}{\mathrm{2t}^{\mathrm{2}} +\mathrm{3}}+\frac{\mathrm{c}}{\mathrm{t}}=\frac{\left(\mathrm{at}+\mathrm{b}\right)\mathrm{t}+\mathrm{c}\left(\mathrm{2t}^{\mathrm{2}} +\mathrm{3}\right)}{\mathrm{t}\left(\mathrm{2t}^{\mathrm{2}} +\mathrm{3}\right)} \\ $$$$\mathrm{t}\rightarrow\mathrm{0}\:,\:\mathrm{c}=\frac{\mathrm{1}}{\mathrm{3}}\:,\:\mathrm{a}+\mathrm{2c}=\mathrm{1},\:\mathrm{a}=\frac{\mathrm{1}}{\mathrm{3}}\:,\:\mathrm{b}=\mathrm{0} \\ $$$$\Rightarrow\frac{\mathrm{1}+\mathrm{sh}^{\mathrm{2}} \mathrm{u}}{\mathrm{shu}\left(\mathrm{3}+\mathrm{2sh}^{\mathrm{2}} \mathrm{u}\right)}=\frac{\frac{\mathrm{1}}{\mathrm{3}}\mathrm{shu}}{\mathrm{3}+\mathrm{2sh}^{\mathrm{2}} \mathrm{u}}+\frac{\frac{\mathrm{1}}{\mathrm{3}}}{\mathrm{shu}} \\ $$$$\Rightarrow\int\frac{\mathrm{ch}^{\mathrm{2}} \mathrm{u}}{\mathrm{shu}\left(\mathrm{3}+\mathrm{2sh}^{\mathrm{2}} \mathrm{u}\right)}\mathrm{du}=\frac{\mathrm{1}}{\mathrm{3}}\left\{\int\frac{\mathrm{shu}\:\mathrm{du}}{\mathrm{3}+\mathrm{2ch}^{\mathrm{2}} −\mathrm{2}}+\int\frac{\mathrm{du}}{\mathrm{shu}}\right\} \\ $$$$=\frac{\mathrm{1}}{\mathrm{3}}\left\{\frac{\mathrm{1}}{\sqrt{\mathrm{2}}}\int\frac{\mathrm{d}\left(\sqrt{\mathrm{2}}\mathrm{chu}\right)}{\mathrm{1}+\mathrm{2ch}^{\mathrm{2}} \mathrm{u}}+\int\frac{\mathrm{shu}\:\mathrm{du}}{\mathrm{ch}^{\mathrm{2}} \mathrm{u}−\mathrm{1}}\right\} \\ $$$$=\frac{\mathrm{1}}{\mathrm{3}}\left\{\frac{\mathrm{1}}{\sqrt{\mathrm{2}}}\mathrm{Arctan}\left(\sqrt{\mathrm{2}}\mathrm{chu}\right)−\mathrm{Arctanh}\left(\mathrm{chu}\right)\right\} \\ $$$$ \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com