Question and Answers Forum

All Questions      Topic List

Arithmetic Questions

Previous in All Question      Next in All Question      

Previous in Arithmetic      Next in Arithmetic      

Question Number 104428 by bemath last updated on 21/Jul/20

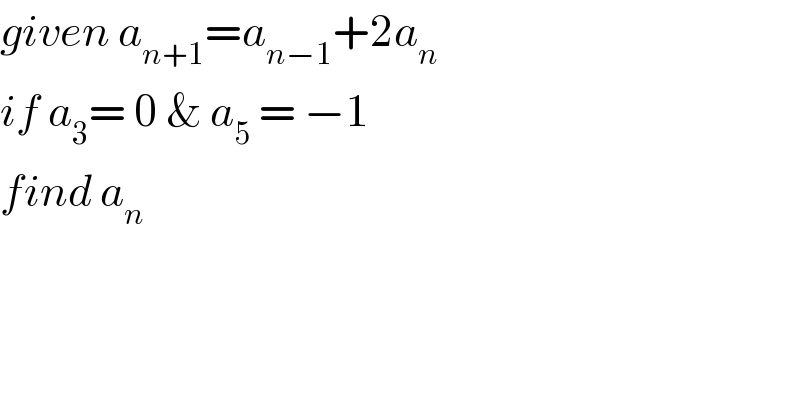
given a_(n+1) =a_(n−1) +2a_n if a_3 = 0 & a_5 = −1 find a_n

$${given}\:{a}_{{n}+\mathrm{1}} ={a}_{{n}−\mathrm{1}} +\mathrm{2}{a}_{{n}} \\ $$ $${if}\:{a}_{\mathrm{3}} =\:\mathrm{0}\:\&\:{a}_{\mathrm{5}} \:=\:−\mathrm{1} \\ $$ $${find}\:{a}_{{n}} \\ $$

Answered by Dwaipayan Shikari last updated on 21/Jul/20

Put n=4 a_5 =a_3 +2a_4 −1=2a_4 a_4 =−(1/2)

$$\mathrm{Put}\:\mathrm{n}=\mathrm{4} \\ $$ $$\mathrm{a}_{\mathrm{5}} =\mathrm{a}_{\mathrm{3}} +\mathrm{2a}_{\mathrm{4}} \\ $$ $$−\mathrm{1}=\mathrm{2a}_{\mathrm{4}} \\ $$ $$\mathrm{a}_{\mathrm{4}} =−\frac{\mathrm{1}}{\mathrm{2}} \\ $$

Commented bybemath last updated on 21/Jul/20

a_n ?

$${a}_{{n}} \:? \\ $$

Commented bybemath last updated on 21/Jul/20

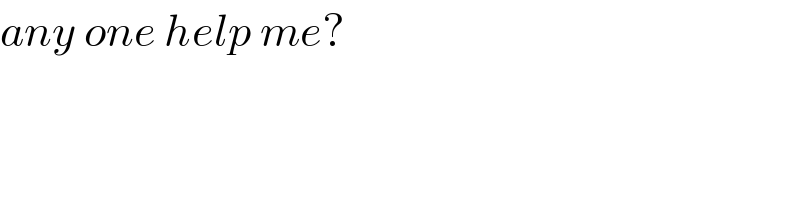
any one help me?

$${any}\:{one}\:{help}\:{me}? \\ $$

Answered by mathmax by abdo last updated on 22/Jul/20

a_(n+1) =a_(n−1) +2a_n ⇒a_(n+2) =a_(n ) +2a_(n+1) ⇒a_(n+2) −2a_(n+1) −a_n =0 caracteristic equation r^2 −2r−1 =0 ⇒r^2 −2r+1−2=0 ⇒ (r−1)^2 −((√2))^2 =0 ⇒(r−1−(√2))(r−1+(√2))=0 ⇒r =1+(√2)or r =1−(√2) a_n =α(1+(√2))^n +β(1−(√2))^n a_3 =α(1+(√2))^3 +β(1−(√2))^3 =0 and a_5 =α(1+(√2))^5 +β(1−(√2))^5 =−1 ⇒ we get the system { (((1+(√2))^3 α +(1−(√2))^3 β =0)),(((1+(√2))^5 α +(1−(√2))^5 β=−1)) :} Δ_s =(1+(√2))^3 (1−(√2))^5 −(1+(√2))^5 (1−(√2))^3 =(1+(√2))^3 (1−(√2))^3 (1−(√2))^2 −(1+(√2))^2 (1−(√2))^3 (1+(√2))^3 =−(1−(√2))^2 +(1+(√2))^2 =3+2(√2)−(3−2(√2)) =4(√2)≠0 ⇒ α =(Δ_α /(Δs)) =( determinant (((o (1−(√2))^3 )),((−1 (1−(√2))^5 )))/(4(√2))) =(((1−(√2))^3 )/(4(√2))) β =(Δ_β /Δ_s ) =( determinant ((((1+(√2))^3 0)),(((1+(√2))^5 −1)))/(4(√2))) =−(((1+(√2))^3 )/(4(√2))) ⇒ a_n =(((1−(√2))^3 )/(4(√2)))(1+(√2))^n −(((1+(√2))^3 )/(4(√2)))(1−(√2))^n

$$\mathrm{a}_{\mathrm{n}+\mathrm{1}} =\mathrm{a}_{\mathrm{n}−\mathrm{1}} \:+\mathrm{2a}_{\mathrm{n}} \:\Rightarrow\mathrm{a}_{\mathrm{n}+\mathrm{2}} \:=\mathrm{a}_{\mathrm{n}\:} \:+\mathrm{2a}_{\mathrm{n}+\mathrm{1}} \:\Rightarrow\mathrm{a}_{\mathrm{n}+\mathrm{2}} −\mathrm{2a}_{\mathrm{n}+\mathrm{1}} −\mathrm{a}_{\mathrm{n}} =\mathrm{0} \\ $$ $$\mathrm{caracteristic}\:\mathrm{equation}\:\mathrm{r}^{\mathrm{2}} −\mathrm{2r}−\mathrm{1}\:=\mathrm{0}\:\Rightarrow\mathrm{r}^{\mathrm{2}} −\mathrm{2r}+\mathrm{1}−\mathrm{2}=\mathrm{0}\:\Rightarrow \\ $$ $$\left(\mathrm{r}−\mathrm{1}\right)^{\mathrm{2}} −\left(\sqrt{\mathrm{2}}\right)^{\mathrm{2}} \:=\mathrm{0}\:\Rightarrow\left(\mathrm{r}−\mathrm{1}−\sqrt{\mathrm{2}}\right)\left(\mathrm{r}−\mathrm{1}+\sqrt{\mathrm{2}}\right)=\mathrm{0}\:\Rightarrow\mathrm{r}\:=\mathrm{1}+\sqrt{\mathrm{2}}\mathrm{or}\:\mathrm{r}\:=\mathrm{1}−\sqrt{\mathrm{2}} \\ $$ $$\mathrm{a}_{\mathrm{n}} =\alpha\left(\mathrm{1}+\sqrt{\mathrm{2}}\right)^{\mathrm{n}} \:+\beta\left(\mathrm{1}−\sqrt{\mathrm{2}}\right)^{\mathrm{n}} \\ $$ $$\mathrm{a}_{\mathrm{3}} =\alpha\left(\mathrm{1}+\sqrt{\mathrm{2}}\right)^{\mathrm{3}} +\beta\left(\mathrm{1}−\sqrt{\mathrm{2}}\right)^{\mathrm{3}} \:=\mathrm{0}\:\mathrm{and}\:\mathrm{a}_{\mathrm{5}} =\alpha\left(\mathrm{1}+\sqrt{\mathrm{2}}\right)^{\mathrm{5}} \:+\beta\left(\mathrm{1}−\sqrt{\mathrm{2}}\right)^{\mathrm{5}} =−\mathrm{1}\:\Rightarrow \\ $$ $$\mathrm{we}\:\mathrm{get}\:\mathrm{the}\:\mathrm{system}\:\:\:\begin{cases}{\left(\mathrm{1}+\sqrt{\mathrm{2}}\right)^{\mathrm{3}} \:\alpha\:+\left(\mathrm{1}−\sqrt{\mathrm{2}}\right)^{\mathrm{3}} \beta\:=\mathrm{0}}\\{\left(\mathrm{1}+\sqrt{\mathrm{2}}\right)^{\mathrm{5}} \:\alpha\:+\left(\mathrm{1}−\sqrt{\mathrm{2}}\right)^{\mathrm{5}} \:\beta=−\mathrm{1}}\end{cases} \\ $$ $$\Delta_{\mathrm{s}} =\left(\mathrm{1}+\sqrt{\mathrm{2}}\right)^{\mathrm{3}} \left(\mathrm{1}−\sqrt{\mathrm{2}}\right)^{\mathrm{5}} \:−\left(\mathrm{1}+\sqrt{\mathrm{2}}\right)^{\mathrm{5}} \left(\mathrm{1}−\sqrt{\mathrm{2}}\right)^{\mathrm{3}} \\ $$ $$=\left(\mathrm{1}+\sqrt{\mathrm{2}}\right)^{\mathrm{3}} \left(\mathrm{1}−\sqrt{\mathrm{2}}\right)^{\mathrm{3}} \left(\mathrm{1}−\sqrt{\mathrm{2}}\right)^{\mathrm{2}} \:−\left(\mathrm{1}+\sqrt{\mathrm{2}}\right)^{\mathrm{2}} \left(\mathrm{1}−\sqrt{\mathrm{2}}\right)^{\mathrm{3}} \left(\mathrm{1}+\sqrt{\mathrm{2}}\right)^{\mathrm{3}} \\ $$ $$=−\left(\mathrm{1}−\sqrt{\mathrm{2}}\right)^{\mathrm{2}} \:\:+\left(\mathrm{1}+\sqrt{\mathrm{2}}\right)^{\mathrm{2}} \:=\mathrm{3}+\mathrm{2}\sqrt{\mathrm{2}}−\left(\mathrm{3}−\mathrm{2}\sqrt{\mathrm{2}}\right)\:=\mathrm{4}\sqrt{\mathrm{2}}\neq\mathrm{0}\:\Rightarrow \\ $$ $$\alpha\:=\frac{\Delta_{\alpha} }{\Delta\mathrm{s}}\:=\frac{\begin{vmatrix}{\mathrm{o}\:\:\:\:\:\:\:\:\:\:\:\:\left(\mathrm{1}−\sqrt{\mathrm{2}}\right)^{\mathrm{3}} }\\{−\mathrm{1}\:\:\:\:\:\:\:\:\:\:\:\:\:\left(\mathrm{1}−\sqrt{\mathrm{2}}\right)^{\mathrm{5}} }\end{vmatrix}}{\mathrm{4}\sqrt{\mathrm{2}}}\:=\frac{\left(\mathrm{1}−\sqrt{\mathrm{2}}\right)^{\mathrm{3}} }{\mathrm{4}\sqrt{\mathrm{2}}} \\ $$ $$\beta\:=\frac{\Delta_{\beta} }{\Delta_{\mathrm{s}} }\:\:=\frac{\begin{vmatrix}{\left(\mathrm{1}+\sqrt{\mathrm{2}}\right)^{\mathrm{3}} \:\:\:\:\:\:\:\:\:\:\mathrm{0}}\\{\left(\mathrm{1}+\sqrt{\mathrm{2}}\right)^{\mathrm{5}} \:\:\:\:\:\:\:\:\:\:−\mathrm{1}}\end{vmatrix}}{\mathrm{4}\sqrt{\mathrm{2}}}\:=−\frac{\left(\mathrm{1}+\sqrt{\mathrm{2}}\right)^{\mathrm{3}} }{\mathrm{4}\sqrt{\mathrm{2}}} \\ $$ $$\Rightarrow\:\mathrm{a}_{\mathrm{n}} =\frac{\left(\mathrm{1}−\sqrt{\mathrm{2}}\right)^{\mathrm{3}} }{\mathrm{4}\sqrt{\mathrm{2}}}\left(\mathrm{1}+\sqrt{\mathrm{2}}\right)^{\mathrm{n}} \:−\frac{\left(\mathrm{1}+\sqrt{\mathrm{2}}\right)^{\mathrm{3}} }{\mathrm{4}\sqrt{\mathrm{2}}}\left(\mathrm{1}−\sqrt{\mathrm{2}}\right)^{\mathrm{n}} \\ $$

Commented bybemath last updated on 22/Jul/20

thank you sir

$${thank}\:{you}\:{sir} \\ $$

Answered by john santu last updated on 21/Jul/20

Commented bybemath last updated on 21/Jul/20

wow...

$${wow}... \\ $$

Commented bybemath last updated on 21/Jul/20

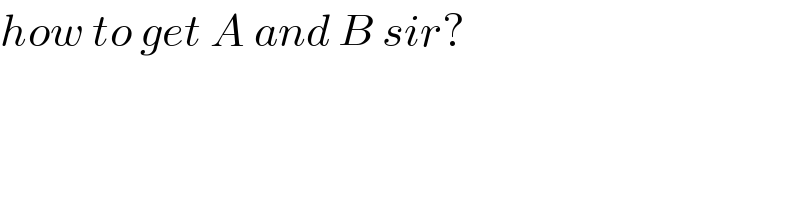
how to get A and B sir?

$${how}\:{to}\:{get}\:{A}\:{and}\:{B}\:{sir}? \\ $$

Answered by mr W last updated on 21/Jul/20

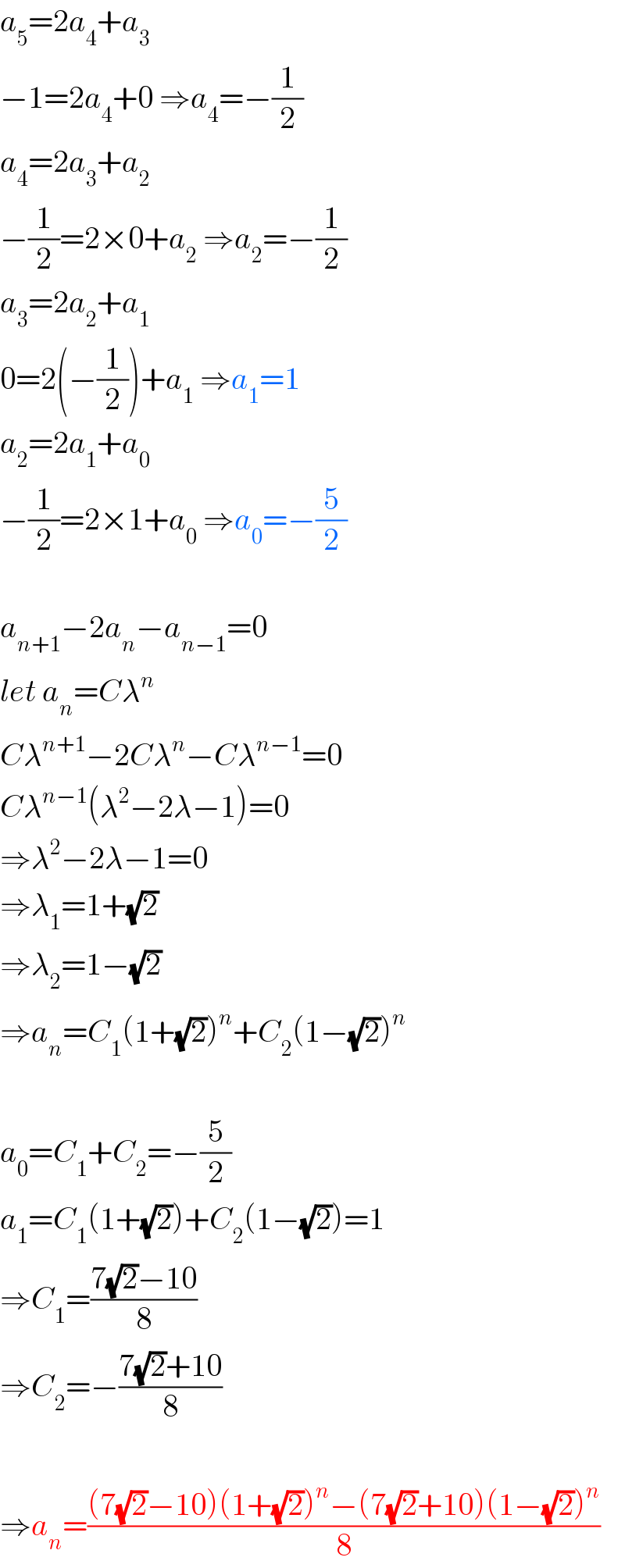
a_5 =2a_4 +a_3 −1=2a_4 +0 ⇒a_4 =−(1/2) a_4 =2a_3 +a_2 −(1/2)=2×0+a_2 ⇒a_2 =−(1/2) a_3 =2a_2 +a_1 0=2(−(1/2))+a_1 ⇒a_1 =1 a_2 =2a_1 +a_0 −(1/2)=2×1+a_0 ⇒a_0 =−(5/2) a_(n+1) −2a_n −a_(n−1) =0 let a_n =Cλ^n Cλ^(n+1) −2Cλ^n −Cλ^(n−1) =0 Cλ^(n−1) (λ^2 −2λ−1)=0 ⇒λ^2 −2λ−1=0 ⇒λ_1 =1+(√2) ⇒λ_2 =1−(√2) ⇒a_n =C_1 (1+(√2))^n +C_2 (1−(√2))^n a_0 =C_1 +C_2 =−(5/2) a_1 =C_1 (1+(√2))+C_2 (1−(√2))=1 ⇒C_1 =((7(√2)−10)/8) ⇒C_2 =−((7(√2)+10)/8) ⇒a_n =(((7(√2)−10)(1+(√2))^n −(7(√2)+10)(1−(√2))^n )/8)

$${a}_{\mathrm{5}} =\mathrm{2}{a}_{\mathrm{4}} +{a}_{\mathrm{3}} \\ $$ $$−\mathrm{1}=\mathrm{2}{a}_{\mathrm{4}} +\mathrm{0}\:\Rightarrow{a}_{\mathrm{4}} =−\frac{\mathrm{1}}{\mathrm{2}} \\ $$ $${a}_{\mathrm{4}} =\mathrm{2}{a}_{\mathrm{3}} +{a}_{\mathrm{2}} \\ $$ $$−\frac{\mathrm{1}}{\mathrm{2}}=\mathrm{2}×\mathrm{0}+{a}_{\mathrm{2}} \:\Rightarrow{a}_{\mathrm{2}} =−\frac{\mathrm{1}}{\mathrm{2}} \\ $$ $${a}_{\mathrm{3}} =\mathrm{2}{a}_{\mathrm{2}} +{a}_{\mathrm{1}} \\ $$ $$\mathrm{0}=\mathrm{2}\left(−\frac{\mathrm{1}}{\mathrm{2}}\right)+{a}_{\mathrm{1}} \:\Rightarrow{a}_{\mathrm{1}} =\mathrm{1} \\ $$ $${a}_{\mathrm{2}} =\mathrm{2}{a}_{\mathrm{1}} +{a}_{\mathrm{0}} \\ $$ $$−\frac{\mathrm{1}}{\mathrm{2}}=\mathrm{2}×\mathrm{1}+{a}_{\mathrm{0}} \:\Rightarrow{a}_{\mathrm{0}} =−\frac{\mathrm{5}}{\mathrm{2}} \\ $$ $$ \\ $$ $${a}_{{n}+\mathrm{1}} −\mathrm{2}{a}_{{n}} −{a}_{{n}−\mathrm{1}} =\mathrm{0} \\ $$ $${let}\:{a}_{{n}} ={C}\lambda^{{n}} \\ $$ $${C}\lambda^{{n}+\mathrm{1}} −\mathrm{2}{C}\lambda^{{n}} −{C}\lambda^{{n}−\mathrm{1}} =\mathrm{0} \\ $$ $${C}\lambda^{{n}−\mathrm{1}} \left(\lambda^{\mathrm{2}} −\mathrm{2}\lambda−\mathrm{1}\right)=\mathrm{0} \\ $$ $$\Rightarrow\lambda^{\mathrm{2}} −\mathrm{2}\lambda−\mathrm{1}=\mathrm{0} \\ $$ $$\Rightarrow\lambda_{\mathrm{1}} =\mathrm{1}+\sqrt{\mathrm{2}} \\ $$ $$\Rightarrow\lambda_{\mathrm{2}} =\mathrm{1}−\sqrt{\mathrm{2}} \\ $$ $$\Rightarrow{a}_{{n}} ={C}_{\mathrm{1}} \left(\mathrm{1}+\sqrt{\mathrm{2}}\right)^{{n}} +{C}_{\mathrm{2}} \left(\mathrm{1}−\sqrt{\mathrm{2}}\right)^{{n}} \\ $$ $$ \\ $$ $${a}_{\mathrm{0}} ={C}_{\mathrm{1}} +{C}_{\mathrm{2}} =−\frac{\mathrm{5}}{\mathrm{2}} \\ $$ $${a}_{\mathrm{1}} ={C}_{\mathrm{1}} \left(\mathrm{1}+\sqrt{\mathrm{2}}\right)+{C}_{\mathrm{2}} \left(\mathrm{1}−\sqrt{\mathrm{2}}\right)=\mathrm{1} \\ $$ $$\Rightarrow{C}_{\mathrm{1}} =\frac{\mathrm{7}\sqrt{\mathrm{2}}−\mathrm{10}}{\mathrm{8}} \\ $$ $$\Rightarrow{C}_{\mathrm{2}} =−\frac{\mathrm{7}\sqrt{\mathrm{2}}+\mathrm{10}}{\mathrm{8}} \\ $$ $$ \\ $$ $$\Rightarrow{a}_{{n}} =\frac{\left(\mathrm{7}\sqrt{\mathrm{2}}−\mathrm{10}\right)\left(\mathrm{1}+\sqrt{\mathrm{2}}\right)^{{n}} −\left(\mathrm{7}\sqrt{\mathrm{2}}+\mathrm{10}\right)\left(\mathrm{1}−\sqrt{\mathrm{2}}\right)^{{n}} }{\mathrm{8}} \\ $$

Commented bybemath last updated on 22/Jul/20

thank you sir

$${thank}\:{you}\:{sir} \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com