Question and Answers Forum

All Questions      Topic List

Algebra Questions

Previous in All Question      Next in All Question      

Previous in Algebra      Next in Algebra      

Question Number 105456 by 1549442205PVT last updated on 29/Jul/20

Prove that the curve y=x^4 +3x^2 +2x does not meet the straight line y=2x−1 and find the distace between their nearest points.(Answer (1/(√5)))

$$\mathrm{Prove}\:\mathrm{that}\:\mathrm{the}\:\mathrm{curve}\:\mathrm{y}=\mathrm{x}^{\mathrm{4}} +\mathrm{3x}^{\mathrm{2}} +\mathrm{2x} \\ $$$$\mathrm{does}\:\mathrm{not}\:\mathrm{meet}\:\mathrm{the}\:\mathrm{straight}\:\mathrm{line} \\ $$$$\mathrm{y}=\mathrm{2x}−\mathrm{1}\:\mathrm{and}\:\mathrm{find}\:\mathrm{the}\:\mathrm{distace}\:\mathrm{between} \\ $$$$\mathrm{their}\:\mathrm{nearest}\:\mathrm{points}.\left(\mathrm{Answer}\:\frac{\mathrm{1}}{\sqrt{\mathrm{5}}}\right) \\ $$

Answered by ajfour last updated on 29/Jul/20

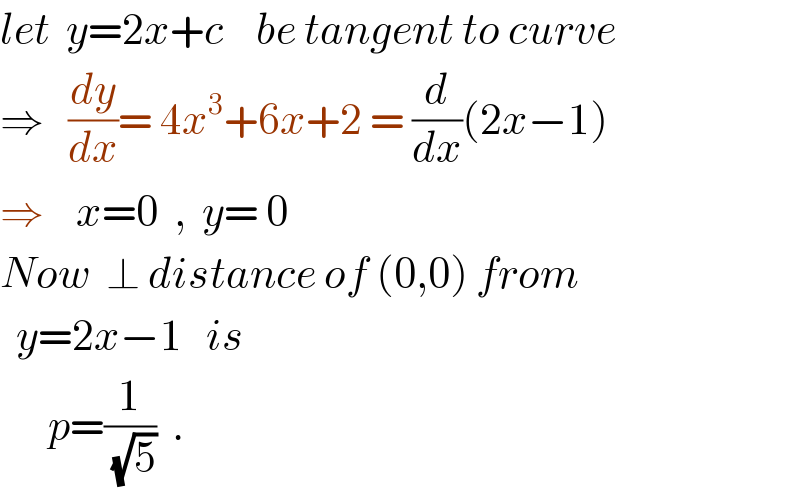
let y=2x+c be tangent to curve ⇒ (dy/dx)= 4x^3 +6x+2 = (d/dx)(2x−1) ⇒ x=0 , y= 0 Now ⊥ distance of (0,0) from y=2x−1 is p=(1/(√5)) .

$${let}\:\:{y}=\mathrm{2}{x}+{c}\:\:\:\:{be}\:{tangent}\:{to}\:{curve} \\ $$$$\Rightarrow\:\:\:\frac{{dy}}{{dx}}=\:\mathrm{4}{x}^{\mathrm{3}} +\mathrm{6}{x}+\mathrm{2}\:=\:\frac{{d}}{{dx}}\left(\mathrm{2}{x}−\mathrm{1}\right) \\ $$$$\Rightarrow\:\:\:\:{x}=\mathrm{0}\:\:,\:\:{y}=\:\mathrm{0} \\ $$$${Now}\:\:\bot\:{distance}\:{of}\:\left(\mathrm{0},\mathrm{0}\right)\:{from} \\ $$$$\:\:{y}=\mathrm{2}{x}−\mathrm{1}\:\:\:{is} \\ $$$$\:\:\:\:\:\:{p}=\frac{\mathrm{1}}{\sqrt{\mathrm{5}}}\:\:. \\ $$

Commented by Ari last updated on 29/Jul/20

Sir, if you can, clarify through actions your reasoning that there is ambiguity

Commented by 1549442205PVT last updated on 29/Jul/20

Thank you both sir.

$$\mathrm{Thank}\:\mathrm{you}\:\mathrm{both}\:\mathrm{sir}. \\ $$

Answered by 1549442205PVT last updated on 29/Jul/20

i)Abscissa of intersection point of the curve y=x^(4 ) +3x^(2 ) +2x (C)and the line y=2x−1(L) must be a real root of the equation: x^(4 ) +3x^(2 ) +2x=2x−1⇔x^4 +3x^2 +1=0 But this equation has no real roots simce x^4 +3x^2 +1≥1∀x∈R.Hence the curve (C)don′t meet the line(L)(q.e.d) ii)Denote by A(x_0 ,y_0 )be a point at which the tangent of the curve parallel to the line y=2x+1 .Then (x^(4 ) +3x^(2 ) +2x)^′ ∣_x_0 =2⇔4x_0 ^3 +6x_0 +2=2 ⇔2x_0 (2x_0 ^2 +3)=0⇔x_0 =0⇒y_0 =0.Then the nearest distance between the curve and the line be the distance between A(x_0 ,y_0 ) and the line y=2x−1which means d_0 =((∣2.0−1.0∣)/(√(2^2 +1^2 )))=(1/(√5))

$$\left.\mathrm{i}\right)\mathrm{Abscissa}\:\mathrm{of}\:\mathrm{intersection}\:\mathrm{point}\:\mathrm{of}\:\mathrm{the}\:\mathrm{curve} \\ $$$$\mathrm{y}=\mathrm{x}^{\mathrm{4}\:} +\mathrm{3x}^{\mathrm{2}\:\:} +\mathrm{2x}\:\left(\mathrm{C}\right)\mathrm{and}\:\mathrm{the}\:\mathrm{line}\:\mathrm{y}=\mathrm{2x}−\mathrm{1}\left(\mathrm{L}\right) \\ $$$$\mathrm{must}\:\mathrm{be}\:\mathrm{a}\:\mathrm{real}\:\mathrm{root}\:\mathrm{of}\:\mathrm{the}\:\mathrm{equation}: \\ $$$$\mathrm{x}^{\mathrm{4}\:} +\mathrm{3x}^{\mathrm{2}\:\:} +\mathrm{2x}=\mathrm{2x}−\mathrm{1}\Leftrightarrow\mathrm{x}^{\mathrm{4}} +\mathrm{3x}^{\mathrm{2}} +\mathrm{1}=\mathrm{0} \\ $$$$\mathrm{But}\:\mathrm{this}\:\mathrm{equation}\:\mathrm{has}\:\mathrm{no}\:\mathrm{real}\:\mathrm{roots} \\ $$$$\mathrm{simce}\:\mathrm{x}^{\mathrm{4}} +\mathrm{3x}^{\mathrm{2}} +\mathrm{1}\geqslant\mathrm{1}\forall\mathrm{x}\in\mathbb{R}.\mathrm{Hence}\:\mathrm{the} \\ $$$$\mathrm{curve}\:\left(\mathrm{C}\right)\mathrm{don}'\mathrm{t}\:\mathrm{meet}\:\mathrm{the}\:\mathrm{line}\left(\mathrm{L}\right)\left(\mathrm{q}.\mathrm{e}.\mathrm{d}\right) \\ $$$$\left.\mathrm{ii}\right)\mathrm{Denote}\:\mathrm{by}\:\mathrm{A}\left(\mathrm{x}_{\mathrm{0}} ,\mathrm{y}_{\mathrm{0}} \right)\mathrm{be}\:\mathrm{a}\:\mathrm{point}\:\mathrm{at} \\ $$$$\mathrm{which}\:\mathrm{the}\:\mathrm{tangent}\:\mathrm{of}\:\mathrm{the}\:\mathrm{curve}\:\mathrm{parallel} \\ $$$$\mathrm{to}\:\mathrm{the}\:\mathrm{line}\:\mathrm{y}=\mathrm{2x}+\mathrm{1}\:.\mathrm{Then}\: \\ $$$$\left(\mathrm{x}^{\mathrm{4}\:} +\mathrm{3x}^{\mathrm{2}\:\:} +\mathrm{2x}\right)^{'} \mid_{\mathrm{x}_{\mathrm{0}} } =\mathrm{2}\Leftrightarrow\mathrm{4x}_{\mathrm{0}} ^{\mathrm{3}} +\mathrm{6x}_{\mathrm{0}} +\mathrm{2}=\mathrm{2} \\ $$$$\Leftrightarrow\mathrm{2x}_{\mathrm{0}} \left(\mathrm{2x}_{\mathrm{0}} ^{\mathrm{2}} +\mathrm{3}\right)=\mathrm{0}\Leftrightarrow\mathrm{x}_{\mathrm{0}} =\mathrm{0}\Rightarrow\mathrm{y}_{\mathrm{0}} =\mathrm{0}.\mathrm{Then}\: \\ $$$$\mathrm{the}\:\mathrm{nearest}\:\mathrm{distance}\:\mathrm{between}\:\mathrm{the}\:\mathrm{curve} \\ $$$$\mathrm{and}\:\mathrm{the}\:\mathrm{line}\:\mathrm{be}\:\mathrm{the}\:\mathrm{distance}\:\mathrm{between} \\ $$$$\mathrm{A}\left(\mathrm{x}_{\mathrm{0}} ,\mathrm{y}_{\mathrm{0}} \right)\:\mathrm{and}\:\mathrm{the}\:\mathrm{line}\:\mathrm{y}=\mathrm{2x}−\mathrm{1which} \\ $$$$\mathrm{means}\:\mathrm{d}_{\mathrm{0}} =\frac{\mid\mathrm{2}.\mathrm{0}−\mathrm{1}.\mathrm{0}\mid}{\sqrt{\mathrm{2}^{\mathrm{2}} +\mathrm{1}^{\mathrm{2}} }}=\frac{\mathrm{1}}{\sqrt{\mathrm{5}}} \\ $$

Commented by Ari last updated on 29/Jul/20

you are right

Terms of Service

Privacy Policy

Contact: info@tinkutara.com