Question and Answers Forum

All Questions      Topic List

Trigonometry Questions

Previous in All Question      Next in All Question      

Previous in Trigonometry      Next in Trigonometry      

Question Number 105759 by bramlex last updated on 31/Jul/20

If α and β are the solution of equation a tan θ + b sec θ = c . find the value of tan (α+β).

$${If}\:\alpha\:{and}\:\beta\:{are}\:{the}\:{solution}\:{of} \\ $$$${equation}\:{a}\:\mathrm{tan}\:\theta\:+\:{b}\:\mathrm{sec}\:\theta\:=\:{c}\:.\: \\ $$$${find}\:{the}\:{value}\:{of}\:\mathrm{tan}\:\left(\alpha+\beta\right). \\ $$

Commented by bramlex last updated on 31/Jul/20

thx both

$${thx}\:{both} \\ $$

Answered by john santu last updated on 31/Jul/20

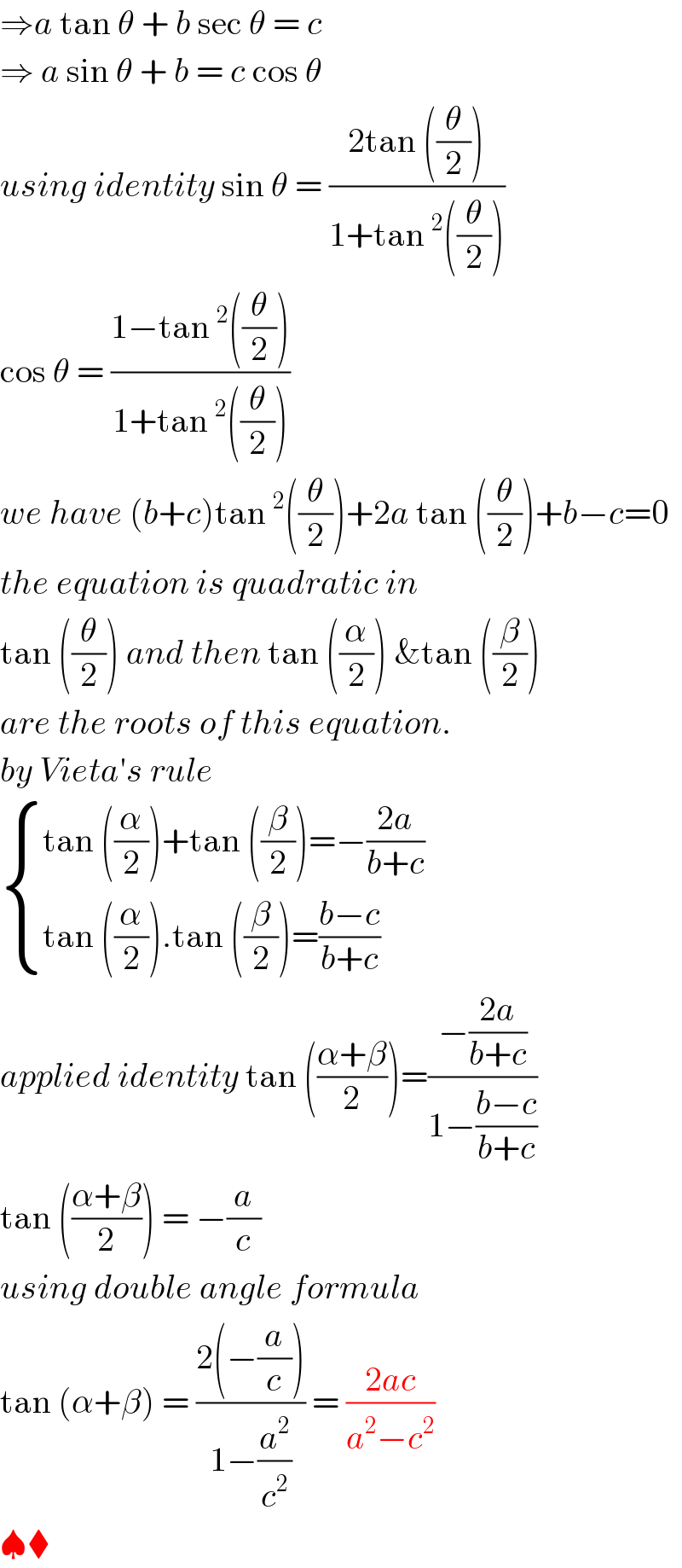
⇒a tan θ + b sec θ = c ⇒ a sin θ + b = c cos θ using identity sin θ = ((2tan ((θ/2)))/(1+tan^2 ((θ/2)))) cos θ = ((1−tan^2 ((θ/2)))/(1+tan^2 ((θ/2)))) we have (b+c)tan^2 ((θ/2))+2a tan ((θ/2))+b−c=0 the equation is quadratic in tan ((θ/2)) and then tan ((α/2)) &tan ((β/2)) are the roots of this equation. by Vieta′s rule { ((tan ((α/2))+tan ((β/2))=−((2a)/(b+c)))),((tan ((α/2)).tan ((β/2))=((b−c)/(b+c)))) :} applied identity tan (((α+β)/2))=((−((2a)/(b+c)))/(1−((b−c)/(b+c)))) tan (((α+β)/2)) = −(a/c) using double angle formula tan (α+β) = ((2(−(a/c)))/(1−(a^2 /c^2 ))) = ((2ac)/(a^2 −c^2 )) ♠⧫

$$\Rightarrow{a}\:\mathrm{tan}\:\theta\:+\:{b}\:\mathrm{sec}\:\theta\:=\:{c}\: \\ $$$$\Rightarrow\:{a}\:\mathrm{sin}\:\theta\:+\:{b}\:=\:{c}\:\mathrm{cos}\:\theta\: \\ $$$${using}\:{identity}\:\mathrm{sin}\:\theta\:=\:\frac{\mathrm{2tan}\:\left(\frac{\theta}{\mathrm{2}}\right)}{\mathrm{1}+\mathrm{tan}\:^{\mathrm{2}} \left(\frac{\theta}{\mathrm{2}}\right)} \\ $$$$\mathrm{cos}\:\theta\:=\:\frac{\mathrm{1}−\mathrm{tan}\:^{\mathrm{2}} \left(\frac{\theta}{\mathrm{2}}\right)}{\mathrm{1}+\mathrm{tan}\:^{\mathrm{2}} \left(\frac{\theta}{\mathrm{2}}\right)} \\ $$$${we}\:{have}\:\left({b}+{c}\right)\mathrm{tan}\:^{\mathrm{2}} \left(\frac{\theta}{\mathrm{2}}\right)+\mathrm{2}{a}\:\mathrm{tan}\:\left(\frac{\theta}{\mathrm{2}}\right)+{b}−{c}=\mathrm{0} \\ $$$${the}\:{equation}\:{is}\:{quadratic}\:{in}\: \\ $$$$\mathrm{tan}\:\left(\frac{\theta}{\mathrm{2}}\right)\:{and}\:{then}\:\mathrm{tan}\:\left(\frac{\alpha}{\mathrm{2}}\right)\:\&\mathrm{tan}\:\left(\frac{\beta}{\mathrm{2}}\right) \\ $$$${are}\:{the}\:{roots}\:{of}\:{this}\:{equation}. \\ $$$${by}\:{Vieta}'{s}\:{rule}\: \\ $$$$\begin{cases}{\mathrm{tan}\:\left(\frac{\alpha}{\mathrm{2}}\right)+\mathrm{tan}\:\left(\frac{\beta}{\mathrm{2}}\right)=−\frac{\mathrm{2}{a}}{{b}+{c}}}\\{\mathrm{tan}\:\left(\frac{\alpha}{\mathrm{2}}\right).\mathrm{tan}\:\left(\frac{\beta}{\mathrm{2}}\right)=\frac{{b}−{c}}{{b}+{c}}}\end{cases} \\ $$$${applied}\:{identity}\:\mathrm{tan}\:\left(\frac{\alpha+\beta}{\mathrm{2}}\right)=\frac{−\frac{\mathrm{2}{a}}{{b}+{c}}}{\mathrm{1}−\frac{{b}−{c}}{{b}+{c}}} \\ $$$$\mathrm{tan}\:\left(\frac{\alpha+\beta}{\mathrm{2}}\right)\:=\:−\frac{{a}}{{c}} \\ $$$${using}\:{double}\:{angle}\:{formula} \\ $$$$\mathrm{tan}\:\left(\alpha+\beta\right)\:=\:\frac{\mathrm{2}\left(−\frac{{a}}{{c}}\right)}{\mathrm{1}−\frac{{a}^{\mathrm{2}} }{{c}^{\mathrm{2}} }}\:=\:\frac{\mathrm{2}{ac}}{{a}^{\mathrm{2}} −{c}^{\mathrm{2}} } \\ $$$$\spadesuit\blacklozenge \\ $$

Commented by Coronavirus last updated on 01/Aug/20

clear thanks you sir

Answered by bobhans last updated on 31/Jul/20

a tan θ + b sec θ = c →a tan θ−c = −b sec θ squaring both side (a tan θ−c)^2 = b^2 (1+tan^2 θ) (a^2 −b^2 )tan^2 θ −2ac tan θ + c^2 −b^2 =0 has the roots are tan α and tan β Vieta′s rule { ((tan α+tan β=((2ac)/(a^2 −b^2 )))),((tan α.tan β = ((c^2 −b^2 )/(a^2 −b^2 )))) :} therefore tan (α+β) = ((tan α+tan β)/(1−tan α.tan β)) = ((2ac)/(a^2 −c^2 )) ★

$${a}\:\mathrm{tan}\:\theta\:+\:{b}\:\mathrm{sec}\:\theta\:=\:{c}\: \\ $$$$\rightarrow{a}\:\mathrm{tan}\:\theta−{c}\:=\:−{b}\:\mathrm{sec}\:\theta \\ $$$${squaring}\:{both}\:{side}\: \\ $$$$\left({a}\:\mathrm{tan}\:\theta−{c}\right)^{\mathrm{2}} \:=\:{b}^{\mathrm{2}} \left(\mathrm{1}+\mathrm{tan}\:^{\mathrm{2}} \theta\right) \\ $$$$\left({a}^{\mathrm{2}} −{b}^{\mathrm{2}} \right)\mathrm{tan}\:^{\mathrm{2}} \theta\:−\mathrm{2}{ac}\:\mathrm{tan}\:\theta\:+\:{c}^{\mathrm{2}} −{b}^{\mathrm{2}} =\mathrm{0}\: \\ $$$${has}\:{the}\:{roots}\:{are}\:\mathrm{tan}\:\alpha\:{and}\:\mathrm{tan}\:\beta \\ $$$${Vieta}'{s}\:{rule}\:\begin{cases}{\mathrm{tan}\:\alpha+\mathrm{tan}\:\beta=\frac{\mathrm{2}{ac}}{{a}^{\mathrm{2}} −{b}^{\mathrm{2}} }}\\{\mathrm{tan}\:\alpha.\mathrm{tan}\:\beta\:=\:\frac{{c}^{\mathrm{2}} −{b}^{\mathrm{2}} }{{a}^{\mathrm{2}} −{b}^{\mathrm{2}} }}\end{cases} \\ $$$${therefore}\:\mathrm{tan}\:\left(\alpha+\beta\right)\:=\:\frac{\mathrm{tan}\:\alpha+\mathrm{tan}\:\beta}{\mathrm{1}−\mathrm{tan}\:\alpha.\mathrm{tan}\:\beta} \\ $$$$\:=\:\frac{\mathrm{2}{ac}}{{a}^{\mathrm{2}} −{c}^{\mathrm{2}} }\:\bigstar \\ $$

Commented by Coronavirus last updated on 01/Aug/20

wouah very fast method

Terms of Service

Privacy Policy

Contact: info@tinkutara.com