
Question and Answers Forum
Question Number 106709 by bemath last updated on 06/Aug/20
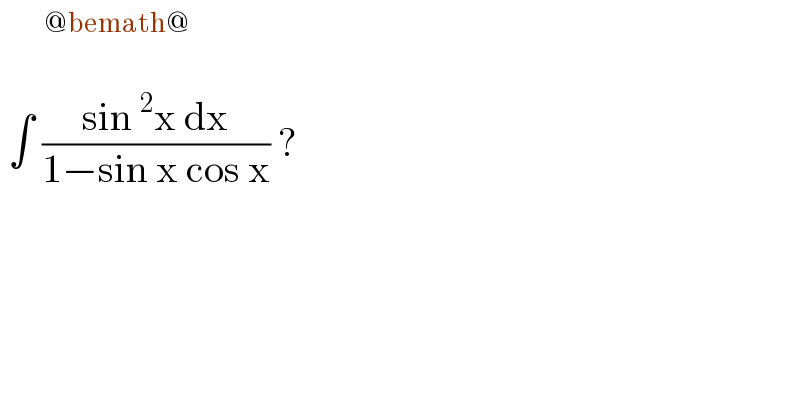
Answered by john santu last updated on 06/Aug/20
![^(@JS@) I=∫ (((1/2)−(1/2)cos 2x)/(1−((sin 2x)/2))) dx = ∫ ((1−cos 2x)/(2−sin 2x)) dx I=∫ (dx/(2−sin 2x)) −∫ ((cos 2x)/(2−sin 2x)) dx set I_1 =∫ (dx/(2−sin 2x)) [ let tan x = ♭ ] I_1 = ∫ ((((1/(♭^2 +1))) d♭)/(2−(((2♭)/(♭^2 +1))))) = ∫ (d♭/(2♭^2 −2♭+2)) I_1 =(1/2)∫ (d♭/((♭−(1/2))^2 +(((√3)/2))^2 )) I_2 =∫ ((cos 2x dx)/(2−sin 2x)) = −(1/2)∫ ((d(2−sin 2x))/(2−sin 2x)) I_1 =−(1/2)ln ∣2−sin 2x∣ ∴ I = I_1 −I_2](Q106715.png)
Answered by Dwaipayan Shikari last updated on 06/Aug/20
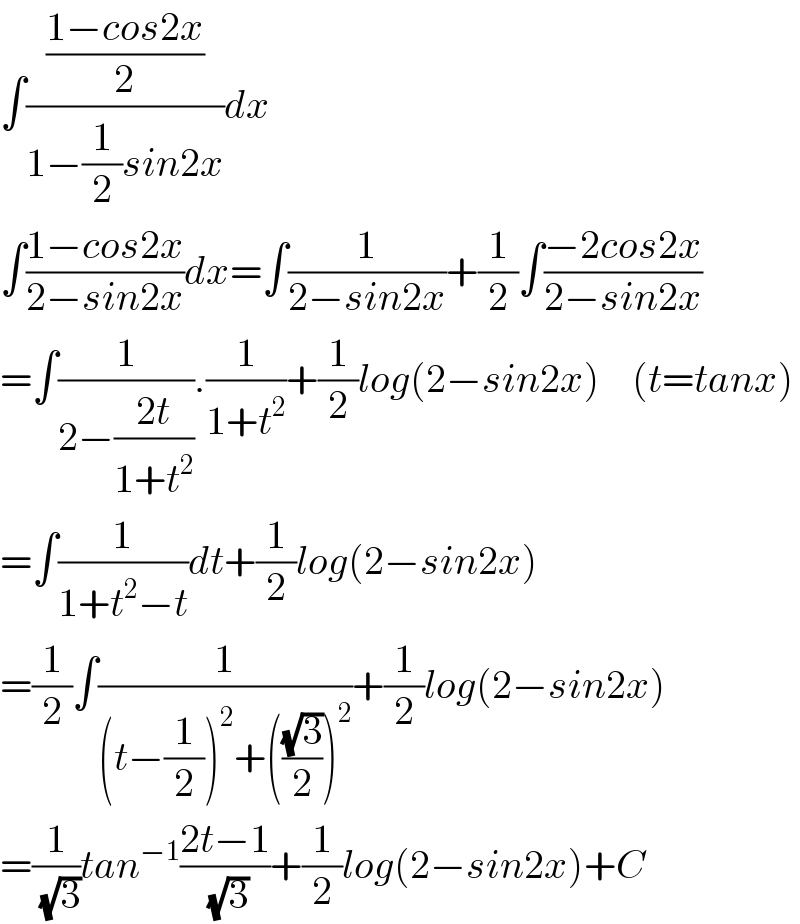
Answered by mathmax by abdo last updated on 07/Aug/20

| ||
Question and Answers Forum | ||
Question Number 106709 by bemath last updated on 06/Aug/20 | ||
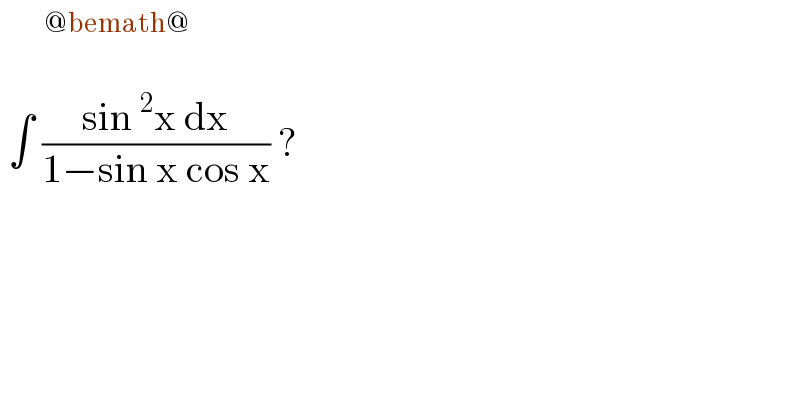 | ||
Answered by john santu last updated on 06/Aug/20 | ||
![^(@JS@) I=∫ (((1/2)−(1/2)cos 2x)/(1−((sin 2x)/2))) dx = ∫ ((1−cos 2x)/(2−sin 2x)) dx I=∫ (dx/(2−sin 2x)) −∫ ((cos 2x)/(2−sin 2x)) dx set I_1 =∫ (dx/(2−sin 2x)) [ let tan x = ♭ ] I_1 = ∫ ((((1/(♭^2 +1))) d♭)/(2−(((2♭)/(♭^2 +1))))) = ∫ (d♭/(2♭^2 −2♭+2)) I_1 =(1/2)∫ (d♭/((♭−(1/2))^2 +(((√3)/2))^2 )) I_2 =∫ ((cos 2x dx)/(2−sin 2x)) = −(1/2)∫ ((d(2−sin 2x))/(2−sin 2x)) I_1 =−(1/2)ln ∣2−sin 2x∣ ∴ I = I_1 −I_2](Q106715.png) | ||
| ||
Answered by Dwaipayan Shikari last updated on 06/Aug/20 | ||
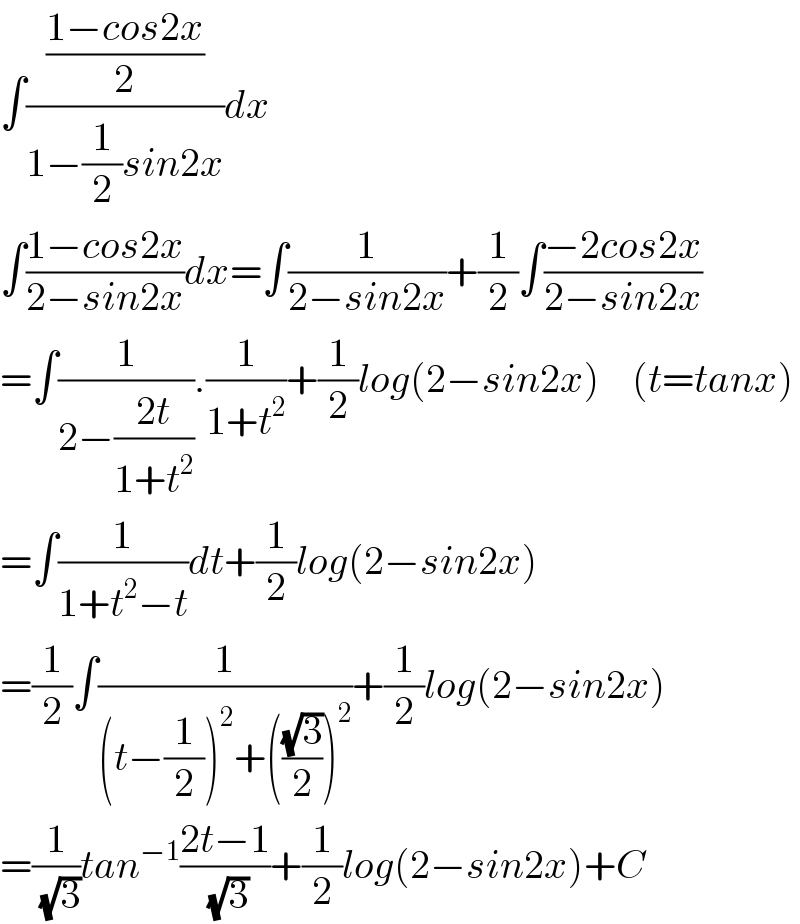 | ||
| ||
Answered by mathmax by abdo last updated on 07/Aug/20 | ||
 | ||
| ||