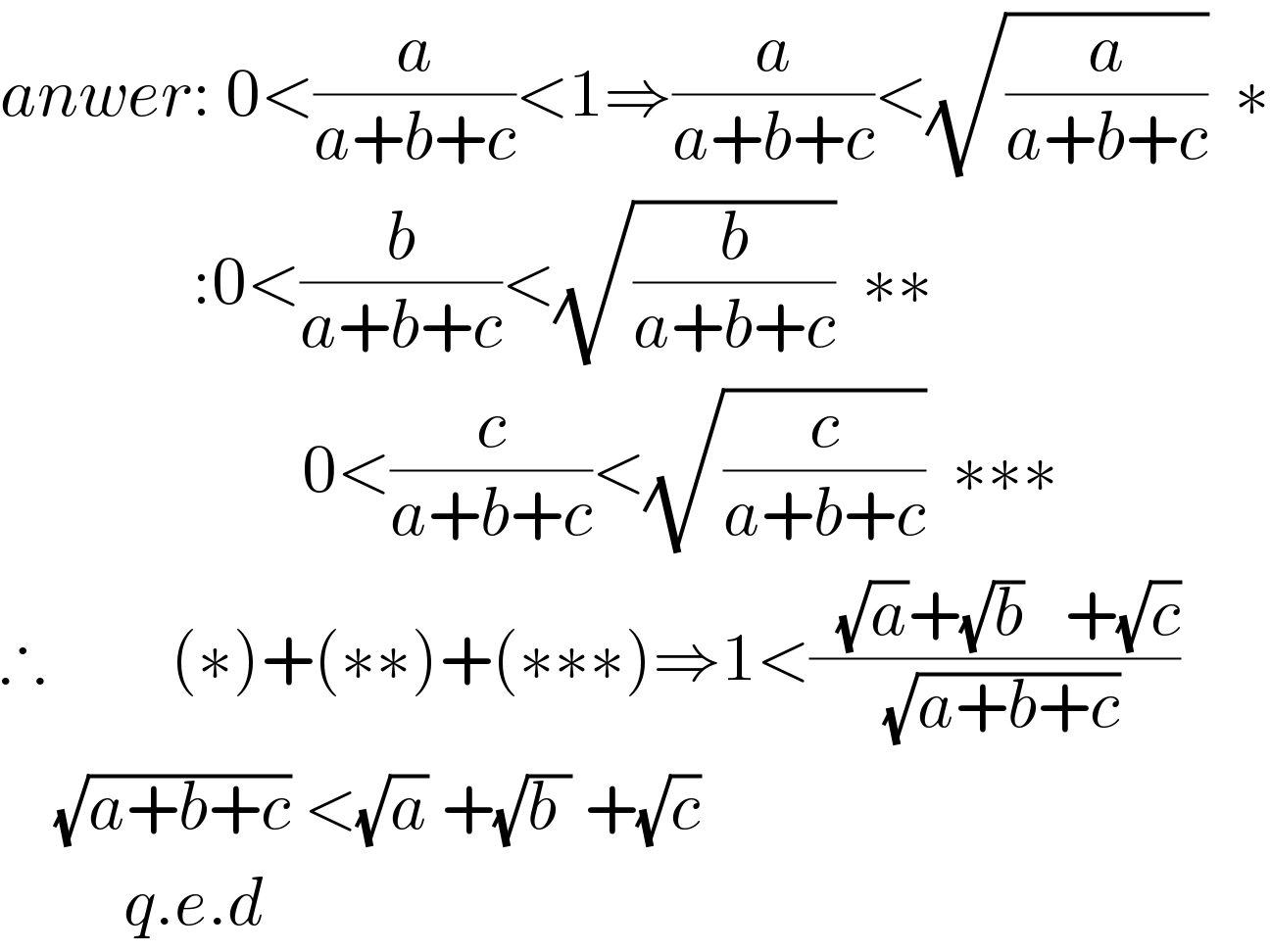Question and Answers Forum

All Questions      Topic List

Algebra Questions

Previous in All Question      Next in All Question      

Previous in Algebra      Next in Algebra      

Question Number 107242 by mnjuly1970 last updated on 09/Aug/20

$$...question...$$ $$provethat:$$ $$ifa,b,c\in {\mathbb{R}}^{+}then:$$ $$\mathrm{♣}\sqrt{a}+\sqrt{b}+\sqrt{c}>\sqrt{a+b+c}\mathrm{♣}$$ $$....sincerlyyours...$$ $$...\mathcal{M}.\mathcal{N}...$$ $$$$

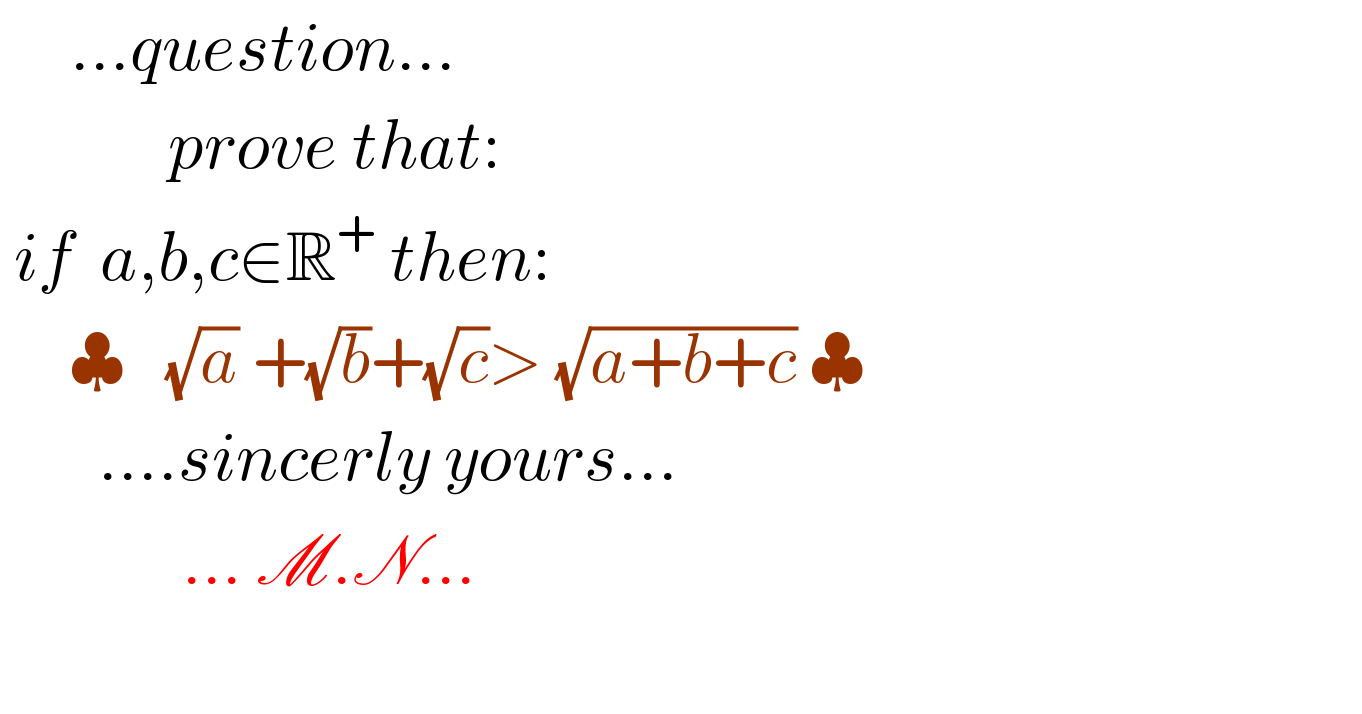
Answered by abdomathmax last updated on 09/Aug/20

$$\mathrm{a}={\mathrm{x}}^2,\mathrm{b}={\mathrm{y}}^2,\mathrm{c}={\mathrm{z}}^2\left(\mathrm{i}\right)\Rightarrow \mathrm{x}+\mathrm{y}+\mathrm{z}>\sqrt{{\mathrm{x}}^2+{\mathrm{y}}^2+{\mathrm{z}}^2}$$ $$\Rightarrow {(\mathrm{x}+\mathrm{y}+\mathrm{z})}^2>{\mathrm{x}}^2+{\mathrm{y}}^2+{\mathrm{z}}^2\Rightarrow$$ $${\mathrm{x}}^2+{\mathrm{y}}^2+{\mathrm{z}}^2+2(\mathrm{xy}+\mathrm{yz}+\mathrm{zx})>{\mathrm{x}}^2+{\mathrm{y}}^2+{\mathrm{z}}^2$$ $$\mathrm{xy}+\mathrm{yz}+\mathrm{zx}>0\mathrm{condition}5\mathrm{erified}\mathrm{if}\mathrm{a}>0,\mathrm{b}>0$$ $$\mathrm{and}\mathrm{c}>0$$

Commented bymnjuly1970 last updated on 09/Aug/20

$$thankyou$$

Commented bymathmax by abdo last updated on 09/Aug/20

$$\mathrm{you}\mathrm{are}\mathrm{welcome}$$

Answered by 1549442205PVT last updated on 09/Aug/20

$$\mathrm{The}\mathrm{inequality}\sqrt{\mathrm{a}}+\sqrt{\mathrm{b}}+\sqrt{\mathrm{c}}>\sqrt{\mathrm{a}+\mathrm{b}+\mathrm{c}}$$ $$\mathrm{is}\mathrm{always}\mathrm{true}\mathrm{\forall }\mathrm{a},\mathrm{b},\mathrm{c}\in {\mathbb{R}}^{+}\mathrm{since}$$ $$\mathrm{squaring}\mathrm{two}\mathrm{sides}\mathrm{we}\mathrm{get}\mathrm{an}\mathrm{equivalent}\mathrm{inequality}$$ $$\mathrm{a}+\mathrm{b}+\mathrm{c}+2\sqrt{\mathrm{ab}}+2\sqrt{\mathrm{bc}}+2\sqrt{\mathrm{ca}}>\mathrm{a}+\mathrm{b}+\mathrm{c}$$ $$\mathrm{which}\mathrm{is}\mathrm{always}\mathrm{true}$$

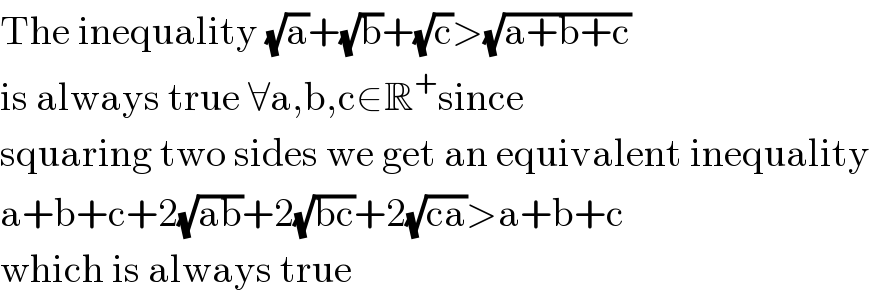
Commented bymnjuly1970 last updated on 09/Aug/20

$$\ast thankyousomuch\ast$$ $$thanks$$

Answered by udaythool last updated on 09/Aug/20

$$\mathrm{By}\mathrm{contradiction}\mathrm{method}:$$ $$\mathrm{If}\mathrm{for}\mathrm{some}a,b,c\in {\mathbb{R}}^{+}$$ $$\sqrt{a}+\sqrt{b}+\sqrt{c}⩽\sqrt{a+b+c}$$ $$\Rightarrow 0<\sqrt{ab}+\sqrt{ac}+\sqrt{bc}⩽0$$ $$\mathrm{which}\mathrm{is}\mathrm{absurd}.$$

Commented bymnjuly1970 last updated on 09/Aug/20

$$niceverynice$$

Answered by mnjuly1970 last updated on 09/Aug/20

$$anwer:0<\frac{a}{a+b+c}<1\Rightarrow \frac{a}{a+b+c}<\sqrt{\frac{a}{a+b+c}}\ast$$ $$:0<\frac{b}{a+b+c}<\sqrt{\frac{b}{a+b+c}}\ast \ast$$ $$0<\frac{c}{a+b+c}<\sqrt{\frac{c}{a+b+c}}\ast \ast \ast$$ $$\therefore (\ast )+(\ast \ast )+(\ast \ast \ast )\Rightarrow 1<\frac{\sqrt{a}+\sqrt{b}+\sqrt{c}}{\sqrt{a+b+c}}$$ $$\sqrt{a+b+c}<\sqrt{a}+\sqrt{b}+\sqrt{c}$$ $$q.e.d$$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com