
Question Number 107310 by bemath last updated on 10/Aug/20

Answered by bobhans last updated on 10/Aug/20
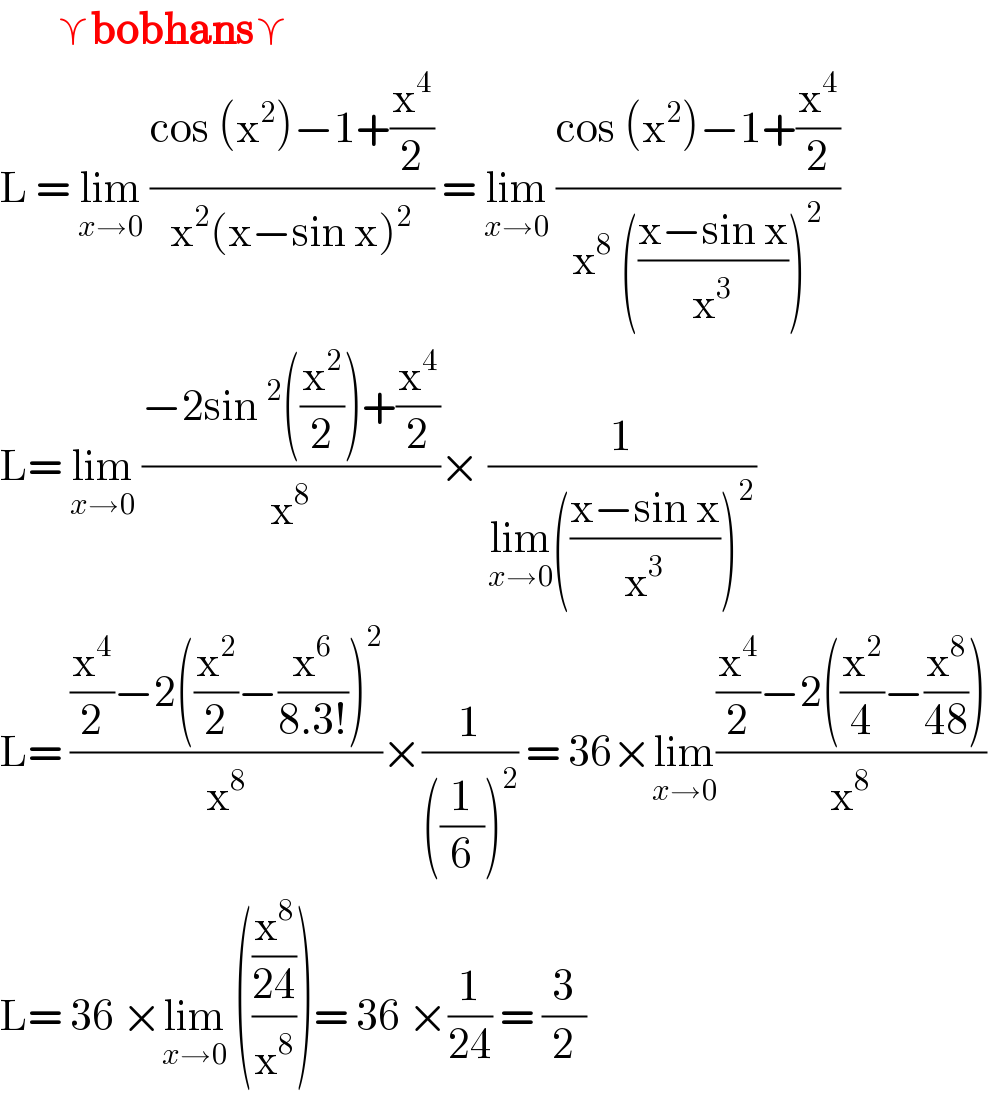
$$\:\:\:\:\:\:\:\curlyvee\boldsymbol{\mathrm{bobhans}}\curlyvee \\ $$$$\mathrm{L}\:=\:\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\:\frac{\mathrm{cos}\:\left(\mathrm{x}^{\mathrm{2}} \right)−\mathrm{1}+\frac{\mathrm{x}^{\mathrm{4}} }{\mathrm{2}}}{\mathrm{x}^{\mathrm{2}} \left(\mathrm{x}−\mathrm{sin}\:\mathrm{x}\right)^{\mathrm{2}} }\:=\:\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\:\frac{\mathrm{cos}\:\left(\mathrm{x}^{\mathrm{2}} \right)−\mathrm{1}+\frac{\mathrm{x}^{\mathrm{4}} }{\mathrm{2}}}{\mathrm{x}^{\mathrm{8}} \:\left(\frac{\mathrm{x}−\mathrm{sin}\:\mathrm{x}}{\mathrm{x}^{\mathrm{3}} }\right)^{\mathrm{2}} } \\ $$$$\mathrm{L}=\:\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\:\frac{−\mathrm{2sin}\:^{\mathrm{2}} \left(\frac{\mathrm{x}^{\mathrm{2}} }{\mathrm{2}}\right)+\frac{\mathrm{x}^{\mathrm{4}} }{\mathrm{2}}}{\mathrm{x}^{\mathrm{8}} }×\:\frac{\mathrm{1}}{\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\left(\frac{\mathrm{x}−\mathrm{sin}\:\mathrm{x}}{\mathrm{x}^{\mathrm{3}} }\right)^{\mathrm{2}} } \\ $$$$\mathrm{L}=\:\frac{\frac{\mathrm{x}^{\mathrm{4}} }{\mathrm{2}}−\mathrm{2}\left(\frac{\mathrm{x}^{\mathrm{2}} }{\mathrm{2}}−\frac{\mathrm{x}^{\mathrm{6}} }{\mathrm{8}.\mathrm{3}!}\right)^{\mathrm{2}} }{\mathrm{x}^{\mathrm{8}} }×\frac{\mathrm{1}}{\left(\frac{\mathrm{1}}{\mathrm{6}}\right)^{\mathrm{2}} }\:=\:\mathrm{36}×\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\frac{\frac{\mathrm{x}^{\mathrm{4}} }{\mathrm{2}}−\mathrm{2}\left(\frac{\mathrm{x}^{\mathrm{2}} }{\mathrm{4}}−\frac{\mathrm{x}^{\mathrm{8}} }{\mathrm{48}}\right)}{\mathrm{x}^{\mathrm{8}} } \\ $$$$\mathrm{L}=\:\mathrm{36}\:×\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\:\left(\frac{\frac{\mathrm{x}^{\mathrm{8}} }{\mathrm{24}}}{\mathrm{x}^{\mathrm{8}} }\right)=\:\mathrm{36}\:×\frac{\mathrm{1}}{\mathrm{24}}\:=\:\frac{\mathrm{3}}{\mathrm{2}} \\ $$
Answered by 1549442205PVT last updated on 10/Aug/20
![Lim_(x→0) ((cos(x^2 )−1+(x^4 /2))/(x^2 (x−sinx)^2 )) = _(maclaurent) lim_(x→0) ((cos(x^2 )−1+(x^4 /4))/(x^2 [x−(x−(x^3 /6))]^2 )) =lim_(x→0) ((cos(x^2 )−1+(x^4 /4))/(x^8 /(36))) = _(L′Hopital) lim_(x→0) ((−sin(x^2 ).2x+x^3 )/((8x^7 )/(36))) =lim_(x→0) ((−2sin(x^2 )+x^2 )/((8x^6 )/(36)))=lim_(x→0) ((−2cos(x^2 ).2x+2x)/((48x^5 )/(36))) =lim_(x→0) ((−2cos(x^2 )+1)/((24x^4 )/(36)))=lim_(x→0) ((−72cos(x^2 )+1)/(24x^4 )) =+∞](Q107335.png)
$$ \\ $$$$\underset{\mathrm{x}\rightarrow\mathrm{0}} {\mathrm{Lim}}\:\frac{\mathrm{cos}\left(\mathrm{x}^{\mathrm{2}} \right)−\mathrm{1}+\frac{\mathrm{x}^{\mathrm{4}} }{\mathrm{2}}}{\mathrm{x}^{\mathrm{2}} \left(\mathrm{x}−\mathrm{sinx}\right)^{\mathrm{2}} }\underset{\mathrm{maclaurent}} {\:\:\:=\:\:\:\:}\underset{\mathrm{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\frac{\mathrm{cos}\left(\mathrm{x}^{\mathrm{2}} \right)−\mathrm{1}+\frac{\mathrm{x}^{\mathrm{4}} }{\mathrm{4}}}{\mathrm{x}^{\mathrm{2}} \left[\mathrm{x}−\left(\mathrm{x}−\frac{\mathrm{x}^{\mathrm{3}} }{\mathrm{6}}\right)\right]^{\mathrm{2}} } \\ $$$$=\underset{\mathrm{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\frac{\mathrm{cos}\left(\mathrm{x}^{\mathrm{2}} \right)−\mathrm{1}+\frac{\mathrm{x}^{\mathrm{4}} }{\mathrm{4}}}{\frac{\mathrm{x}^{\mathrm{8}} }{\mathrm{36}}}\underset{\mathrm{L}'\mathrm{Hopital}} {\:\:\:\:=\:\:\:\:}\underset{\mathrm{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\frac{−\mathrm{sin}\left(\mathrm{x}^{\mathrm{2}} \right).\mathrm{2x}+\mathrm{x}^{\mathrm{3}} }{\frac{\mathrm{8x}^{\mathrm{7}} }{\mathrm{36}}} \\ $$$$=\underset{\mathrm{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\frac{−\mathrm{2sin}\left(\mathrm{x}^{\mathrm{2}} \right)+\mathrm{x}^{\mathrm{2}} }{\frac{\mathrm{8x}^{\mathrm{6}} }{\mathrm{36}}}=\underset{\mathrm{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\frac{−\mathrm{2cos}\left(\mathrm{x}^{\mathrm{2}} \right).\mathrm{2x}+\mathrm{2x}}{\frac{\mathrm{48x}^{\mathrm{5}} }{\mathrm{36}}} \\ $$$$=\underset{\mathrm{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\frac{−\mathrm{2cos}\left(\mathrm{x}^{\mathrm{2}} \right)+\mathrm{1}}{\frac{\mathrm{24x}^{\mathrm{4}} }{\mathrm{36}}}=\underset{\mathrm{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\frac{−\mathrm{72cos}\left(\mathrm{x}^{\mathrm{2}} \right)+\mathrm{1}}{\mathrm{24x}^{\mathrm{4}} } \\ $$$$=+\infty \\ $$
Commented by bemath last updated on 10/Aug/20

$${limit}\:{exist} \\ $$
Commented by 1549442205PVT last updated on 10/Aug/20

$$\mathrm{Thank}\:\mathrm{Sir}\:.\mathrm{I}\:\mathrm{mistaked}\:\mathrm{and}\:\mathrm{corrected} \\ $$
Answered by Dwaipayan Shikari last updated on 10/Aug/20
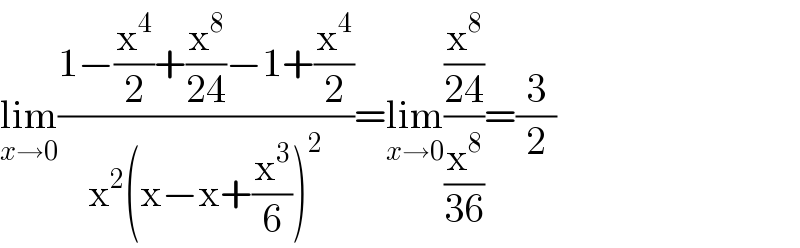
$$\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\frac{\mathrm{1}−\frac{\mathrm{x}^{\mathrm{4}} }{\mathrm{2}}+\frac{\mathrm{x}^{\mathrm{8}} }{\mathrm{24}}−\mathrm{1}+\frac{\mathrm{x}^{\mathrm{4}} }{\mathrm{2}}}{\mathrm{x}^{\mathrm{2}} \left(\mathrm{x}−\mathrm{x}+\frac{\mathrm{x}^{\mathrm{3}} }{\mathrm{6}}\right)^{\mathrm{2}} }=\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\frac{\frac{\mathrm{x}^{\mathrm{8}} }{\mathrm{24}}}{\frac{\mathrm{x}^{\mathrm{8}} }{\mathrm{36}}}=\frac{\mathrm{3}}{\mathrm{2}} \\ $$
